Solid Klein bottle

In mathematics, a solid Klein bottle is a three-dimensional topological space (a 3-manifold) whose boundary is the Klein bottle.

It is homeomorphic to the quotient space obtained by gluing the top disk of a cylinder $\scriptstyle D^{2}\times I$ to the bottom disk by a reflection across a diameter of the disk.

Mö x I: the circle of black points marks an absolute deformation retract of this space, and any regular neighbourhood of it has again boundary as a Klein bottle, so Mö x I is an onion of Klein bottles

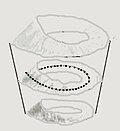
Alternatively, one can visualize the solid Klein bottle as the trivial product $\scriptstyle M{\ddot {o}}\times I$, of the möbius strip and an interval $\scriptstyle I=[0,1]$. In this model one can see that the core central curve at 1/2 has a regular neighborhood which is again a trivial cartesian product: $\scriptstyle M{\ddot {o}}\times [{\frac {1}{2}}-\varepsilon ,{\frac {1}{2}}+\varepsilon ]$ and whose boundary is a Klein bottle.