Heronian triangle

In geometry, a Heronian triangle is a triangle whose side lengths and area are all integers. Heronian triangles are named after Hero of Alexandria. The term is sometimes applied more widely to triangles whose sides and area are all rational numbers, since one can rescale the sides by a common multiple to obtain a triangle whose sides and area are integers.

Properties

Any right-angled triangle whose sidelengths are a Pythagorean triple is a Heronian triangle. The side lengths of such a triangle are integers, by definition. In any such triangle, one of the two shorter sides has even length, so the area (the product of these two sides, divided by two) is also an integer.

A triangle with sidelengths c, e and b + d, and height a.

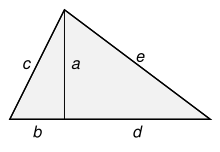
An example of a Heronian triangle which is not right-angled is the isosceles triangle with sidelengths 5, 5, and 6, whose area is 12. This triangle is obtained by joining two copies of the right-angled triangle with sides 3, 4, and 5 along the sides of length 4. This approach works in general, as illustrated in the adjacent picture. One takes a Pythagorean triple (a, b, c), with c being largest, then another one (a, d, e), with e being largest, constructs the triangles with these sidelengths, and joins them together along the sides of length a, to obtain a triangle with integer side lengths c, e, and b + d, and with area

$A={\frac {1}{2}}(b+d)a$ (one half times the base times the height).

If a is even then the area A is an integer. Less obviously, if a is odd then A is still an integer, as b and d must both be even, making b+d even too.

Some Heronian triangles cannot be obtained by joining two right-angled triangles with integer sides as described above. For example, a 5, 29, 30 Heronian triangle with area 72 cannot be constructed from two integer Pythagorean triangles since none of its altitudes are integers. Also no primitive Pythagorean triangle can be constructed from two smaller integer Pythagorean triangles. Such Heronian triangles are known as indecomposable. However, if one allows Pythagorean triples with rational values, not necessarily integers, then a decomposition into right triangles with rational sides always exists. The altitude of such a triangle must be a rational number, equal to twice the area divided by the base. So the Heronian triangle with sides 5, 29, 30 can be constructed from rational Pythagorean triangles with sides 7/5, 24/5, 5 and 143/5, 24/5, 29. The resulting decomposition into right triangles can be rescaled so that all dimensions are integers.

Other properties of Heronian triangles are as follows:

• The perimeter of a Heronian triangle is always an even number. Thus every Heronian triangle has an odd number of sides of even length, and every primitive Heronian triangle has exactly one even side.
• The semiperimeter s of a Heronian triangle with sides a, b and c can never be prime. This can be seen from the fact that s(s−a)(s−b)(s−c) has to be a perfect square and if s is a prime then one of the other terms must have s as a factor but this is impossible as these terms are all less than s.
• The area of a Heronian triangle is always divisible by 6.
• All the altitudes of a Heronian triangle are rational. This can be seen from the fact that the area of a triangle is half of one side times its altitude from that side, and a Heronian triangle has integer sides and area. Some Heronian triangles have three non-integer altitudes, for example the acute (15, 34, 35) with area 252 and the obtuse (5, 29, 30) with area 72. Any Heronian triangle with one or more non-integer altitudes can be scaled up by a factor equalling the least common multiple of the altitudes' denominators in order to obtain a similar Heronian triangle with three integer altitudes.
• Heronian triangles that have no integer altitude (indecomposable and non-Pythagorean) have sides that are all divisible by primes of the form 4k+1. However decomposable Heronian triangles must have two sides that are the hypotenuse of Pythagorean triangles. Hence all Heronian triangles that are not Pythagorean have at least two sides that are divisible by primes of the form 4k+1. All that remains are Pythagorean triangles. Therefore, all Heronian triangles have at least one side that is divisible by primes of the form 4k+1. Finally if a Heronian triangle has only one side divisible by primes of the form 4k+1 it has to be Pythagorean with the side as the hypotenuse and the hypotenuse must be divisible by 5.
• All the interior perpendicular bisectors of a Heronian triangle are rational: For any triangle these are given by $p_{a}={\tfrac {2aA}{a^{2}+b^{2}-c^{2}}},$ $p_{b}={\tfrac {2bA}{a^{2}+b^{2}-c^{2}}},$ and $p_{c}={\tfrac {2cA}{a^{2}-b^{2}+c^{2}}},$ where the sides are a ≥ b ≥ c and the area is A; in a Heronian triangle all of a, b, c, and A are integers.
• There are no equilateral Heronian triangles.
• There are no Heronian triangles with a side length of either 1 or 2.
• There exist an infinite number of primitive Heronian triangles with one side length equal to a provided that a > 2.
• There are no Heronian triangles whose side lengths form a geometric progression.
• If any two sides (but not three) of a Heronian triangle have a common factor, that factor must be the sum of two squares.
• Every interior angle of a Heronian triangle has a rational sine. This follows from the area formula Area = (1/2)ab sin C, in which the area and the sides a and b are integers, and equivalently for the other interior angles.
• Every interior angle of a Heronian triangle has a rational cosine. This follows from the law of cosines , c² = a² + b² − 2ab cos C, in which the sides a, b, and c are integers, and equivalently for the other interior angles.
• Because all Heronian triangles have all interior angles' sines and cosines rational, this implies that the tangent, cotangent, secant, and cosecant of each interior angle is either rational or infinite.
• Half of each interior angle has a rational tangent because tan C/2 = sin C / (1 + cos C), and equivalently for other interior angles. Knowledge of these half-angle tangent values is sufficient to reconstruct the side lengths of a primitive Heronian triangle (see below).
• For any triangle, the angle spanned by a side as viewed from the center of the circumcircle is twice the interior angle of the triangle vertex opposite the side. Because the half-angle tangent for each interior angle of a Heronian triangle is rational, it follows that the quarter-angle tangent of each such central angle of a Heronian triangle is rational. (Also, the quarter-angle tangents are rational for the central angles of a Brahmagupta quadrilateral, but is an unsolved problem whether this is true for all Robbins pentagons.) The reverse is true for all cyclic polygons generally; if all such central angles have rational tangents for their quarter angles then the cyclic polygon can be scaled to simultaneously have integer side lengths and integer area.
• There are no Heronian triangles whose three internal angles form an arithmetic progression. This is because all plane triangles with interior angles in an arithmetic progression must have one interior angle of 60°, which does not have a rational sine.
• Any square inscribed in a Heronian triangle has rational sides: For a general triangle the inscribed square on side of length a has length ${\tfrac {2Aa}{a^{2}+2A}}$ where A is the triangle's area; in a Heronian triangle, both A and a are integers.
• Every Heronian triangle has a rational inradius (radius of its inscribed circle): For a general triangle the inradius is the ratio of the area to half the perimeter, and both of these are rational in a Heronian triangle.
• Every Heronian triangle has a rational circumradius (the radius of its circumscribed circle): For a general triangle the circumradius equals one-fourth the product of the sides divided by the area; in a Heronian triangle the sides and area are integers.
• In a Heronian triangle the distance from the centroid to each side is rational, because for all triangles this distance is the ratio of twice the area to three times the side length. This can be generalized by stating that all centers associated with Heronian triangles whose barycentric coordinates are rational ratios have a rational distance to each side. These centers include the circumcenter, orthocenter, nine-point center, symmedian point, Gergonne point and Nagel point.
• All Heronian triangles can be placed on a lattice with each vertex at a lattice point.

Exact formula for all Heronian triangles

The Indian mathematician Brahmagupta (598-668 A.D.) derived the parametric solution such that every Heronian triangle has sides proportional to:

$a=n(m^{2}+k^{2})$

$b=m(n^{2}+k^{2})$

$c=(m+n)(mn-k^{2})$

${\text{Semiperimeter}}=s=(a+b+c)/2=mn(m+n)$

${\text{Area}}=mnk(m+n)(mn-k^{2})$

${\text{Inradius}}=k(mn-k^{2})$

$s-a=n(mn-k^{2})$

$s-b=m(mn-k^{2})$

$s-c=(m+n)k^{2}$

for integers m, n and k where:

$\gcd {(m,n,k)}=1$

$mn>k^{2}\geq 1$

$m\geq n\geq 1$.

The proportionality factor is generally a rational  p⁄q  where  q = gcd(a, b, c)  reduces the generated Heronian triangle to its primitive and  p  scales up this primitive to the required size. For example, taking m = 36, n = 4 and k = 3 produces a triangle with a = 5220, b = 900 and c = 5400, which is similar to the 5, 29, 30 Heronian triangle and the proportionality factor used has p = 1 and q = 180.

The obstacle for a computational use of Brahmagupta's parametric solution is the denominator q of the proportionality factor. q can only be determined by calculating the greatest common divisor of the three sides ( gcd(a, b, c) ) and introduces an element of unpredictability into the generation process. The easiest way of generating lists of Heronian triangles is to generate all integer triangles up to a maximum side length and test for an integral area.

A variant

The following method of generating all Heronian triangles was noted by Leonard Euler, who was the first to provably parametrize all such triangles via

$a=mn(p^{2}+q^{2})$

$b=pq(m^{2}+n^{2})$

$c=(mq+np)(mp-nq)$

${\text{Semiperimeter}}=s=(a+b+c)/2=mp(mq+np)$

$s-a=mq(mp-nq)$

$s-b=np(mp-nq)$

$s-c=nq(mq+np)$

${\text{Area}}=mnpq(mq+np)(mp-nq)$

for integers m, n, p and q such that

$\gcd {(m,n)}=1$

$\gcd {(p,q)}=1$

$m>n$

$p>q$

Even if one chooses m, n, p, q without common divisors, it is still possible that the resulting integers a, b, c still contain a common divisor other than 2. If one chooses e.g. m = 2, n = 1, p = 7, q = 4, then one obtains: a = 2 · 65, b = 2 · 70, c = 2 · 75. If one divides by 2 as well as by 5 one obtains: a = 13, b = 14, c = 15, the same triple that one obtains if one sets m = 2, n = 1, p = 3, q = 2, except that b and c appear interchanged.

Faster algorithms have been derived by Kurz (2008).

There are infinitely many primitive and indecomposable non-Pythagorean Heronian triangles with integer values for the inradius and all three of the exradii, including the ones generated by

$a=25n^{2}+5n-5=5(5n^{2}+n-1),$

$b=25n^{3}-5n^{2}-7n+3=(5n+3)(5n^{2}-4n+1),$

$c=25n^{3}+20n^{2}-2n-4=(5n-2)(5n^{2}+6n+2),$

$A=(5n-2)(5n+3)(5n^{2}+n-1),$

$r=5n-2,$

$r_{a}=5n+3,$

$r_{b}=5n^{2}+n-1,$

$r_{c}=A=(5n-2)(5n+3)(5n^{2}+n-1).$

There are infinitely many Heronian triangles that can be placed on a lattice such that not only are the vertices at lattice points, as holds for all Heronian triangles, but additionally the centers of the incircle and excircles are at lattice points.

See also formulas for Heronian triangles with one angle equal to twice another, Heronian triangles with sides in arithmetic progression, and isosceles Heronian triangles.

All Heronian triangles from half-angle tangents

A triangle with side lengths and interior angles labeled. Capital A, B and C are the interior angles, and lower-case a, b, and c are the sides opposite them.

The tangent of half of any interior angle of a Heronian triangle is necessarily a positive rational number; see properties above. The reverse is also true; any triangle with rational half-angle tangents for all its interior angles can be scaled to be a Heronian triangle. In particular, any primitive Heronian triangle can be reconstructed from any two of its half-angle tangents values as follows.

These half angles are positive, and they sum to 90° (π/2 radians) because the interior angles (A, B, C) of any triangle sum to 180° (π radians). We start by choosing r = tan(A/2) and s = tan(B/2) to be any positive rational numbers satisfying rs < 1. The limit of 1 ensures that angle A/2 + B/2 is less than 90° and thus that the angle C/2 will be positive. The value t = tan(C/2) will also be a positive rational number because

$t=\tan(C/2)={\frac {1}{\tan(90^{\circ }-C/2)}}={\frac {1}{\tan(A/2+B/2)}}={\frac {1-\tan(A/2)\tan(B/2)}{\tan(A/2)+\tan(B/2)}}={\frac {1-rs}{r+s}}\,.$

We can compute the sine of any angle using the formula $\sin \theta ={\frac {2\tan(\theta /2)}{1+\tan ^{2}(\theta /2)}}$, so the sines of $A,B,C$ are ${\frac {2r}{1+r^{2}}},{\frac {2s}{1+s^{2}}},{\frac {2t}{1+t^{2}}}\,,$ respectively. These values are rational numbers because the values of r, s, and t are rational numbers.

We use the Law of sines to conclude that a triangle with interior angles A, B, and C can be scaled to have side lengths a, b, and c equal to these sines. The area of such a triangle is (ab sin C) / 2, which is one half the product of the three sines and will necessarily be a rational number. Integer values for the side lengths and area are obtained by multiplying the side-length values by the least common multiple of their denominators and then by dividing out by the greatest common factor of the results. Thus, we have computed the side lengths and area of a primitive Heronian triangle from two of its half-angle tangents.

When it is also the case that r, s, or t equals 1 then the corresponding interior angle will be a right angle and the three sides will also define a primitive Pythagorean triple. Starting with arbitrary positive rational numbers r = s < 1 will give all primitive isosceles Heronian triangles.

Examples

The list of primitive integer Heronian triangles, sorted by area and, if this is the same, by perimeter, starts as in the following table. "Primitive" means that the greatest common divisor of the three side lengths equals 1.

Area	Perimeter	side length b+d	side length e	side length c
6	12	5	4	3
12	16	6	5	5
12	18	8	5	5
24	32	15	13	4
30	30	13	12	5
36	36	17	10	9
36	54	26	25	3
42	42	20	15	7
60	36	13	13	10
60	40	17	15	8
60	50	24	13	13
60	60	29	25	6
66	44	20	13	11
72	64	30	29	5
84	42	15	14	13
84	48	21	17	10
84	56	25	24	7
84	72	35	29	8
90	54	25	17	12
90	108	53	51	4
114	76	37	20	19
120	50	17	17	16
120	64	30	17	17
120	80	39	25	16
126	54	21	20	13
126	84	41	28	15
126	108	52	51	5
132	66	30	25	11
156	78	37	26	15
156	104	51	40	13
168	64	25	25	14
168	84	39	35	10
168	98	48	25	25
180	80	37	30	13
180	90	41	40	9
198	132	65	55	12
204	68	26	25	17
210	70	29	21	20
210	70	28	25	17
210	84	39	28	17
210	84	37	35	12
210	140	68	65	7
210	300	149	148	3
216	162	80	73	9
234	108	52	41	15
240	90	40	37	13
252	84	35	34	15
252	98	45	40	13
252	144	70	65	9
264	96	44	37	15
264	132	65	34	33
270	108	52	29	27
288	162	80	65	17
300	150	74	51	25
300	250	123	122	5
306	108	51	37	20
330	100	44	39	17
330	110	52	33	25
330	132	61	60	11
330	220	109	100	11
336	98	41	40	17
336	112	53	35	24
336	128	61	52	15
336	392	195	193	4
360	90	36	29	25
360	100	41	41	18
360	162	80	41	41
390	156	75	68	13
396	176	87	55	34
396	198	97	90	11
396	242	120	109	13

Lists of primitive Heronian triangles whose sides do not exceed 6,000,000 can be found at "Lists of primitive Heronian triangles". Sascha Kurz, University of Bayreuth, Germany. Archived (PDF) from the original on May 2016. Retrieved 29 March 2016. {{cite web}}: Check date values in: |archive-date= (help)

Heronian triangles with perfect square sides

Heronian triangles with perfect square sides are related to the Perfect cuboid problem. As of February 2021, it is known about only two primitive Heronian triangles with perfect square sides:

(1853², 4380², 4427², Area=32918611718880) discovered by Pantelimon Stănică and coauthors at 2013,

(11789², 68104² , 68595², Area=284239560530875680) found by Randall L. Rathbun at April 4, 2018.

Equable triangles

A shape is called equable if its area equals its perimeter. There are exactly five equable Heronian triangles: the ones with side lengths (5,12,13), (6,8,10), (6,25,29), (7,15,20), and (9,10,17), though only four of them are primitive.

Almost-equilateral Heronian triangles

Since the area of an equilateral triangle with rational sides is an irrational number, no equilateral triangle is Heronian. However, a sequence of isosceles Heronian triangles that are "almost equilateral" can be developed from the duplication of right-angled triangles, in which the hypotenuse is almost twice as long as one of the legs. The first few examples of these almost-equilateral triangles are listed in the following table (sequence A102341 in the OEIS):

Side length			Area
a	b=a	c
5	5	6	12
17	17	16	120
65	65	66	1848
241	241	240	25080
901	901	902	351780
3361	3361	3360	4890480
12545	12545	12546	68149872
46817	46817	46816	949077360

There is a unique sequence of Heronian triangles that are "almost equilateral" because the three sides are of the form n − 1, n, n + 1. A method for generating all solutions to this problem based on continued fractions was described in 1864 by Edward Sang, and in 1880 Reinhold Hoppe gave a closed-form expression for the solutions. The first few examples of these almost-equilateral triangles are listed in the following table (sequence A003500 in the OEIS):

Side length			Area	Inradius
n − 1	n	n + 1
3	4	5	6	1
13	14	15	84	4
51	52	53	1170	15
193	194	195	16296	56
723	724	725	226974	209
2701	2702	2703	3161340	780
10083	10084	10085	44031786	2911
37633	37634	37635	613283664	10864

Subsequent values of n can be found by multiplying the previous value by 4, then subtracting the value prior to that one (52 = 4 × 14 − 4, 194 = 4 × 52 − 14, etc.), thus:

$n_{t}=4n_{t-1}-n_{t-2}\,,$

where t denotes any row in the table. This is a Lucas sequence. Alternatively, the formula $(2+{\sqrt {3}})^{t}+(2-{\sqrt {3}})^{t}$ generates all n for positive integers t. Equivalently, let A = area and y = inradius, then,

${\big (}(n-1)^{2}+n^{2}+(n+1)^{2}{\big )}^{2}-2{\big (}(n-1)^{4}+n^{4}+(n+1)^{4}{\big )}=(6ny)^{2}=(4A)^{2}$

where {n, y} are solutions to n² − 12y² = 4. A small transformation n = 2x yields a conventional Pell equation x² − 3y² = 1, the solutions of which can then be derived from the regular continued fraction expansion for √3.

The variable n is of the form $n={\sqrt {2+2k}}$, where k is 7, 97, 1351, 18817, …. The numbers in this sequence have the property that k consecutive integers have integral standard deviation.

See also

• Heronian tetrahedron
• Brahmagupta quadrilateral
• Robbins pentagon
• Integer triangle#Heronian triangles

External links

• Weisstein, Eric W. "Heronian triangle". MathWorld.
• Online Encyclopedia of Integer Sequences Heronian
• Wm. Fitch Cheney, Jr. (January 1929), "Heronian Triangles", Amer. Math. Monthly, 36 (1): 22–28, doi:10.1080/00029890.1929.11986902, JSTOR 2300173
• S. sh. Kozhegel'dinov (1994), "On fundamental Heronian triangles", Math. Notes, 55 (2): 151–6, doi:10.1007/BF02113294, S2CID 115233024