Hypercube

A projection of a cube (into a two-dimensional image)

A projection of a tesseract (into a two-dimensional image)

In geometry, a hypercube is an n-dimensional analogue of a square (n = 2) and a cube (n = 3). It is a closed, compact, convex figure whose 1-skeleton consists of groups of opposite parallel line segments aligned in each of the space's dimensions, at right angles to each other.

An n-dimensional hypercube is also called an n-cube. The term "measure polytope" is also used, notably in the work of H.S.M. Coxeter, but it has now been superseded.

The hypercube is the special case of a hyperrectangle (also called an orthotope).

A unit hypercube is a hypercube whose side has length one unit. Often, the hypercube whose corners (or vertices) are the 2ⁿ points in Rⁿ with coordinates equal to 0 or 1 is called "the" unit hypercube.

A point is a hypercube of dimension zero. If one moves this point one unit length, it will sweep out a line segment, which is a unit hypercube of dimension one. If one moves this line segment its length in a perpendicular direction from itself; it sweeps out a two-dimensional square. If one moves the square one unit length in the direction perpendicular to the plane it lies on, it will generate a three-dimensional cube. This can be generalized to any number of dimensions. For example, if one moves the cube one unit length into the fourth dimension, it generates a 4-dimensional unit hypercube (a unit tesseract).

The 1-skeleton of a hypercube is a hypercube graph.

Related families of polytopes

The hypercubes are one of the few families of regular polytopes that are represented in any number of dimensions.

The hypercube family is the first of three regular polytope families, labeled by Coxeter as γ_n, the other two being the hypercube dual family, the cross-polytopes, labeled as β_n, and the simplices, labeled as α_n. A fourth family, the infinite tessellation of hypercubes he labeled as δ_n.

Another related family of semiregular and uniform polytopes is the demihypercubes which are constructed from hypercubes with alternate vertices deleted and simplex facets added in the gaps, labeled as hγ_n.

Elements

A hypercube of dimension n has 2n "sides" (a 1-dimensional line has 2 end points; a 2-dimensional square has 4 sides or edges; a 3-dimensional cube has 6 2-dimensional faces; a 4-dimensional tesseract has 8 cells). The number of vertices (points) of a hypercube is 2ⁿ (a cube has 2³ vertices, for instance).

The number of m-dimensional hypercubes (just referred to as m-cube from here on) on the boundary of an n-cube is

E_{m,n}=2^{n-m}{n \choose m}

, where

{n \choose m}={\frac {n!}{m!\,(n-m)!}}

and n! denotes the factorial of n.

For example, the boundary of a 4-cube (n=4) contains 8 cubes (3-cubes), 24 squares (2-cubes), 32 lines (1-cubes) and 16 vertices (0-cubes).

This identity can be proved by combinatorial arguments; each of the $2^{n}$ vertices defines a vertice in a $m$ -dimensional boundrary. There are ${n \choose m}$ ways of choosing which lines ("sides") that defines the subspace that the boundrary is in. But, each side is counted $2^{m}$ times since it has that many vertices, we need to divide with this number. Hence the identity above.

These numbers can also be generated by the linear recurrence relation

E_{m,n}=2E_{m,n-1}+E_{m-1,n-1}\!

, with

E_{0,0}=1\!

, and undefined elements = 0.

For example, extending a square via its 4 vertices adds one extra line (edge) per vertex, and also adds the final second square, to form a cube, giving $E_{1,3}\!$ = 12 lines in total.

Hypercube elements $E_{m,n}\!$
					m	0	1	2	3	4	5	6	7	8	9
n	γ_n	n-cube	Projection graph	Center Graph	Names Schläfli symbol Coxeter-Dynkin	Vertices	Edges	Faces	Cells	4-faces	5-faces	6-faces	7-faces	8-faces	9-faces
0	γ₀	0-cube			Point -	1
1	γ₁	1-cube			Line segment {}	2	1
2	γ₂	2-cube			Square Tetragon {4}	4	4	1
3	γ₃	3-cube	File:3-cube column graph.gif		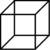Cube Hexahedron {4,3}	8	12	6	1
4	γ₄	4-cube			Tesseract Octachoron {4,3,3}	16	32	24	8	1
5	γ₅	5-cube	File:5-cube column graph.gif		Penteract Decateron {4,3,3,3}	32	80	80	40	10	1
6	γ₆	6-cube			Hexeract Dodecapeton {4,3,3,3,3}	64	192	240	160	60	12	1
7	γ₇	7-cube	File:7-cube column graph.gif		Hepteract Tetradeca-7-tope {4,3,3,3,3,3}	128	448	672	560	280	84	14	1
8	γ₈	8-cube	File:8-cube column graph.gif		Octeract Hexadeca-8-tope {4,3,3,3,3,3,3}	256	1024	1792	1792	1120	448	112	16	1
9	γ₉	9-cube	File:9-cube column graph.gif		Enneract Octadeca-9-tope {4,3,3,3,3,3,3,3}	512	2304	4608	5376	4032	2016	672	144	18	1
10	γ₁₀	10-cube	File:10-cube column graph.gif		10-cube icosa-10-tope {4,3,3,3,3,3,3,3,3}	1024	5120	11520	15360	13440	8064	3360	960	180	20

n-cube rotation

Based on observations of how 1-, 2-, and 3-dimensional hypercubes can be rotated, it is possible to hypothesize how objects with n dimensions can be rotated. A 3-dimensional hypercube can be rotated about 3 axes in 2 different ways: rotation by edge or rotation by vertex. Rotation by edge involves changing the position of every vertex but the two vertices on that particular edge. Rotation by vertex involves changing the positions of all vertices but the point of rotation. A 3-dimensional hypercube can be rotated by edge and by vertex, a 2-dimensional hypercube can only be rotated by vertex, and so on. If these series of observations are extended to higher dimensions, a 4-dimensional hypercube can be rotated about a whole face, and a 5-cube can be rotated about a whole cube.

References

Bowen, J. P., Hypercubes, Practical Computing, 5(4):97–99, April 1982.

Coxeter, H. S. M., Regular Polytopes. 3rd edition, Dover, 1973, p. 123. ISBN 0-486-61480-8. p.296, Table I (iii): Regular Polytopes, three regular polytopes in n dimensions (n ≥ 5)

Frederick J. Hill and Gerald R. Peterson, Introduction to Switching Theory and Logical Design: Second Edition, John Wiley & Sons, NY, ISBN: 0-471-39882-9. Cf Chapter 7.1 "Cubical Representation of Boolean Functions" wherein the notion of "hypercube" is introduced as a means of demonstrating a distance-1 code (Gray code) as the vertices of a hypercube, and then the hypercube with its vertices so labelled is squashed into two dimensions to form either a Veitch diagram or Karnaugh map.

External links

Weisstein, Eric W. "Hypercube". MathWorld.
Olshevsky, George. "Measure polytope". Glossary for Hyperspace. Archived from the original on 4 February 2007.
Hypercube images (2D–15D)
Animation of a Hypercube
www.4d-screen.de (Rotation of 4D – 7D-Cube)

Related families of polytopes

Elements

n-cube rotation

See also

References

External links