Complex logarithm

$~f=\ln(z)~$ with lines $~\Re (f)={\rm {const~}}$ and lines $~\Im (f)={\rm {const~}}$ in the complex $~z~$-plane.

complex logarithm drawn with a color map

In complex analysis, the complex logarithm is the extension of the natural logarithm function ln(x) – originally defined for real numbers only – into the complex plane.

The natural logarithm can be defined for all non-zero complex numbers z. It is usually denoted log(z), in line with the notational conventions of complex analysis, where the variable z is commonly used to denote a complex variable, just as x customarily stands for a real variable.

Derivation from the Taylor series

From the viewpoint of analysis, the most natural way to define log(z) is by the method of analytic continuation, from the positive real number line (where log(x) = ln(x)) into the complex plane. Here's how that works.

The Taylor series (also known as the Mercator series)

$\log(1+z)=\sum _{n=1}^{\infty }{\frac {(-1)^{n+1}}{n}}z^{n}=z-{\frac {z^{2}}{2}}+{\frac {z^{3}}{3}}-\cdots \,$

converges uniformly for all complex numbers z with absolute value less than 1. And by applying Abel's test to the Taylor series for log(1−z), it can be shown that the series for log(1+z) converges when |z| = 1, except when z = −1 (in other words, log(0) does not exist).

A careful analysis of the power series for

$\log {\frac {1+z}{1-z}}=2\left(z+{\frac {z^{3}}{3}}+{\frac {z^{5}}{5}}+\cdots \right)=2\sum _{n=0}^{\infty }{\frac {z^{2n+1}}{2n+1}}.\,$

shows that this series converges for |z| ≤ 1, except when z = ±1. By plugging

${\frac {1+i}{1-i}}=i\qquad {\frac {1-i}{1+i}}=-i$

into the power series direct calculation reveals that

$\log(i)=-\log(-i)=2i\left(1-{\frac {1}{3}}+{\frac {1}{5}}-{\frac {1}{7}}+-\cdots \right)=2i\arctan(1)=i{\frac {\pi }{2}}\,$

where the last equality follows from the well-known series for the (real) inverse tangent function.

By supposing that the laws of (real) logarithms apply to complex logarithms we obtain two results:

$i^{2}=-1\Rightarrow \log(-1)=2\log(i)=i\pi \qquad (-i)^{2}=-1\Rightarrow \log(-1)=2\log(-i)=-i\pi .\,$

The fact that log(−1) has two values can be explained this way. The complex logarithm is only continuous in a finite neighborhood of a point z, and log(0) does not exist. The "path" from z = 1 through z = i to z = −1 leads, by continuity, to one value of log(−1), while the "path" that passes through z = −i leads, by continuity, to the other value. The two paths lead to two different values because they encircle a branch point at z = 0. This multi-valued aspect of the complex logarithm is traditionally handled by making a "cut" in the complex plane all along the negative real axis. And the preceding explanation is, historically, why the argument of a complex number is often specified in the range from −π to π.

Log(z) as the inverse of the exponential function

If the non-zero complex number z is expressed in polar coordinates as z = re^iθ with r > 0 and −π < θ ≤ π, then

$\log(z)=\ln(r)+i\theta \,$

where ln(r) is the usual natural logarithm of a real number.

So defined, log (z) is holomorphic for all complex numbers which are not real numbers ≤ 0, and it has the property

$e^{\log(z)}=z\,$

for all nonzero z. Some familiar properties of the real-valued natural logarithm are no longer valid for this complex extension. For example, log(e^z) does not always equal z, and log(zw) does not always equal log(z) + log(w) – in either case, the result might have to be adjusted modulo 2πi to stay within the range of this principal branch of the complex log function.

Log(z) as a multi-valued function

A visualization of the Riemann surface of log(z). The surface appears to spiral around a vertical line corresponding to the origin of the complex plane. The actual surface extends arbitrarily far outward, but is cut off in the visualization.

A somewhat more natural definition of log(z) interprets it as a multi-valued function, or, more precisely, as a multi-valued relationship. For z = re^iθ, it would be possible to choose

$\log(z)=\ln(r)+i(\theta +2\pi k)\,$

for any integer k. There is no other complex number u for which e^u =  z, because the period of e^z is 2πi (see Euler's identity).

The standard way to deal with multi-valued relationships such as this in complex analysis is via Riemann surfaces: the function log(z) is then defined not on the complex plane, but on a suitable Riemann surface having countably many "leaves" or "sheets" instead. The function is then single-valued on each sheet, and the values of the function differ by 2πi from one sheet to the next.

More precisely, the logarithm has a branch point at z = 0 and another at the point at infinity, where the point at infinity is understood to be the point that compactifies the complex plane into the Riemann sphere. There is a branch cut between these two points; by convention, it is placed so that the cut runs off to the left, along the negative real axis, between z = 0 and z = −∞. The location of this cut is by convention only: it could be placed running from the origin in any direction, and some textbooks show the cut running downwards.

At the cut, different sheets join together, and a continuous path moving through the cut simply goes from one sheet to the next. The glueing of the sheets is such that the phase differs by 2π between two sheets at any point that is not on the cut, and by 0 at the cut itself. On the Riemann surface, then, log(−x) for positive, real x, can be defined uniquely, as long as it is made clear which sheet one is on; otherwise, by convention, log(−x) lies in the branch cut and remains undefined.

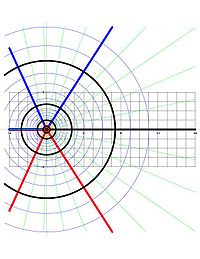
The complex logarithm as a conformal mapping

Since the derivative of log(z), z⁻¹, is a meromorphic function with one simple pole at z = 0, the principal branch of log(z) is a conformal map from ℂ/{0} into an infinitely long strip of height 2π, −π < Im(w) ≤ π. We can gain insight into the way this mapping works by writing it as

$w=\log(z)=\ln(|z|)+i\arg(z)$

and then analyzing the images in the w-plane of certain circles and straight lines in the z-plane.

• The unit circle in the z-plane is mapped into a segment of the imaginary axis in the w-plane, running from −πi to πi. Notice that the length of the resulting straight line segment equals the circumference of the original circle.

• The interior of the unit circle is mapped into the left-hand portion of the strip; that is, into the region where Re(w) < 0. More precisely, each circle centered on the origin and of radius r < 1, |z| = r, is mapped into the straight line segment Re(w) = ln(|z|). Each of these line segments is longer than the circle whose image it is, and the smaller the circle, the more it gets stretched out.

• The exterior of the unit circle is mapped into the right-hand portion of the strip; each circle |z| = r > 1 is straightened out and compressed to fit on the line segment Re(w) = ln(|z|).

• The positive real axis in the z-plane is mapped into the entire real axis in the w-plane. More precisely, if z₀ is real and z₀ > 0, its image w₀ is given by w₀ = ln(z₀). The negative real axis is mapped into the straight line Im(w) = π, with one notable difference – its direction is reversed. That is to say, the negative real axis, which runs from right to left in the z-plane, is mapped into a straight line running from left to right in the w-plane.

• All the rays originating at z = 0 are mapped into parallel horizontal lines in the w-plane. Rays that are "close" to the positive real axis lie close together in the w-plane, and rays that are '"close" to the negative real axis are farther apart, because the image of each ray arg(z) = θ is the straight line Im(w) = θ.

Since the mapping w = log(z) transforms circles into vertical straight line segments, it is useful in engineering applications involving an annulus, or doughnut-shaped region; the image of the annulus is a rectangle of height 2π, whose width depends on the thickness of the annulus. By the same token, the inverse mapping z = e^w transforms a rectangle into an annulus.

Log(z) also serves as a particularly simple illustration of the principle that conformal maps preserve angles. All the circles and radial lines discussed above are mutually perpendicular, as are the vertical and horizontal lines into which they are transformed.

Finally, the complete map of the corkscrew Riemann surface on which log(z) is holomorphic into the complex plane is easily visualized – each "sheet" of that surface is mapped into an infinitely wide strip of height 2π, and those strips cover the entire complex plane like wallpaper.

Imaginary-base logarithms

An imaginary-base logarithm is a logarithm with base a real multiple of i. These logarithms can be calculated with the formula

$\log _{i}(z)={{2\ln(z)} \over i\pi }.\,$

Where z is any complex number but 0.

Proof of the formula

Using Euler's formula, we know that

$i=e^{{i\pi }/2}.\,$

Taking the natural logarithm of each side yields

$\ln(i)={{i\pi } \over 2}.\,$

Changing the base:

$\log _{i}(z)={\ln(z) \over \ln(i)}={2\ln(z) \over i\pi }.\,$

Multiples of i for bases

If the base is a multiple of i, the formula can be generalized as follows:

$\log _{ki}(z)={{2\ln(z)} \over {i\pi +2\ln(k)}}.\,$

Where k is any real number.

This can be proved as follows:

$\log _{ki}(z)={\ln(z) \over \ln(ki)}={\ln(z) \over \ln(i)+\ln(k)}={\ln(z) \over {i\pi \over 2}+\ln(k)}={\ln(z) \over {i\pi +2\ln(k) \over 2}}={2\ln(z) \over i\pi +2\ln(k)}.\,$

Plots of the natural logarithm function on the complex plane (principal branch)

• widths="200px"
• 
  z = Re(ln(x+iy))
• 
• 
• 
  Superposition of the previous 3 graphs

See also

• Complex argument (continued fraction)
• Inverse trigonometric function
• Logarithm

References

• Gino Moretti, Functions of a Complex Variable, Prentice-Hall, Inc., 1964.
• E. T. Whittaker and G. N. Watson, A Course in Modern Analysis, fourth edition, Cambridge University Press, 1927.