File:Ballistic-trajectories-planet2.svg
Page contents not supported in other languages.
Tools
Actions
General
In other projects
Appearance
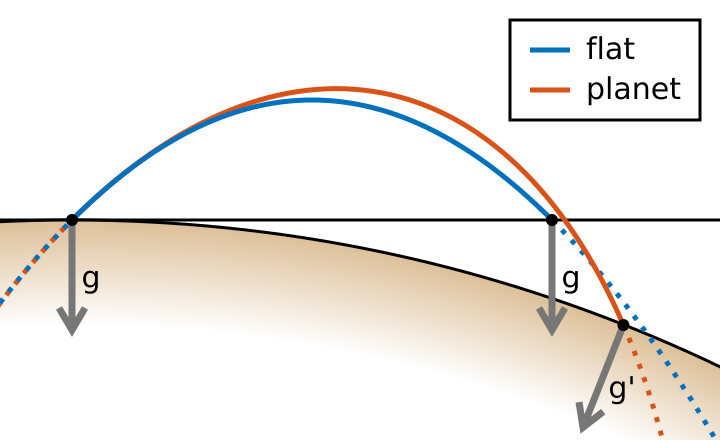
Size of this PNG preview of this SVG file: 720 × 440 pixels. Other resolutions: 320 × 196 pixels | 640 × 391 pixels | 1,024 × 626 pixels | 1,280 × 782 pixels | 2,560 × 1,564 pixels.
Original file (SVG file, nominally 720 × 440 pixels, file size: 4 KB)
| This is a file from the Wikimedia Commons. Information from its description page there is shown below. Commons is a freely licensed media file repository. You can help. |
Summary
| DescriptionBallistic-trajectories-planet2.svg |
English: Plot of two ballistic trajectories with identical initial velocity, one with a uniform gravity field (blue) and one in the central potential field of a planet (orange). The trajectories are one parabola and one ellipse, respectively. The graphical representation is accurately computed using the script, given below. |
||
| Date | |||
| Source | Own work | ||
| Author | Geek3 | ||
| Other versions | Ballistic-trajectories-planet.svg | ||
| SVG development InfoField | This vector image was created with Python. | ||
| Source code InfoField | Python code
|
Licensing
I, the copyright holder of this work, hereby publish it under the following license:
This file is licensed under the Creative Commons Attribution-Share Alike 4.0 International license.
- You are free:
- to share – to copy, distribute and transmit the work
- to remix – to adapt the work
- Under the following conditions:
- attribution – You must give appropriate credit, provide a link to the license, and indicate if changes were made. You may do so in any reasonable manner, but not in any way that suggests the licensor endorses you or your use.
- share alike – If you remix, transform, or build upon the material, you must distribute your contributions under the same or compatible license as the original.
Captions
Add a one-line explanation of what this file represents
Items portrayed in this file
depicts
image/svg+xml
3e29a2a78e43f1ad0e0653baf1aa9420e8390190
3,699 byte
440 pixel
720 pixel
File history
Click on a date/time to view the file as it appeared at that time.
| Date/Time | Thumbnail | Dimensions | User | Comment | |
|---|---|---|---|---|---|
| current | 13:03, 13 October 2020 |  | 720 × 440 (4 KB) | Geek3 | Uploaded own work with UploadWizard |
File usage
The following page uses this file:
Metadata
This file contains additional information, probably added from the digital camera or scanner used to create or digitize it.
If the file has been modified from its original state, some details may not fully reflect the modified file.
| Short title | Ballistic-trajectories-planet2 |
|---|---|
| Image title | Ballistic-trajectories-planet2.svg
https://commons.wikimedia.org/wiki/File:Ballistic-trajectories-planet2.svg rights: Creative Commons Attribution-Share Alike 4.0 International license |
| Width | 720 |
| Height | 440 |
