Jump to content

File:Cubicpoly.svg

Page contents not supported in other languages.
This is a file from the Wikimedia Commons
From Wikipedia, the free encyclopedia

Original file (SVG file, nominally 1,000 × 1,000 pixels, file size: 14 KB)

Summary

Description
English: Graph of the function y = x3 - 9x in range [-4,+4]
Date
Source Self-made using gnuplot and inkscape
Author Qualc1
SVG development
InfoField
 
The SVG code is valid.
 
This chart was created with Gnuplot.
 
 This plot uses embedded text that can be easily translated using a text editor.
Source code
InfoField

Gnuplot code

set terminal svg size 1000 1000 fixed enhanced fname 'Times'  fsize 36
set output "Cubicpoly.svg"

set samples 400

set autoscale fix
set xrange [-8:8]
set yrange [-17:17]

set grid
set xzeroaxis linewidth 2.5
set yzeroaxis linewidth 2.5

set key left

unset border
plot x**3-9*x lw 5

Licensing

I, the copyright holder of this work, hereby publish it under the following licenses:
GNU head Permission is granted to copy, distribute and/or modify this document under the terms of the GNU Free Documentation License, Version 1.2 or any later version published by the Free Software Foundation; with no Invariant Sections, no Front-Cover Texts, and no Back-Cover Texts. A copy of the license is included in the section entitled GNU Free Documentation License.
w:en:Creative Commons
attribution share alike 		This file is licensed under the Creative Commons Attribution-Share Alike 3.0 Unported license.
You are free:
  • to share – to copy, distribute and transmit the work
  • to remix – to adapt the work
Under the following conditions:
  • attribution – You must give appropriate credit, provide a link to the license, and indicate if changes were made. You may do so in any reasonable manner, but not in any way that suggests the licensor endorses you or your use.
  • share alike – If you remix, transform, or build upon the material, you must distribute your contributions under the same or compatible license as the original.
This licensing tag was added to this file as part of the GFDL licensing update.
w:en:Creative Commons
attribution share alike
This file is licensed under the Creative Commons Attribution-Share Alike 2.5 Generic license.
You are free:
  • to share – to copy, distribute and transmit the work
  • to remix – to adapt the work
Under the following conditions:
  • attribution – You must give appropriate credit, provide a link to the license, and indicate if changes were made. You may do so in any reasonable manner, but not in any way that suggests the licensor endorses you or your use.
  • share alike – If you remix, transform, or build upon the material, you must distribute your contributions under the same or compatible license as the original.
You may select the license of your choice.

Captions

Add a one-line explanation of what this file represents

Items portrayed in this file

depicts

inception

3 August 2006

media type

image/svg+xml

checksum

6d2b4e5e14ef7eee3fa7e986c636043e94343270

determination method or standard: SHA-1

data size

14,550 byte

height

1,000 pixel

width

1,000 pixel

File history

Click on a date/time to view the file as it appeared at that time.

Date/TimeThumbnailDimensionsUserComment
current20:06, 21 January 2010Thumbnail for version as of 20:06, 21 January 2010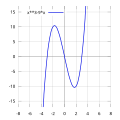1,000 × 1,000 (14 KB)BeaoChanged to supported font.
13:18, 6 December 2009Thumbnail for version as of 13:18, 6 December 20091,000 × 1,000 (15 KB)MakotoyI broke something. Revert to the prev. ver. while investigate the problem
13:15, 6 December 2009Thumbnail for version as of 13:15, 6 December 20091,000 × 1,000 (13 KB)Makotoyhand-optimized graph label for better thumbnail
13:34, 3 August 2006Thumbnail for version as of 13:34, 3 August 20061,000 × 1,000 (15 KB)Qualc1== Summary == {{Information| |Description=Function: <math>y=x^3 - 9*x</math> |Source=Self-made using gnuplot and inkscape |Date=2006-08-03 |Author=Qualc1 |Permission=See below |other_versions= - }} == Inst
No pages on the English Wikipedia use this file (pages on other projects are not listed).

Global file usage

The following other wikis use this file:

View more global usage of this file.

Metadata

This file contains additional information, probably added from the digital camera or scanner used to create or digitize it.

If the file has been modified from its original state, some details may not fully reflect the modified file.

Retrieved from "https://en.wikipedia.org/wiki/File:Cubicpoly.svg"