List of second moments of area

The following is list of area moments of inertia. The area moment of inertia or second moment of area has a unit of dimension length⁴, and should not be confused with the mass moment of inertia. Although if the piece is thin; the mass moment of inertia equals the areal density times the area moment of inertia. Each is with respect to a horizontal axis through the centroid of the given shape, unless otherwise specified.

Description	Figure	Area moment of inertia	Comment	Reference
a filled circular area of radius r		$I_{0}={\frac {\pi r^{4}}{4}}$		^[1]
an annulus of inner diameter d₁ and outer diameter d₂		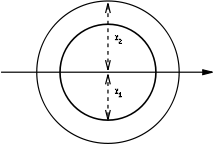$I_{0}={\frac {\pi }{64}}\left({d_{2}}^{4}-{d_{1}}^{4}\right)$	For thin tubes, this is approximately equal to: $\pi \left({\frac {{r_{2}}+{r_{1}}}{2}}\right)^{3}\left({r_{2}}-{r_{1}}\right)$ or $\pi {r}^{3}{t}$ .
a filled circular sector of angle θ in radians and radius r with respect to an axis through the centroid of the sector and the centre of the circle		$I_{0}=\left(\theta -\sin \theta \right){\frac {r^{4}}{8}}$
a filled semicircle with radius r with respect to a horizontal line passing through the centroid of the area		$I_{0}=\left({\frac {\pi }{8}}-{\frac {8}{9\pi }}\right)r^{4}=0.1098r^{4}$		^[2]
a filled semicircle as above but with respect to an axis collinear with the base		$I={\frac {\pi r^{4}}{8}}$	This is a consequence of the parallel axis theorem and the fact that the distance between these two axes is ${\frac {4r}{3\pi }}$	^[2]
a filled semicircle as above but with respect to a vertical axis through the centroid		$I_{0}={\frac {\pi r^{4}}{8}}$		^[2]
a filled quarter circle with radius r entirely in the 1st quadrant of the Cartesian coordinate system		$I={\frac {\pi r^{4}}{16}}$		^[3]
a filled quarter circle as above but with respect to a horizontal or vertical axis through the centroid		$I_{0}=\left({\frac {\pi }{16}}-{\frac {4}{9\pi }}\right)r^{4}$	This is a consequence of the parallel axis theorem and the fact that the distance between these two axes is ${\frac {4r}{3\pi }}$	^[3]
a filled ellipse whose radius along the x-axis is a and whose radius along the y-axis is b		$I_{0}={\frac {\pi }{4}}ab^{3}$
a filled rectangular area with a base width of b and height h		$I_{0}={\frac {bh^{3}}{12}}$		^[4]
a filled rectangular area as above but with respect to an axis collinear with the base		$I={\frac {bh^{3}}{3}}$	This is a result from the parallel axis theorem	^[4]
a filled rectangular area as above but with respect to an axis collinear with a distance x to the base away from the center(perpendicular to the axis)	This file may be deleted at any time.\|\| $I={\frac {bh^{3}}{3}}+bhx^{2}$ \|\|This is a result from the parallel axis theorem\|\|^[4]
a filled triangular area with a base width of b and height h with respect to an axis through the centroid		$I_{0}={\frac {bh^{3}}{36}}$		^[5]
a filled triangular area as above but with respect to an axis collinear with the base		$I={\frac {bh^{3}}{12}}$	This is a consequence of the parallel axis theorem	^[5]
a filled regular hexagon with a side length of a		$I_{0}={\frac {5{\sqrt {3}}}{16}}a^{4}$	The result is valid for both a horizontal and a vertical axis through the centroid, and therefore is also valid for an axis with arbitrary direction that passes through the origin.