Quadrifolium

The quadrifolium (also known as four-leaved clover^[1]) is a type of rose curve with n=2. It has polar equation:

r=\cos(2\theta ),\,

with corresponding algebraic equation

(x^{2}+y^{2})^{3}=(x^{2}-y^{2})^{2}.\,

Rotated by 45°, this becomes

r=\sin(2\theta )\,

with corresponding algebraic equation

(x^{2}+y^{2})^{3}=4x^{2}y^{2}.\,

In either form, it is a plane algebraic curve of genus zero.

The dual curve to the quadrifolium is

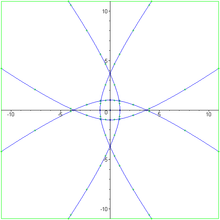
(x^{2}-y^{2})^{4}+837(x^{2}+y^{2})^{2}+108x^{2}y^{2}=16(x^{2}+7y^{2})(y^{2}+7x^{2})(x^{2}+y^{2})+729(x^{2}+y^{2}).\,

The area inside the curve is ${\tfrac {1}{2}}\pi$ , which is exactly half of the area of the circumcircle of the quadrifolium. The length of the curve is ca. 9.6884.^[2]

Notes

^ C G Gibson, Elementary Geometry of Algebraic Curves, An Undergraduate Introduction, Cambridge University Press, Cambridge, 2001, ISBN 978-0521-646413. Pages 92 and 93
^ http://mathworld.wolfram.com/Quadrifolium.html

References

J. Dennis Lawrence (1972). A catalog of special plane curves. Dover Publications. p. 175. ISBN 0-486-60288-5.

External links

Interactive example with JSXGraph

[1] C G Gibson, Elementary Geometry of Algebraic Curves, An Undergraduate Introduction, Cambridge University Press, Cambridge, 2001, ISBN 978-0521-646413. Pages 92 and 93

[2] ttp://mathworld.wolfram.com/Quadrifolium.html

[1]

[2]