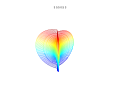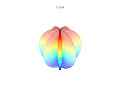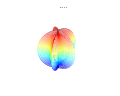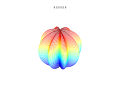Superformula

Some superformula samples: a = b = 1; m, n₁, n₂ and n₃ are shown in picture

The superformula is a generalization of the superellipse and was first proposed by Johan Gielis in 2003. Gielis suggested that the formula can be used to describe many complex shapes and curves that are found in nature.

In polar coordinates, with $r$ the radius and $\varphi$ the angle, the superformula is:

$r\left(\varphi \right)=\left(\left|{\frac {\cos \left({\frac {m\varphi }{4}}\right)}{a}}\right|^{n_{2}}+\left|{\frac {\sin \left({\frac {m\varphi }{4}}\right)}{b}}\right|^{n_{3}}\right)^{-{\frac {1}{n_{1}}}}.$

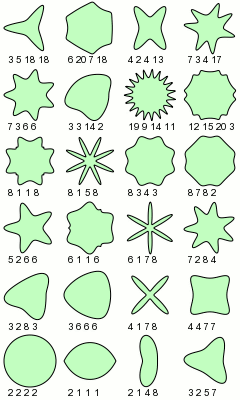
By choosing different values for the parameters $a,b,m,n_{1},n_{2},n_{3}$, different shapes can be generated.

The formula was obtained by generalizing the superellipse, named and popularized by Piet Hein, a Danish mathematician.

Extension to higher dimensions

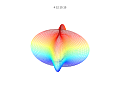
It is possible to extend the formula to 3, 4, or n dimensions, by means of the spherical product of superformulas. For example, the 3D parametric surface is obtained by multiplying two superformulas r₁ and r₂. The coordinates are defined by the relations:

$x=r_{1}(\theta )\cos \theta \cdot r_{2}(\phi )\cos \phi ,$

$y=r_{1}(\theta )\sin \theta \cdot r_{2}(\phi )\cos \phi ,$

$z=r_{2}(\phi )\sin \phi ,$

where $\phi$ (latitude) varies between −π/2 and π/2 and θ (longitude) between −π and π.

Plots

A GNU Octave program for generating these figures:

  function sf2d(n,a)
    u=[0:.001:2*pi];
    raux=abs(1/a(1).*abs(cos(n(1)*u/4))).^n(3)+abs(1/a(2).*abs(sin(n(1)*u/4))).^n(4);
    r=abs(raux).^(-1/n(2));
    x=r.*cos(u);
    y=r.*sin(u);
    plot(x,y);
  end

---

3D superformula: a = b = 1; m, n₁, n₂ and n₃ are shown in the pictures.

• 
• 
• 
• 
• 
• 
• 
• 

A GNU Octave program for generating these figures:

 function sf3d(n, a)
  u=[-pi:.05:pi];
  v=[-pi/2:.05:pi/2];
  nu=length(u);
  nv=length(v);
    for i=1:nu
    for j=1:nv
      raux1=abs(1/a(1)*abs(cos(n(1).*u(i)/4))).^n(3)+abs(1/a(2)*abs(sin(n(1)*u(i)/4))).^n(4);
      r1=abs(raux1).^(-1/n(2));
      raux2=abs(1/a(1)*abs(cos(n(1)*v(j)/4))).^n(3)+abs(1/a(2)*abs(sin(n(1)*v(j)/4))).^n(4);
      r2=abs(raux2).^(-1/n(2));
      x(i,j)=r1*cos(u(i))*r2*cos(v(j));
      y(i,j)=r1*sin(u(i))*r2*cos(v(j));
      z(i,j)=r2*sin(v(j));
    endfor;
  endfor;
  mesh(x,y,z);
 endfunction;

External links

• Website with information about the superformula and Johan Gielis
• Some Experiments on Fitting of Gielis Curves by Simulated Annealing and Particle Swarm Methods of Global Optimization
• Least Squares Fitting of Chacón-Gielis Curves By the Particle Swarm Method of Optimization
• Superformula 2D Plotter & SVG Generator
• Interactive example using JSXGraph
• 3D Superdupershape Explorer using Processing
• Interactive 3D Superformula plotter using Processing (with code)
• SuperShaper: An OpenSource, OpenCL accelerated, interactive 3D SuperShape generator with shader based visualisation (OpenGL3)
• Simpel, WebGL based SuperShape implementation
• The Gielis Supershape Formula, Provides an interactive Java applet for exploring the variety of different shapes, natural or mathematical, that can be formed with the formula.