From Wikipedia, the free encyclopedia
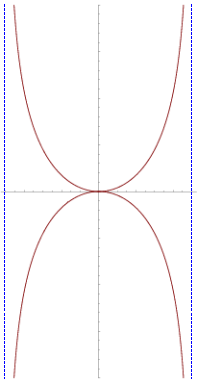
The kappa curve has two vertical asymptotes In geometry , the kappa curve or Gutschoven's curve is a two-dimensional algebraic curve resembling the Greek letter ϰ (kappa)Gérard van Gutschoven around 1662. In the history of mathematics , it is remembered as one of the first examples of Isaac Barrow 's application of rudimentary calculus methods to determine the tangent of a curve. Isaac Newton and Johann Bernoulli continued the studies of this curve subsequently.
Using the Cartesian coordinate system it can be expressed as
x
2
(
x
2
+
y
2
)
=
a
2
y
2
{\displaystyle x^{2}\left(x^{2}+y^{2}\right)=a^{2}y^{2}}
or, using parametric equations ,
x
=
a
sin
t
,
y
=
a
sin
t
tan
t
.
{\displaystyle {\begin{aligned}x&=a\sin t,\\y&=a\sin t\tan t.\end{aligned}}}
In polar coordinates its equation is even simpler:
r
=
a
tan
θ
.
{\displaystyle r=a\tan \theta .}
It has two vertical asymptotes at x = ±a
The kappa curve's curvature :
κ
(
θ
)
=
8
(
3
−
sin
2
θ
)
sin
4
θ
a
(
sin
2
(
2
θ
)
+
4
)
3
2
.
{\displaystyle \kappa (\theta )={\frac {8\left(3-\sin ^{2}\theta \right)\sin ^{4}\theta }{a\left(\sin ^{2}(2\theta )+4\right)^{\frac {3}{2}}}}.}
Tangential angle:
ϕ
(
θ
)
=
−
arctan
(
1
2
sin
(
2
θ
)
)
.
{\displaystyle \phi (\theta )=-\arctan \left({\tfrac {1}{2}}\sin(2\theta )\right).}
Tangents via infinitesimals [ edit ] The tangent lines of the kappa curve can also be determined geometrically using differentials and the elementary rules of infinitesimal arithmetic. Suppose x and y are variables, while a is taken to be a constant. From the definition of the kappa curve,
x
2
(
x
2
+
y
2
)
−
a
2
y
2
=
0
{\displaystyle x^{2}\left(x^{2}+y^{2}\right)-a^{2}y^{2}=0}
Now, an infinitesimal change in our location must also change the value of the left hand side, so
d
(
x
2
(
x
2
+
y
2
)
−
a
2
y
2
)
=
0
{\displaystyle d\left(x^{2}\left(x^{2}+y^{2}\right)-a^{2}y^{2}\right)=0}
Distributing the differential and applying appropriate rules ,
d
(
x
2
(
x
2
+
y
2
)
)
−
d
(
a
2
y
2
)
=
0
(
2
x
d
x
)
(
x
2
+
y
2
)
+
x
2
(
2
x
d
x
+
2
y
d
y
)
−
a
2
2
y
d
y
=
0
(
4
x
3
+
2
x
y
2
)
d
x
+
(
2
y
x
2
−
2
a
2
y
)
d
y
=
0
x
(
2
x
2
+
y
2
)
d
x
+
y
(
x
2
−
a
2
)
d
y
=
0
x
(
2
x
2
+
y
2
)
y
(
a
2
−
x
2
)
=
d
y
d
x
{\displaystyle {\begin{aligned}d\left(x^{2}\left(x^{2}+y^{2}\right)\right)-d\left(a^{2}y^{2}\right)&=0\\[6px](2x\,dx)\left(x^{2}+y^{2}\right)+x^{2}(2x\,dx+2y\,dy)-a^{2}2y\,dy&=0\\[6px]\left(4x^{3}+2xy^{2}\right)dx+\left(2yx^{2}-2a^{2}y\right)dy&=0\\[6px]x\left(2x^{2}+y^{2}\right)dx+y\left(x^{2}-a^{2}\right)dy&=0\\[6px]{\frac {x\left(2x^{2}+y^{2}\right)}{y\left(a^{2}-x^{2}\right)}}&={\frac {dy}{dx}}\end{aligned}}}
If we use the modern concept of a functional relationship y (x )implicit differentiation , the slope of a tangent line to the kappa curve at a point (x ,y ) is:
2
x
(
x
2
+
y
2
)
+
x
2
(
2
x
+
2
y
d
y
d
x
)
=
2
a
2
y
d
y
d
x
2
x
3
+
2
x
y
2
+
2
x
3
=
2
a
2
y
d
y
d
x
−
2
x
2
y
d
y
d
x
4
x
3
+
2
x
y
2
=
(
2
a
2
y
−
2
x
2
y
)
d
y
d
x
2
x
3
+
x
y
2
a
2
y
−
x
2
y
=
d
y
d
x
{\displaystyle {\begin{aligned}2x\left(x^{2}+y^{2}\right)+x^{2}\left(2x+2y{\frac {dy}{dx}}\right)&=2a^{2}y{\frac {dy}{dx}}\\[6px]2x^{3}+2xy^{2}+2x^{3}&=2a^{2}y{\frac {dy}{dx}}-2x^{2}y{\frac {dy}{dx}}\\[6px]4x^{3}+2xy^{2}&=\left(2a^{2}y-2x^{2}y\right){\frac {dy}{dx}}\\[6px]{\frac {2x^{3}+xy^{2}}{a^{2}y-x^{2}y}}&={\frac {dy}{dx}}\end{aligned}}}








\left(x^{2}+y^{2}\right)+x^{2}(2x\,dx+2y\,dy)-a^{2}2y\,dy&=0\\[6px]\left(4x^{3}+2xy^{2}\right)dx+\left(2yx^{2}-2a^{2}y\right)dy&=0\\[6px]x\left(2x^{2}+y^{2}\right)dx+y\left(x^{2}-a^{2}\right)dy&=0\\[6px]{\frac {x\left(2x^{2}+y^{2}\right)}{y\left(a^{2}-x^{2}\right)}}&={\frac {dy}{dx}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e2fbbee06e516acb095da379a192f66d2c38e956)
![{\displaystyle {\begin{aligned}2x\left(x^{2}+y^{2}\right)+x^{2}\left(2x+2y{\frac {dy}{dx}}\right)&=2a^{2}y{\frac {dy}{dx}}\\[6px]2x^{3}+2xy^{2}+2x^{3}&=2a^{2}y{\frac {dy}{dx}}-2x^{2}y{\frac {dy}{dx}}\\[6px]4x^{3}+2xy^{2}&=\left(2a^{2}y-2x^{2}y\right){\frac {dy}{dx}}\\[6px]{\frac {2x^{3}+xy^{2}}{a^{2}y-x^{2}y}}&={\frac {dy}{dx}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ba914f68cdf1eee7a64e63203b830e54b63a6522)