Pythagorean tiling: Difference between revisions
given cnotent disagreements please discuss on talk first |
|||
| Line 4: | Line 4: | ||
==Topology and symmetry== |
==Topology and symmetry== |
||
[[File:A pattern of Pythagorean tiling.svg|thumb|upright=1.3|left|A square in dashed red is indefinitely repeated horizontally and vertically: the tiling is periodic.]] |
|||
The Pythagorean tiling is the unique tiling by squares of two different sizes that is both ''unilateral'' (no two squares have a common side) and ''equitransitive'' (each two squares of the same size can be mapped into each other by a symmetry of the tiling).<ref name="mms">{{citation |
The Pythagorean tiling is the unique tiling by squares of two different sizes that is both ''unilateral'' (no two squares have a common side) and ''equitransitive'' (each two squares of the same size can be mapped into each other by a symmetry of the tiling).<ref name="mms">{{citation |
||
| last1 = Martini | first1 = Horst |
| last1 = Martini | first1 = Horst |
||
| Line 28: | Line 29: | ||
==Pythagorean theorem and dissections== |
==Pythagorean theorem and dissections== |
||
{{Merge to |Pythagorean theorem#Proofs |discuss=Talk:Pythagorean_tiling#Proof_of_theorem |date=November 2012}} |
|||
[[File:Pythagorean dissections.svg|thumb|300px|left|The five-piece dissections used in the proofs by [[Al-Nayrizi]] and [[Thābit ibn Qurra]] (left) and by [[Henry Perigal]] (right)]] |
|||
{| style="margin:1em 0em" |
|||
| ⚫ | |||
|- |
|||
| colspan="2" style="padding:0.5em;padding-left:180px"| First position of the grid relative to the tiling. |
|||
|- |
|||
| style="padding:0.5em 1em 2em 1em"|[[File:Academ Pythagorean tiling and Pythagorean theorem.svg|frameless|360px|left]]<br/>Case where the initial triangle is not isosceles. |
|||
| style="vertical-align:top;padding:0.5em 1em"|[[File:Academ Pythagorean theorem in case of isosceles right triangle.svg|frameless|360px|left]]{{clear}}Particular case where the initial triangle is isosceles. |
|||
|- |
|||
| style="padding:0.5em 1em" |[[File:A tiling and a proof of the Pythagorean theorem.svg|frameless|360px|left]]<br/>Second position of the grid relative to the tiling. |
|||
| style="vertical-align:top;padding:0.5em 1em" | A right triangle is given, from which a tiling is created. Then a grid is positioned on the tiling, depicted in dashed red. The size of its repeated squares is the hypotenuse length of the initial triangle, denoted by ''c.'' Its first position relative to the tiling determines four or five puzzle pieces, depending on whether the initial triangle is isosceles or not. The puzzle pieces form together either two tiles or one square element of the grid. These two assemblages have {{Nowrap|1=equal areas: ''a''<sup> 2</sup> + ''b''<sup> 2</sup> = ''c''<sup> 2</sup>, }} hence the Pythagorean theorem. |
|||
|} |
|||
{{clear}} |
|||
[[File:Pythagorean dissections.svg|thumb|300px|left]] |
|||
| ⚫ | The image shows the five-piece dissections used in the proofs of the Pythagorean theorem by the ninth-century Arabic mathematicians [[Al-Nayrizi]] and [[Thābit ibn Qurra]] (left), and by the 19th-century British amateur mathematician [[Henry Perigal]] (right).<ref name="rbn">{{citation|title=Paintings, plane tilings, and proofs|first=Roger B.|last=Nelsen|journal=Math Horizons|date=November 2003|pages=5–8|url=http://www-stat.wharton.upenn.edu/~steele/Publications/Books/CSMC/New%20Problems/paintings.pdf}}. Reprinted in {{citation|title=The Edge of the Universe: Celebrating Ten Years of Math Horizons|series=Spectrum Series|publisher=Mathematical Association of America|first1=Deanna|last1=Haunsperger|first2=Stephen|last2=Kennedy|year=2007|isbn=978-0-88385-555-3|pages=295–298}}. See also {{citation|title=Charming proofs: a journey into elegant mathematics|volume=42|series=Dolciani mathematical expositions|first1=Claudi|last1=Alsina|first2=Roger B.|last2=Nelsen|publisher=Mathematical Association of America|year=2010|isbn=978-0-88385-348-1|pages=168–169}}.</ref><ref name="gnf">{{citation|title=Dissections: Plane & Fancy|first=Greg N.|last=Frederickson|pages=30–31|publisher=Cambridge University Press|year=1997}}.</ref><ref>{{citation |
||
| last1 = Aguiló | first1 = Francesc |
| last1 = Aguiló | first1 = Francesc |
||
| last2 = Fiol | first2 = Miquel Angel |
| last2 = Fiol | first2 = Miquel Angel |
||
| Line 40: | Line 53: | ||
| title = Periodic tilings as a dissection method |
| title = Periodic tilings as a dissection method |
||
| volume = 107 |
| volume = 107 |
||
| year = 2000}}.</ref><ref>{{harvtxt|Grünbaum|Shephard|1987}}, p. 94.</ref> If the sides of the two squares forming the tiling are the numbers ''a'' and ''b'', then the closest distance between corresponding points on congruent squares is ''c'', where ''c'' is the length of the [[hypotenuse]] of a [[right triangle]] having sides ''a'' and ''b'' |
| year = 2000}}.</ref><ref>{{harvtxt|Grünbaum|Shephard|1987}}, p. 94.</ref> If the sides of the two squares forming the tiling are the numbers ''a'' and ''b'', then the closest distance between corresponding points on congruent squares is ''c'', where ''c'' is the length of the [[hypotenuse]] of a [[right triangle]] having sides ''a'' and ''b''. |
||
By overlaying a square grid of side length ''c'' onto the Pythagorean tiling, it may be used to generate a five-piece [[Dissection problem|dissection]] of two unequal squares of sides ''a'' and ''b'' into a single square of side ''c'', showing that the two smaller squares have the same area as the larger one. |
By overlaying a square grid of side length ''c'' onto the Pythagorean tiling, it may be used to generate a five-piece [[Dissection problem|dissection]] of two unequal squares of sides ''a'' and ''b'' into a single square of side ''c'', showing that the two smaller squares have the same area as the larger one. |
||
== Aperiodic cross sections == |
== Aperiodic cross sections == |
||
Revision as of 17:15, 29 November 2012
A Pythagorean tiling or two squares tessellation is a tessellation of an Euclidean plane by squares of two different sizes, in which each square touches four squares of the other size on its four sides. Because of numerous proofs of the Pythagorean theorem based on such a tiling,[2] it is called a Pythagorean tiling.[1] It also is commonly used as a pattern for floor tiles; in this context it is also known as a hopscotch pattern.[3]
Topology and symmetry
The Pythagorean tiling is the unique tiling by squares of two different sizes that is both unilateral (no two squares have a common side) and equitransitive (each two squares of the same size can be mapped into each other by a symmetry of the tiling).[4]
Topologically, the Pythagorean tiling has the same structure as the truncated square tiling by squares and regular octagons.[5] The smaller squares in the Pythagorean tiling are adjacent to four larger tiles, as are the squares in the truncated square tiling, while the larger squares in the Pythagorean tiling are adjacent to eight neighbors that alternate between large and small, just as the octagons in the truncated square tiling. However, the two tilings have different sets of symmetries: the truncated square tiling has dihedral symmetry around the center of each tile, while the Pythagorean tiling has a smaller cyclic set of symmetries around the corresponding points, giving it p4 symmetry.[6] It is a chiral pattern, meaning that it is impossible to superpose it on top of its mirror image using only translations and rotations.
A uniform tiling is a tiling in which each tile is a regular polygon and in which there is a symmetry, mapping every vertex to every other vertex. Usually, uniform tilings additionally are required to have tiles that meet edge-to-edge, but if this requirement is relaxed then there are eight additional uniform tilings: four formed from infinite strips of squares or equilateral triangles, three formed from equilateral triangles and regular hexagons, and one more, the Pythagorean tiling.[7]
Pythagorean theorem and dissections
 | It has been suggested that this article be merged into Pythagorean theorem#Proofs. (Discuss) Proposed since November 2012. |
| First position of the grid relative to the tiling. | |
 Case where the initial triangle is not isosceles. |
 |
 Second position of the grid relative to the tiling. |
A right triangle is given, from which a tiling is created. Then a grid is positioned on the tiling, depicted in dashed red. The size of its repeated squares is the hypotenuse length of the initial triangle, denoted by c. Its first position relative to the tiling determines four or five puzzle pieces, depending on whether the initial triangle is isosceles or not. The puzzle pieces form together either two tiles or one square element of the grid. These two assemblages have equal areas: a 2 + b 2 = c 2, hence the Pythagorean theorem. |
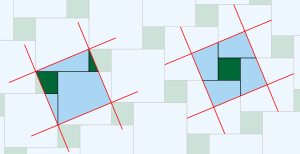
The image shows the five-piece dissections used in the proofs of the Pythagorean theorem by the ninth-century Arabic mathematicians Al-Nayrizi and Thābit ibn Qurra (left), and by the 19th-century British amateur mathematician Henry Perigal (right).[1][8][9][10] If the sides of the two squares forming the tiling are the numbers a and b, then the closest distance between corresponding points on congruent squares is c, where c is the length of the hypotenuse of a right triangle having sides a and b.
By overlaying a square grid of side length c onto the Pythagorean tiling, it may be used to generate a five-piece dissection of two unequal squares of sides a and b into a single square of side c, showing that the two smaller squares have the same area as the larger one.
Aperiodic cross sections
Although the Pythagorean tiling is itself periodic (it has a square lattice of translational symmetries) its sections can be used to generate one-dimensional aperiodic sequences.[11]
In the "Klotz construction" for aperiodic sequences (Klotz is a German word for a block), one forms a Pythagorean tiling with two squares for which the ratio between the two side lengths is an irrational number x. Then, one chooses a line parallel to the sides of the squares, and forms a sequence of binary values from the sizes of the squares crossed by the line: a 0 corresponds to a crossing of a large square and a 1 corresponds to a crossing of a small square. In this sequence, the relative proportion of 0s and 1s will be in the ratio x:1. This proportion cannot be achieved by a periodic sequence of 0s and 1s, because it is irrational, so the sequence is aperiodic.[11]
If x is chosen as the golden ratio, the sequence of 0s and 1s generated in this way has the same recursive structure as the Fibonacci word: it can be split into substrings of the form "01" and "0" (that is, there are no two consecutive ones) and if these two substrings are consistently replaced by the shorter strings "0" and "1" then another string with the same structure results.[11]
Related results
According to Keller's conjecture, any tiling of the plane by congruent squares must include two squares that meet edge-to-edge.[12] None of the squares in the Pythagorean tiling meet edge-to-edge,[4] but this fact does not violate Keller's conjecture because the tiles are not all congruent to each other.
The Pythagorean tiling may be generalized to a three-dimensional tiling of Euclidean space by cubes of two different sizes, which also is unilateral and equitransitive. Attila Bölcskei calls this three-dimensional tiling the Rogers filling. He conjectures that, in any dimension greater than three, there is again a unique unilateral and equitransitive way of tiling space by hypercubes of two different sizes.[13]
Burns and Rigby found several prototiles, including the Koch snowflake, that may be used to tile the plane only by using copies of the prototile in two or more different sizes.[14] An earlier paper by Danzer, Grünbaum, and Shephard provides another example, a convex pentagon that tiles the plane only when combined in two sizes.[15] Although the Pythagorean tiling uses two different sizes of squares, the square does not have the same property as these prototiles of only tiling by similarity, because it also is possible to tile the plane using only squares of a single size.
References
- ^ a b c Nelsen, Roger B. (November 2003), "Paintings, plane tilings, and proofs" (PDF), Math Horizons: 5–8. Reprinted in Haunsperger, Deanna; Kennedy, Stephen (2007), The Edge of the Universe: Celebrating Ten Years of Math Horizons, Spectrum Series, Mathematical Association of America, pp. 295–298, ISBN 978-0-88385-555-3. See also Alsina, Claudi; Nelsen, Roger B. (2010), Charming proofs: a journey into elegant mathematics, Dolciani mathematical expositions, vol. 42, Mathematical Association of America, pp. 168–169, ISBN 978-0-88385-348-1.
- ^ Wells, David (1991), "two squares tessellation", The Penguin Dictionary of Curious and Interesting Geometry, New York: Penguin Books, pp. 260–261, ISBN 0-14-011813-6.
- ^ Hopscotch: It's more than a kid's game (PDF), Tile Inc., August 2008.
- ^ a b Martini, Horst; Makai, Endre; Soltan, Valeriu (1998), "Unilateral tilings of the plane with squares of three sizes", Beiträge zur Algebra und Geometrie, 39 (2): 481–495, MR 1642720.
- ^ Grünbaum, Branko; Shephard, G. C. (1987), Tilings and Patterns, W. H. Freeman, p. 171.
- ^ Grünbaum & Shephard (1987), p. 42.
- ^ Grünbaum & Shephard (1987), pp. 73–74.
- ^ Frederickson, Greg N. (1997), Dissections: Plane & Fancy, Cambridge University Press, pp. 30–31.
- ^ Aguiló, Francesc; Fiol, Miquel Angel; Fiol, Maria Lluïsa (2000), "Periodic tilings as a dissection method", American Mathematical Monthly, 107 (4): 341–352, doi:10.2307/2589179, MR 1763064.
- ^ Grünbaum & Shephard (1987), p. 94.
- ^ a b c Steurer, Walter; Deloudi, Sofia (2009), "3.5.3.7 The Klotz construction", Crystallography of Quasicrystals: Concepts, Methods and Structures, Springer Series in Materials Science, vol. 126, Springer, pp. 91–92, doi:10.1007/978-3-642-01899-2, ISBN 978-3-642-01898-5.
- ^ The truth of his conjecture for two-dimensional tilings was known already to Keller, but it was since proven false for dimensions eight and above. For a recent survey on results related to this conjecture, see Zong, Chuanming (2005), "What is known about unit cubes", Bulletin of the American Mathematical Society, New Series, 42 (2): 181–211, doi:10.1090/S0273-0979-05-01050-5, MR 2133310.
- ^ Bölcskei, Attila (2001), "Filling space with cubes of two sizes", Publicationes Mathematicae Debrecen, 59 (3–4): 317–326, MR 1874434. See also Dawson (1984), which includes an illustration of the three-dimensional tiling, credited to "Rogers" but cited to a 1960 paper by Richard K. Guy: Dawson, R. J. M. (1984), "On filling space with different integer cubes", Journal of Combinatorial Theory. Series A, 36 (2): 221–229, doi:10.1016/0097-3165(84)90007-4, MR 0734979.
- ^ Burns, Aidan (1994), "78.13 Fractal tilings", Mathematical Gazette, 78 (482): 193–196, JSTOR 3618577. Rigby, John (1995), "79.51 Tiling the plane with similar polygons of two sizes", Mathematical Gazette, 79 (486): 560–561, JSTOR 3618091.
- ^ Figure 3 of Danzer, Ludwig; Grünbaum, Branko; Shephard, G. C. (1982), "Unsolved Problems: Can All Tiles of a Tiling Have Five-Fold Symmetry?", The American Mathematical Monthly, 89 (8): 568–570+583–585, doi:10.2307/2320829, MR 1540019.
