Blueshift

This redirect is about the physical phenomenon. For the term as used in photochemistry, see hypsochromic shift. For other uses of "blueshift" or "blue shift", see Blueshift (disambiguation).

A blueshift is any decrease in wavelength, with a corresponding increase in frequency, of an electromagnetic wave; the opposite effect is referred to as redshift. In visible light, this shifts the color from the red end of the spectrum to the blue end.

Doppler blueshift

Doppler redshift and blueshift

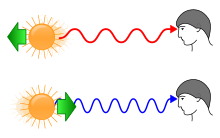
Doppler blueshift is caused by movement of a source towards the observer. The term applies to any decrease in wavelength and increase in frequency caused by relative motion, even outside the visible spectrum. Only objects moving at near-relativistic speeds toward the observer are noticeably bluer to the naked eye, but the wavelength of any reflected or emitted photon or other particle is shortened in the direction of travel.

Doppler blueshift is used in astronomy to determine relative motion:

• The Andromeda Galaxy is moving toward our own Milky Way galaxy within the Local Group; thus, when observed from Earth, its light is undergoing a blueshift.
• Components of a binary star system will be blueshifted when moving towards Earth
• When observing spiral galaxies, the side spinning toward us will have a slight blueshift relative to the side spinning away from us (see Tully–Fisher relation).
• Blazars are known to propel relativistic jets toward us, emitting synchrotron radiation and bremsstrahlung that appears blueshifted.
• Nearby stars such as Barnard's Star are moving toward us, resulting in a very small blueshift.
• Doppler blueshift of distant objects with a high z can be subtracted from the much larger cosmological redshift to determine relative motion in the expanding universe.

Gravitational blueshift

Matter waves (protons, electrons, photons, etc.) falling into a gravity well become more energetic and undergo observer-independent blueshifting.

Unlike the relative Doppler blueshift, caused by movement of a source towards the observer and thus dependent on the received angle of the photon, gravitational blueshift is absolute and does not depend on the received angle of the photon:

Photons climbing out of a gravitating object become less energetic. This loss of energy is known as a "redshifting", as photons in the visible spectrum would appear more red. Similarly, photons falling into a gravitational field become more energetic and exhibit a blueshifting. ... Note that the magnitude of the redshifting (blueshifting) effect is not a function of the emitted angle or the received angle of the photon—it depends only on how far radially the photon had to climb out of (fall into) the potential well.

It is a consequence of conservation of energy—as a matter wave descends into a gravitational potential well, its potential energy becomes more negative, while its actual energy becomes more positive. Since actual energy is E = hf, where h is a constant, it can be made more positive only by increasing f.

At the bottom of a gravity well, all matter waves have higher frequencies than control matter waves outside the gravity well. When such a blueshifted matter wave climbs out of the gravity well, its frequency decreases to a "normal" level, so that comparing its frequency with the frequency of a control matter wave will not show any reddening. An observer at the bottom of a gravity well cannot observe any blueshift of incoming matter waves, because the observer is himself blueshifted. Thus, gravitational redshift and gravitational blueshift are not directly observable.

Cosmological blueshift

Any matter wave is falling into its own gravitational potential well, and a matter wave's free-fall timescale t_ff is inversely proportional to the matter wave's density ρ:

$t_{\mathrm {ff} }=\left({\frac {3\pi }{32G\rho }}\right)^{1/2}.$ (13.8) Equation (13.8) implies that the denser clumps collapse more rapidly, which may lead to separation and fragmentation. As cores are observed to be densest near their centers, the interiors cave in most quickly, producing an inside-out collapse.

Thus, the self-gravitational blueshift of protons proceeds increasingly faster than the self-gravitational blueshift of the ambient photon bath, so that from the perspective of an observer made of atoms, the ambient photon bath appears to be in the process of accelerating expansion and cosmological Doppler redshift, whereas in reality it is in the process of accelerating contraction and self-gravitational blueshift, but at a progressively lower rate compared with atoms.

See also

• Gravitational potential
• Redshift
• Relativistic Doppler effect