Möbius–Kantor polygon

Möbius–Kantor polygon
Orthographic projection shown here with 4 red and 4 blue 3-edge triangles.
Shephard symbol	3(24)3
Schläfli symbol	3{3}3
Coxeter diagram
Edges	8 3{}
Vertices	8
Petrie polygon	Octagon
Shephard group	3[3]3, order 24
Dual polyhedron	Self-dual
Properties	Regular

In geometry, the Möbius–Kantor polygon is a regular complex polygon ₃{3}₃, , in $\mathbb {C} ^{2}$. ₃{3}₃ has 8 vertices, and 8 edges. It is self-dual. Every vertex is shared by 3 triangular edges. Coxeter named it a Möbius–Kantor polygon for sharing the complex configuration structure as the Möbius–Kantor configuration, (8₃).

Discovered by G.C. Shephard in 1952, he represented it as 3(24)3, with its symmetry, Coxeter called as ₃[3]₃, isomorphic to the binary tetrahedral group, order 24.

The 8 vertex coordinates of this polygon can be given in $\mathbb {C} ^{3}$, as:

(ω,−1,0)	(0,ω,−ω2)	(ω2,−1,0)	(−1,0,1)
(−ω,0,1)	(0,ω2,−ω)	(−ω2,0,1)	(1,−1,0)

where $\omega ={\tfrac {-1+i{\sqrt {3}}}{2}}$.

Real representation

It has a real representation as the 16-cell, , in 4-dimensional space, sharing the same 8 vertices. The 24 edges in the 16-cell are seen in the Möbius–Kantor polygon when the 8 triangular edges are drawn as 3-separate edges. The triangles are represented 2 sets of 4 red or blue outlines. The B₄ projections are given in two different symmetry orientations between the two color sets.

orthographic projections

Plane	B4		F4
Graph
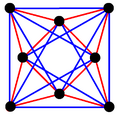
Symmetry	[8]		[12/3]

Related polytopes

This graph shows the two alternated polygons as a compound in red and blue 3{3}3 in dual positions.

3{6}2, or , with 24 vertices in black, and 16 3-edges colored in 2 sets of 3-edges in red and blue.

It can also be seen as an alternation of , represented as . has 16 vertices, and 24 edges. A compound of two, in dual positions, and , can be represented as , contains all 16 vertices of .

The truncation , is the same as the regular polygon, ₃{6}₂, . Its edge-diagram is the cayley diagram for ₃[3]₃.

The regular Hessian polyhedron ₃{3}₃{3}₃, has this polygon as a facet and vertex figure.

References

• Shephard, G.C.; Regular complex polytopes, Proc. London math. Soc. Series 3, Vol 2, (1952), pp 82–97.
• Coxeter, H. S. M. and Moser, W. O. J.; Generators and Relations for Discrete Groups (1965), esp pp 67–80.
• Coxeter, H. S. M.; Regular Complex Polytopes, Cambridge University Press, (1974), second edition (1991).
• Coxeter, H. S. M. and Shephard, G.C.; Portraits of a family of complex polytopes, Leonardo Vol 25, No 3/4, (1992), pp 239–244 [1]