Secant line
A secant line of a curve is a line that intersects two (or more) points on the curve. The word secant comes from the Latin secare, for to cut.
It can be used to approximate the tangent to a curve, at some point P. If the secant to a curve is defined by two points, P and Q, with P fixed and Q variable, as Q approaches P along the curve, the direction of the secant approaches that of the tangent at P (assuming there is just one).
As a consequence, one could say that the limit of the secant's slope, or direction, is that of the tangent.
How the secant function is related to secant lines
Consider some angle θ. Draw a secant line to the unit circle (centered at the origin) that passes through the origin and through (cos θ, sin θ), the point on the unit circle at an angle of θ. The absolute value of the trigonometric secant of θ is equal to the length of a segment of the secant line running from the origin to the line x = 1. If the segment passes through the point (cos θ, sin θ), then the trigonometric secant of θ is positive; if it passes through the antipodal point, then the secant of θ is negative.
Secant approximation
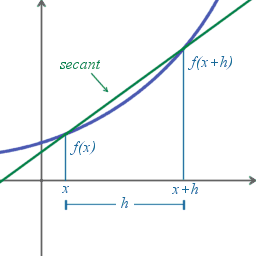
Consider the curve defined by y = f(x) in a Cartesian coordinate system, and consider a point P with coordinates (c, f(c)) and another point Q with coordinates (c + Δx, f(c + Δx)). Then the slope m of the secant line, through P and Q, is given by:
The righthand side of the above equation is a variation of Newton's difference quotient. As Δx approaches zero, this expression approaches the derivative of f(c), assuming a derivative exists.

