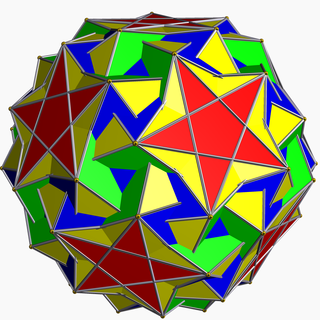
Snub icosidodecadodecahedron

Snub icosidodecadodecahedron
Type	Uniform star polyhedron
Elements	F = 104, E = 180 V = 60 (χ = −16)
Faces by sides	(20+60){3}+12{5}+12{5/2}
Coxeter diagram
Wythoff symbol	| 5/3 3 5
Symmetry group	I, [5,3]+, 532
Index references	U46, C58, W112
Dual polyhedron	Medial hexagonal hexecontahedron
Vertex figure	3.3.3.5.3.5/3
Bowers acronym	Sided

3D model of a snub icosidodecadodecahedron

In geometry, the snub icosidodecadodecahedron is a nonconvex uniform polyhedron, indexed as U₄₆. It has 104 faces (80 triangles, 12 pentagons, and 12 pentagrams), 180 edges, and 60 vertices. As the name indicates, it belongs to the family of snub polyhedra.

The circumradius of the snub icosidodecadodecahedron with unit edge length is

${\frac {1}{2}}{\sqrt {\frac {2\rho -1}{\rho -1}}},$

where ρ is the plastic constant, or the unique real root of ρ³ = ρ + 1.

Cartesian coordinates

Cartesian coordinates for the vertices of a snub icosidodecadodecahedron are all the even permutations of

(±2α, ±2γ, ±2β),

(±(α+β/τ+γτ), ±(-ατ+β+γ/τ), ±(α/τ+βτ-γ)),

(±(-α/τ+βτ+γ), ±(-α+β/τ-γτ), ±(ατ+β-γ/τ)),

(±(-α/τ+βτ-γ), ±(α-β/τ-γτ), ±(ατ+β+γ/τ)) and

(±(α+β/τ-γτ), ±(ατ-β+γ/τ), ±(α/τ+βτ+γ))

with an even number of plus signs, where τ = (1+√5)/2 is the golden ratio; ρ is the plastic constant, or the unique real solution to ρ³=ρ+1;

α = ρ+1 = ρ³;

β = τ²ρ⁴+τ; and

γ = ρ²+τρ.

Taking the odd permutations of the above coordinates with an odd number of plus signs gives another form, the enantiomorph of the other one.

Related polyhedra

Medial hexagonal hexecontahedron

Medial hexagonal hexecontahedron
Type	Star polyhedron
Face
Elements	F = 60, E = 180 V = 104 (χ = −16)
Symmetry group	I, [5,3]+, 532
Index references	DU46
dual polyhedron	Snub icosidodecadodecahedron

3D model of a medial hexagonal hexecontahedron

The medial hexagonal hexecontahedron is a nonconvex isohedral polyhedron. It is the dual of the uniform snub icosidodecadodecahedron.

See also

• List of uniform polyhedra

References

• Wenninger, Magnus (1983), Dual Models, Cambridge University Press, ISBN 978-0-521-54325-5, MR 0730208

External links

• Weisstein, Eric W. "Medial hexagonal hexecontahedron". MathWorld.