Synchronous condenser
In electrical engineering, a synchronous condenser (sometimes called a synchronous capacitor or synchronous compensator) is a device identical to a synchronous motor, whose shaft is not connected to anything but spins freely.[1] Its purpose is not to convert electric power to mechanical power or vice versa, but to adjust conditions on the electric power transmission grid. Its field is controlled by a voltage regulator to either generate or absorb reactive power as needed to adjust the grid's voltage, or to improve power factor. The condenser’s installation and operation are identical to large electric motors and generators.
Increasing the device's field excitation results in its furnishing reactive power (vars) to the system. Its principal advantage is the ease with which the amount of correction can be adjusted. The kinetic energy stored in the rotor of the machine can help stabilize a power system during rapid fluctuations of loads such as those created by short circuits or electric arc furnaces. Large installations of synchronous condensers are sometimes used in association with high-voltage direct current converter stations to supply reactive power to the alternating current grid.
Unlike a capacitor bank, the amount of reactive power from a synchronous condenser can be continuously adjusted. Reactive power from a capacitor bank decreases when grid voltage decreases, while a synchronous condenser can increase reactive current as voltage decreases. However, synchronous machines have higher energy losses than static capacitor banks.[1] Most synchronous condensers connected to electrical grids are rated between 20 MVAr (megavars) and 200 MVAr and many are hydrogen cooled. There is no explosion hazard as long as the hydrogen concentration is maintained above 70%, typically above 91%.[2]
Theory
A rotating coil [3] in a magnetic field tends to produce a sine-wave voltage. When connected to a circuit some current will flow depending on how the voltage on the system is different from this open-circuit voltage. Note that mechanical torque (produced by a motor, required by a generator) corresponds only to the real power. Reactive power does not result in any torque.
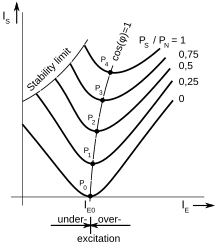
As the mechanical load on a synchronous motor increases, the stator current Ia increases regardless of the field excitation. For both under and over excited motors, the power factor (p.f.) tends to approach unity with increase in mechanical load. This change in power factor is larger than the change in Ia with increase in load.
The phase of armature current varies with field excitation. The current has larger values for lower and higher values of excitation. In between, the current has minimum value corresponding to a particular excitation (see graph on right). The variations of I with excitation are known as V curves because of their shape.
For the same mechanical load, the armature current varies with field excitation over a wide range and so causes the power factor also to vary accordingly. When over-excited, the motor runs with leading power factor (and supplies vars to the grid) and when under-excited with lagging power factor (and absorbs vars from the grid). In between, the power factor is unity. The minimum armature current corresponds to the point of unity power factor (voltage and current in phase).
As in a synchronous motor, the stator of the machine is connected to a three-phase supply of voltage Vs (assumed to be constant), and this creates a rotating magnetic field within the machine. Likewise, the rotor is excited with a DC current (Ie) to act as an electromagnet. In normal operation the rotor magnet follows the stator field at synchronous speed. The rotating electromagnet induces a three-phase voltage (Vg) in the stator windings as if the machine were a synchronous generator. If the machine is considered to be ideal, with no mechanical, magnetic, or electrical losses, its equivalent circuit will be an AC generator in series with the winding inductance (L) of the stator. The magnitude of Vg depends on the excitation current (Ie) and the speed of rotation, and as the latter is fixed, Vg depends only on Ie. If Ie is critically adjusted to a value Ie0, Vg will be equal and opposite to Vs, and the current in the stator (Is) will be zero. This corresponds to the minimum in the curve shown above. If, however, Ie is increased above Ie0, Vg will exceed Vs, and the difference is accounted for by a voltage (Vl) appearing across the stator inductance L: Vl = Is x Xl where Xl is the stator reactance. Now the stator current (Is) is no longer zero. Since the machine is ideal, Vg, Vl and Vs will all be in phase, and Is will be entirely reactive (i.e. in phase quadrature). Viewed from the supply side of the machine's terminals, a negative reactive current will flow out of the terminals, and the machine will therefore appear as a capacitor, the magnitude of whose reactance will fall as Ir increases above Is0. If Ie is adjusted to be less than Ie0, Vs will exceed Vg, and a positive reactive current will flow into the machine. The machine will then appear as an inductor whose reactance falls as Ie is reduced further. These conditions correspond to the two rising arms of the V-curves (above). In a practical machine with losses, the equivalent circuit will contain a resistor in parallel with the terminals to represent mechanical and magnetic losses, and another resistor in series with the generator and L, representing copper losses in the stator. Thus in a practical machine Is will contain a small in-phase component, and will not fall to zero when Ie = Ie0.
Application
An over-excited synchronous motor has a leading power factor. This makes it useful for power factor correction of industrial loads. Both transformers and induction motors draw lagging (magnetising) currents from the line. On light loads, the power drawn by induction motors has a large reactive component and the power factor has a low value. The added current flowing to supply reactive power creates additional losses in the power system. In an industrial plant, synchronous motors can be used to supply some of the reactive power required by induction motors. This improves the plant power factor and reduces the reactive current required from the grid.
A synchronous condenser provides step-less automatic power factor correction with the ability to produce up to 150% additional vars. The system produces no switching transients and is not affected by system electrical harmonics (some harmonics can even be absorbed by synchronous condensers). They will not produce excessive voltage levels and are not susceptible to electrical resonances. Because of the rotating inertia of the synchronous condenser, it can provide limited voltage support during very short power drops .
The use of rotating synchronous condensers was common through the 1950s. They remain an alternative (or a supplement) to capacitors for power factor correction because of problems that have been experienced with harmonics causing capacitor overheating and catastrophic failures. Synchronous condensers are also useful for supporting voltage levels. The reactive power produced by a capacitor bank is in direct proportion to the square of its terminal voltage, and if the system voltage decreases, the capacitors produce less reactive power, when it is most needed, while if the system voltage increases the capacitors produce more reactive power, which exacerbates the problem. In contrast, with a constant field, a synchronous condenser naturally supplies more reactive power to a low voltage and absorbs more reactive power from a high voltage, plus the field can be controlled. This reactive power improves voltage regulation in situations such as when starting large motors, or where power must travel long distances from where it is generated to where it is used, as is the case with power wheeling, the transmission of electric power from one geographic region to another within a set of interconnected electric power systems.
Synchronous condensers may also be referred to as Dynamic Power Factor Correction systems. These machines can prove very effective when advanced controls are utilized. A PLC based controller with PF controller and regulator will allow the system to be set to meet a given power factor or can be set to produce a specified amount of reactive power.
On electric power systems, synchronous condensers can be used to control the voltage on long transmission lines, especially for lines with a relatively high ratio of inductive reactance to resistance.[4]
Gallery
-
Side view of the condenser unit -
Front end of condenser unit -
Blueprint diagram showing interior construction of condenser -
Information plate showing technical specifications of condenser unit
References
- ^ a b B. M. Weedy, Electric Power Systems Second Edition, John Wiley and Sons, London, 1972, ISBN 0-471-92445-8 page 149
- ^ All About Circuits
- ^ http://www.pscpower.com/site/wp-content/uploads/2013/06/Power-Factor.pdf
- ^ Donald Fink, Wayne Beaty (ed) Standard Handbook for Electrical Engineers Eleventh Edition, Mc Graw Hill, 1978, ISBN 0-07-020974-X ,page 14-33