User:CraigNGC/subpage
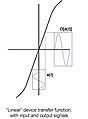
The concept of third-order intercept point (TOI) relates directly to the device transfer function shown in the accompanying diagram.
This transfer function relates the output signal voltage level to the input signal voltage level. We assume a “linear” device having a transfer function whose small signal form may be expressed in terms of a power series containing only odd terms, making the transfer function an odd function of input signal voltage, i.e., O[-s(t)] = -O[s(t)]. Since the signals passing through the actual device will typically be modulated sinusoidal voltage waveforms, device nonlinearities can be expressed in terms of how they affect individual sinusoidal component signals. For example, say the input voltage signal has the form:
s(t) = V cos ωt
And the device transfer function has the form:
O[s(t)] = A1 s(t) + A3 s3(t) + …(additional odd powers)
We may substitute the first equation into the second and, using the trig identity,
cos3x = ¾ cos x + ¼ cos 3x
obtain the device output voltage waveform as:
O[s(t)] = (A1 V + ¾ A3 V3) cos ωt + (A3 V3/4) cos 3ωt .
We note that the output waveform contains the original waveform, cos ωt, plus a new harmonic term, cos 3ωt, the third-order. We also notice that the coefficient of the cos ωt harmonic has two terms, one which varies linearly with V and one which varies with the cube of V (note: the sign of A1 will be positive and the sign of A3 will be negative). In fact, the coefficient of cos ωt has nearly the same form as the original transfer function itself, except for the factor of ¾ on the cubic term. What this says is that as signal level V is increased, the level of the cos ωt term in the output eventually levels off, in similar fashion to the way that the transfer function itself levels off. Of course, the coefficients of the higher-order harmonics will increase (with increasing V) as the coefficient of the cos ωt term levels off, as the power has to go somewhere.
If we now restrict our attention to that portion of the coefficient which varies linearly with V, and then ask ourselves, at what input voltage level, V, will the coefficients of the first and third order terms have equal magnitudes (i.e., where the magnitudes intersect), we find that this happens when
V2 = 4 A1 / |A3 |
which is the Third-Order Intercept Point (TOI). So, we see that the TOI input power level is simply 4 times the ratio of the linear and cubic coefficients in the device transfer function. The smaller the cubic term is in relation to the linear term, the more linear the device is and the higher the TOI is, which clearly makes sense. The TOI, being related to the magnitude squared of the input voltage waveform, is a power quantity, typically measured in milliwatts (mW). The TOI is usually well beyond operational power levels, as the input waveform would be severely distorted at this point.
The TOI is closely related to the amplifier's "1 dB compression point," which is defined as that point at which the total coefficient of the cos ωt term is 1 dB below the linear portion of that coefficient. We can relate the 1 dB compression point to the TOI as follows. First, since 1 dB = 20 log10 1.122, we may say, in a voltage sense, that the 1dB compression point occurs when:
1.122 (A1 V + ¾ A3 V3) = A1 V
or,
V2 = 0.036 * 4 A1 / |A3 |
or,
V2 = 0.036 * TOI.
In a power sense (V2 is a power quantity) a factor of 0.036 corresponds to -14.4 dB, so by this approximate analysis, the 1 dB compression point occurs roughly 14 dB below the TOI.