Quaternion group

Cycle diagram of Q. Each color specifies a series of powers of any element connected to the identity element e = 1. For example, the cycle in red reflects the fact that i² = e, i³ = i and i⁴ = e. The red cycle also reflects that i² = e, i³ = i and i⁴ = e.

In group theory, the quaternion group is a non-abelian group of order eight, isomorphic to a certain eight-element subset of the quaternions under multiplication. It is often denoted by Q or Q₈, and is given by the group presentation

$\mathrm {Q} =\langle {\bar {\mathrm {e} }},\mathrm {i} ,\mathrm {j} ,\mathrm {k} \mid {\bar {\mathrm {e} }}^{2}=\mathrm {e} ,\;\mathrm {i} ^{2}=\mathrm {j} ^{2}=\mathrm {k} ^{2}=\mathrm {ijk} ={\bar {\mathrm {e} }}\rangle ,$

where e is the identity element and e commutes with the other elements of the group.

Compared to dihedral group

The Q₈ group has the same order as the dihedral group D₄, but a different structure, as shown by their Cayley and cycle graphs:

	Q8	Dih4
Cayley graph	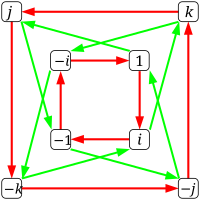Red arrows represent multiplication by i, green arrows by j.
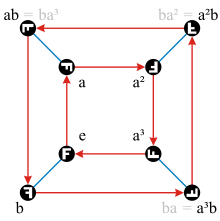
Cycle graph

The dihedral group D₄ arises in the split-quaternions in the same way that Q₈ lies in the quaternions.

Cayley table

The Cayley table (multiplication table) for Q is given by:

×	e	e	i	i	j	j	k	k
e	e	e	i	i	j	j	k	k
e	e	e	i	i	j	j	k	k
i	i	i	e	e	k	k	j	j
i	i	i	e	e	k	k	j	j
j	j	j	k	k	e	e	i	i
j	j	j	k	k	e	e	i	i
k	k	k	j	j	i	i	e	e
k	k	k	j	j	i	i	e	e

Properties

The quaternion group has the unusual property of being Hamiltonian: every subgroup of Q is a normal subgroup, but the group is non-abelian. Every Hamiltonian group contains a copy of Q.

In abstract algebra, one can construct a real four-dimensional vector space as the quotient of the group ring R[Q] by the ideal defined by span_R({e+e, i+i, j+j, k+k}). The result is a skew field called the quaternions. Note that this is not quite the same as the group algebra on Q (which would be eight-dimensional). Conversely, one can start with the quaternions and define the quaternion group as the multiplicative subgroup consisting of the eight elements {1, −1, i, −i, j, −j, k, −k}. The complex four-dimensional vector space on the same basis is called the algebra of biquaternions.

Note that i, j, and k all have order four in Q and any two of them generate the entire group. Another presentation of Q demonstrating this is:

$\langle \mathrm {x} ,\mathrm {y} \mid \mathrm {x} ^{4}=1,\mathrm {x} ^{2}=\mathrm {y} ^{2},\mathrm {y} ^{-1}\mathrm {xy} =\mathrm {x} ^{-1}\rangle .$

One may take, for instance, i = x, j = y and k = xy.

The center and the commutator subgroup of Q is the subgroup {e,e}. The factor group Q/{e,e} is isomorphic to the Klein four-group V. The inner automorphism group of Q is isomorphic to Q modulo its center, and is therefore also isomorphic to the Klein four-group. The full automorphism group of Q is isomorphic to the symmetric group of degree 4, S₄, the symmetric group on four letters. The outer automorphism group of Q is then S₄/V which is isomorphic to S₃.

Matrix representations

Q. g. as a subgroup of SL(2,C)

The quaternion group can be represented as a subgroup of the general linear group GL₂(C). A representation

$\mathrm {Q} =\{\mathrm {e} ,{\bar {\mathrm {e} }},\mathrm {i} ,{\bar {\mathrm {i} }},\mathrm {j} ,{\bar {\mathrm {j} }},\mathrm {k} ,{\bar {\mathrm {k} }}\}\to \mathrm {GL} _{2}(\mathbf {C} )$

is given by

${\begin{matrix}\mathrm {e} \mapsto {\begin{pmatrix}1&0\\0&1\end{pmatrix}}&\mathrm {i} \mapsto {\begin{pmatrix}i&0\\0&-i\end{pmatrix}}&\mathrm {j} \mapsto {\begin{pmatrix}0&1\\-1&0\end{pmatrix}}&\mathrm {k} \mapsto {\begin{pmatrix}0&i\\i&0\end{pmatrix}}\\{\overline {\mathrm {e} }}\mapsto {\begin{pmatrix}-1&0\\0&-1\end{pmatrix}}&{\overline {\mathrm {i} }}\mapsto {\begin{pmatrix}-i&0\\0&i\end{pmatrix}}&{\overline {\mathrm {j} }}\mapsto {\begin{pmatrix}0&-1\\1&0\end{pmatrix}}&{\overline {\mathrm {k} }}\mapsto {\begin{pmatrix}0&-i\\-i&0\end{pmatrix}}\end{matrix}}$

Since all of the above matrices have unit determinant, this is a representation of Q in the special linear group SL₂(C). The standard identities for quaternion multiplication can be verified using the usual laws of matrix multiplication in GL₂(C).

Q. g. as a subgroup of SL(2,3)

There is also an important action of Q on the eight nonzero elements of the 2-dimensional vector space over the finite field F₃. A representation

$\mathrm {Q} =\{\mathrm {e} ,{\bar {\mathrm {e} }},\mathrm {i} ,{\bar {\mathrm {i} }},\mathrm {j} ,{\bar {\mathrm {k} }},{\bar {\mathrm {k} }}\}\to \mathrm {GL} (2,3)$

is given by

${\begin{matrix}\mathrm {e} \mapsto {\begin{pmatrix}1&0\\0&1\end{pmatrix}}&\mathrm {i} \mapsto {\begin{pmatrix}1&1\\1&-1\end{pmatrix}}&\mathrm {j} \mapsto {\begin{pmatrix}-1&1\\1&1\end{pmatrix}}&\mathrm {k} \mapsto {\begin{pmatrix}0&-1\\1&0\end{pmatrix}}\\{\overline {\mathrm {e} }}\mapsto {\begin{pmatrix}-1&0\\0&-1\end{pmatrix}}&{\overline {\mathrm {i} }}\mapsto {\begin{pmatrix}-1&-1\\-1&1\end{pmatrix}}&{\overline {\mathrm {j} }}\mapsto {\begin{pmatrix}1&-1\\-1&-1\end{pmatrix}}&{\overline {\mathrm {k} }}\mapsto {\begin{pmatrix}0&1\\-1&0\end{pmatrix}}\end{matrix}}$

where {−1, 0, 1} are the three elements of F₃. Since all of the above matrices have unit determinant over F₃, this is a representation of Q in the special linear group SL(2, 3). Indeed, the group SL(2, 3) has order 24, and Q is a normal subgroup of SL(2, 3) of index 3.

Galois group

As Richard Dean showed in 1981, the quaternion group can be presented as the Galois group Gal(T/Q) where Q is the field of rational numbers and T is the splitting field, over Q, of the polynomial

$x^{8}-72x^{6}+180x^{4}-144x^{2}+36$.

The development uses the fundamental theorem of Galois theory in specifying four intermediate fields between Q and T and their Galois groups, as well as two theorems on cyclic extension of degree four over a field.

Generalized quaternion group

A group is called a generalized quaternion group when its order is a power of 2 and it is a dicyclic group.

$\langle \mathrm {x} ,\mathrm {y} \mid \mathrm {x} ^{2^{m}}=\mathrm {y} ^{4}=1,\mathrm {x} ^{2^{m-1}}=\mathrm {y} ^{2},\mathrm {y} ^{-1}\mathrm {xy} =\mathrm {x} ^{-1}\rangle .$

It is a part of more general class of dicyclic groups.

Some authors define generalized quaternion group to be the same as dicyclic group.

$\langle \mathrm {x} ,\mathrm {y} \mid \mathrm {x} ^{2n}=\mathrm {y} ^{4}=1,\mathrm {x} ^{n}=\mathrm {y} ^{2},\mathrm {y} ^{-1}\mathrm {xy} =\mathrm {x} ^{-1}\rangle .$

for some integer n ≥ 2. This group is denoted Q_4n and has order 4n. Coxeter labels these dicyclic groups <2,2,n>, being a special case of the binary polyhedral group <l,m,n> and related to the polyhedral groups (p,q,r), and dihedral group (2,2,n). The usual quaternion group corresponds to the case n = 2. The generalized quaternion group can be realized as the subgroup of GL₂(C) generated by

$\left({\begin{array}{cc}\omega _{n}&0\\0&{\overline {\omega }}_{n}\end{array}}\right){\mbox{ and }}\left({\begin{array}{cc}0&-1\\1&0\end{array}}\right)$

where ω_n = e^iπ/n. It can also be realized as the subgroup of unit quaternions generated by x = e^iπ/n and y = j.

The generalized quaternion groups have the property that every abelian subgroup is cyclic. It can be shown that a finite p-group with this property (every abelian subgroup is cyclic) is either cyclic or a generalized quaternion group as defined above. Another characterization is that a finite p-group in which there is a unique subgroup of order p is either cyclic or a 2-group isomorphic to generalized quaternion group. In particular, for a finite field F with odd characteristic, the 2-Sylow subgroup of SL₂(F) is non-abelian and has only one subgroup of order 2, so this 2-Sylow subgroup must be a generalized quaternion group, (Gorenstein 1980, p. 42). Letting p^r be the size of F, where p is prime, the size of the 2-Sylow subgroup of SL₂(F) is 2ⁿ, where n = ord₂(p² − 1) + ord₂(r).

The Brauer–Suzuki theorem shows that groups whose Sylow 2-subgroups are generalized quaternion cannot be simple.

See also

• binary tetrahedral group
• Clifford algebra
• dicyclic group
• Hurwitz integral quaternion
• List of small groups
• 16-cell

References

• Artin, Michael (1991), Algebra, Prentice Hall, ISBN 978-0-13-004763-2
• Brown, Kenneth S. (1982), Cohomology of groups (3 ed.), Springer-Verlag, ISBN 978-0-387-90688-1
• Cartan, Henri; Eilenberg, Samuel (1999), Homological Algebra, Princeton University Press, ISBN 978-0-691-04991-5
• Coxeter, H. S. M. and Moser, W. O. J. (1980). Generators and Relations for Discrete Groups. New York: Springer-Verlag. ISBN 0-387-09212-9.{{cite book}}: CS1 maint: multiple names: authors list (link)
• Dean, Richard A. (1981) "A rational polynomial whose group is the quaternions", American Mathematical Monthly 88:42–5.
• Gorenstein, D. (1980), Finite Groups, New York: Chelsea, ISBN 978-0-8284-0301-6, MR 81b:20002 {{citation}}: Check |mr= value (help)
• Johnson, David L. (1980), Topics in the theory of group presentations, Cambridge University Press, ISBN 978-0-521-23108-4, MR 0695161
• Rotman, Joseph J. (1995), An introduction to the theory of groups (4 ed.), Springer-Verlag, ISBN 978-0-387-94285-8
• P.R. Girard (1984) "The quaternion group and modern physics", European Journal of Physics 5:25–32.
• Hall, Marshall (1999), The theory of groups (2 ed.), AMS Bookstore, ISBN 0-8218-1967-4
• Kurosh, Alexander G. (1979), Theory of Groups, AMS Bookstore, ISBN 0-8284-0107-1

External links

• Weisstein, Eric W. "Quaternion group". MathWorld.
• Quaternion groups on GroupNames