Talk:Coriolis force/archive3

I have archived the Talk:Coriolis effect page, the section 'dynamics of the coriolis effect' has been rewritten. The animated GIF's I have added have finally brought the article into some shape, I think.

The primary source for the coriolis effect article is the writing of the meteorologist/physicist Anders Persson.

The physics involved in the coriolis effect has been well understood for over a hundred years now. However, the physics involved in the coriolis effect is also to this day and age widely misunderstood. (The nature of the misunderstanding is such that it is relativly inconsequential; it has not blocked progress in meteorology. In that sense it is not a case of dissent in the scientific community.) I hope the wikipedia article can be a factor in helping to address the misunderstandings. --Cleon Teunissen | Talk 3 July 2005 07:20 (UTC)

---

Rotating space stations

Could someone write about how Coriolis effect can be used to produce artificial gravity on rotating space stations and how it works there? Kaol 3 July 2005 20:23 (UTC)

Actually, creating artificial gravity by constructing a wheelshaped space-station, and have that space-station rotate is not an option.

I have not performed the calculation myself but I found a source that mentioned that if you have a space wheel with radius of 100 meter then the rotation rate required to get an artificial gravity is one rotation every 20 seconds, and that would result in Coriolis forces that would be almost 4000 times stronger than on the Earth. Machinery with moving or rotating parts, like centrifuges and washing machines might break down. It whould be unconformatable for the crew, for in every motion they make they would experience unsettling coriolis effects.

--Cleon Teunissen | Talk 3 July 2005 22:15 (UTC)

The coriolis effect is just an effect. It cannot be used to produce artificial gravity. And the effect is not relevant directly to the phenomemon of artificial gravity. Spinning the space station creates centripetral forces the same as those which keeps the water in the rotated bucket or the motorcycle against the wall of death. Inside a rotating space station one could think that gravity was pressing you to the wall. "Artificial gravity" so created is, however, not gravity. Paul Beardsell 11:59, 1 August 2005 (UTC)

http://met.no/english/topics/nomek_2005/coriolis.pdf

Whats "erroneous" about this link? William M. Connolley 2005-07-04 19:29:03 (UTC).

I sent an email to Anders Persson, and he responded by sending me two texts but he asked me not to distribute anything because of copyright reasons. One of the texts he sent me was the http://met.no/english/topics/nomek_2005/coriolis.pdf text. I jumped to the assumption that Google had ferreted out something that for copyright reasons wasn't meant to be publicly available. It's not uncommon for Google to dig up information that people mistakenly think is in a directory that won't be Google-indexed.

I removed the link, but it turned out that Anders Persson had made a mistake, and he has confirmed to me that the http://met.no/english/topics/nomek_2005/coriolis.pdf text can be regarded as publicly available.

Anders Persson is currently occupied with other matters that require his full attention.
William, do you have access to Persson's articles in Weather, the magazine of the Royal Metorological Society? --Cleon Teunissen | Talk 4 July 2005 20:34 (UTC)

The two incarnations of 'Coriolis force'

In physics, the expression 'coriolis force' is used in two different contexts, and this difference is rarely appreciated.

(1) One context is the dynamics of objects that are subject to a centripetal force that is proportional to the distance to the center of rotation. Examples: pucks moving over a parabolic surface, as described in the current Coriolis effect article.

(2) The other context is one that is not in our everyday experience: if you are onboard a wheel-shaped rotating space-station, pulling 1 G of artificial gravity at the perimeter, and you toss something up, what will the motion of that object look like?

The clearest exposition of context (2) that I know of is the webpage by Larry Bogan:
External link: Tossing up a ball in a rotating space station

Context (1) and context (2) need to be discussed separately, for they are different subjects. They are different in terms of newtonian dynamics and they are different in terms of the concepts of relativistic dynamics.

I choose to only describe context (1), for that one is what we experience in our everyday lives.

The gif-animations I've made show that the period of the eccentricity of an elliptical orbit is half the period of the complete orbit around the axis of rotation. The counterpart of that in meteorology is called inertial wind.
--Cleon Teunissen | Talk 4 July 2005 21:17 (UTC)

Coriolis flow meter

Engineers refer to the operating principle of mass flow meters as the coriolis effect. They do so because they have context (1) in mind.
External link: The Micro Motion tutorial: operating principle of the curved tube mass flow meter --Cleon Teunissen | Talk 4 July 2005 21:34 (UTC)

Why the 'coriolis effect' is called 'coriolis effect'

There is coriolis dynamics, but there is no such thing as an irreduceble 'Coriolis force'. In that specific sense there is no Coriolis force.

In physics it is quite common to refer to something special with the word 'effect'. Example: the Casimir effect. The Casimir effect is a manifestation of general quantum physics principles, so there is a Casimir effect, but there is no standalone Casimir force, it is a particular behavior that is elicited in that particular configuration. Occasionally the expression 'Casimir force' is used. Again, that does not mean that it is assumed that there is an irreduceble Casimir force, this "Casimir force" is an epiphenomenon, it can and should be accounted for in terms of fundamental principles.

The Casimir effect analogy, featuring ships in a strong swell, is striking. There is an effect, but not a single force.

There is no coriolis force

The coriolis effect gets to have a name of its own because it occurs in certain recognizable circumstances. Like the Casimir effect, the Coriolis effect is an epiphenomenon, it can and should be accounted for in terms of fundamental principles. --Cleon Teunissen | Talk 7 July 2005 08:58 (UTC)

Unlike physics, there are few absolute limits in language. It can be understood that the "Coriolis force" refers to an apparent force resulting from the Coriolis effect. If this wasn't the case, the term wouldn't pop up so often, I think. DavidH 06:06, July 29, 2005 (UTC)

Yes, of course the expression coriolis force is useful physics shorthand, and I support using it as such. Likewise, the expression 'centrifugal force' is useful physics shorthand, as in the name centrifugal governor. Unfortunately the expressions centrifugal force and coriolis force are often taken als literal. --Cleon Teunissen | Talk 07:59, 29 July 2005 (UTC)

The figure skater, the ambassador of the coriolis effect.

The example most often given in presenting the Physics of the Coriolis effect is the figure skater, spinning at a dazzling rate. A google search with the search terms "coriolis" and "figure skater" finds lots of them.

As I described earlier, in the 'two incarnations' posting, there are actually two phenomena, that only have the theme of rotation in common, that are both referred to as "the coriolis effect".

I decided to deal exclusively with the version of 'the coriolis effet' that needs to be taken into account in engineering and meteorology. That is where the action is. --Cleon Teunissen | Talk 18:19, 10 July 2005 (UTC)

Drains and toilets

Everything I've read about Coriolis effect points out that small 'systems' like sink drains and toilets aren't affected, and that the direction of water flow has more to do with the shape of the basin and whatnot. If this is truly the case, then are we to assume that all toilets made in the United States are purposely shaped so the water drains counter-clockwise, while all Australian-made toilets are designed to drain clockwise? It's a conspiracy, I tells ya! --Birdhombre 17:41, 14 July 2005 (UTC)

The edits by 158.147.141.49

The edits by 158.147.141.49 contain a simplification that I think should not be made. I use extensive simplification myself, it is a judgement call wich ones to make.
158.147.141.49 wrote:

The air flow that is initially moving in a direction from South to North is moving closer to the Earth's axis of rotation. The Eastward linear velocity of a point on Earth close to its axis of rotation is less than that of a point close to the equator. As the air flows towards the axis of rotation, its Eastward linear velocity remains constant as the Eastward linear velocity of the point of Earth is it above becomes less and less. This causes the angular velocity of the air flow to increase, and rather than continuing on a straight path towards the center of low pressure, the flow will curve its right, or East as shown in the images to the right.

158.147.141.49 is here invoking conservation of linear velocity. However, that underestimates the coriolis effect.
The first meteorologist to consider the influence of the fact that the Earth is rotating was Hadley, in 1735. Hadley only considered conservation of linear velocity, and thus his theoretical prediction of the strength of the trade winds came out half as strong as in reality, causing doubts about the validity of meteorological theories.

There is in fact a 'double action' which can be seen in the first animation: the weight only moves closer to the axis of rotation if a force is exerted, and that force increases the rotational energy. This is why there is a factor 2 in the formula: :${\vec {F}}_{C}=-2m{\vec {\omega }}\times {\vec {v}}$. It is that double action that is so characteristic of the coriolis effect, and it should be included.

158.147.141.49 as also added:

Cyclones cannot form on, and rarely travel near, the equator because there is little to no Coriolis effect present to sustain them.

That is an important addition. I hadn't written that up yet. --Cleon Teunissen | Talk 20:39, 19 July 2005 (UTC)

Introduction

I'd prefer a slighly longer intro. Some people coming to this article might not have the necessary education to cope with a vector cross product so early in the article. Perhaps a wordy intro would still give them enough. Nice article though. Perhaps it should be put up for featured status? Theresa Knott (a tenth stroke) 11:29, 23 July 2005 (UTC)

Hi Theresa, I agree that the formula - with the vector cross product and all - comes too early in the article. I would prefer starting as simple as possible, and gradually build it up. Mainly, I want to enable people to get a feeling for the physics; if I could I would push people into swivel chairs myself.

The current position of the formula is a bit of a remnant of earlier versions of the article. Some editors feel that the road to understanding starts with the formula, so there was some insistence that the formula should be given first. I hope to get support for moving the formula to a more advanced section of the article.

The main things to bring across, I think, are the swivel chair example, that the coriolis effect in the atmosphere involves mechanical work, and the information that the coriolis effect in the atmosphere doesn't just occur for air flow in North-South or South-North direction, but also for West-East and East-West direction.

And yeah, it would be great if the coriolis effect article would be put up for featured status. I'd like the article to get some exposure --Cleon Teunissen | Talk 16:06, 23 July 2005 (UTC)

---

I'd prefer a slighly longer intro. Theresa Knott (a tenth stroke) 11:29, 23 July 2005 (UTC)

In preparation of requesting for Featured Article status, I have enlarged the intro, and I have moved the formula. Still to do: adding a small section about Taylor columns to the fluid dynamics section. --Cleon Teunissen | Talk 06:48, 25 July 2005 (UTC)

PR comments

I've been avoiding this article for a while, because I disagree with CT over much of the content and structure. But if its up for PR/FA I shall comment:

• Almost all the edits are by CT, and almost all the sections are unsourced, except by the general refs at the end. I find this unsatisfactory.
• In particular the section Imagine a planet with the exact shape of the Earth, but non-rotating (this is in fact physically impossible, the force of gravity contracts a non-rotating celestial body to a near-perfect sphere.) Imagine the planet has no liquid on the surface and the surface is smooth. Imagine you are at the north pole, and you give a frictionless puck a large velocity away from the pole. The puck would soon return to the pole, because in moving away from the pole it is moving uphill. seems dodgy (the corilois force is proportional to the speed, which is not true in this case. So... where did this text come from? Its unsourced. Is it just CTs own example, or what?
• Figureskating is almost always presented as simple conservation of angular momentum.
• Moving the formula down is dubious. I would like to see it right at the start.
• I don't at all understand In the case of the coriolis effects in the atmosphere the cyclonic flow arises because the direction of flow of air masses is strongly affected by the force of gravity. For example: air flowing from East to West is deflected because it is pulled towards the Earth's axis of rotation by the force of gravity.. AFAIK gravity plays no role at all. But this statement, like all others, is unsourced.

William M. Connolley 20:42:24, 2005-07-28 (UTC).

William I want to reply to two of your more minor points.

• The fact that a spinning iceskater is usually explained in terms of conservation of angular momentum is neither hear not there. The only important point is whether it is valid to explain it in terms of conservation of energy as done here.
• You state that "moving the formula down is dubious" but you don't say why. How can anyone adress that concern? Theresa Knott (a tenth stroke) 09:23, 30 July 2005 (UTC)

Replies to William M Connolley

Sources

About sources: if for example an article states that the Earth rotates, and that it is orbiting the Sun, then that particular statement would not rquire specific reference; it is general knowledge, plain for all to see.

It is common practice in physics texts to use thought experiment with a clear outcome. In for example the article about planetary orbitthe image Image:OrbitingCannonBalls.png is shown. The function of that image is to make the reader see the logic, so that the reader does not have to take the article's word for it, the reader can verify for himself that it is correct, by thinking it through.

• If a planet would be perfectly spherical, and it would also rotate, then all the water would flow to the equator, for on the spherical planet the proper centripetal force on the water would not be provided by the planet's gravity.
• Conversely, if a planet would be a ellipsiod, but non-rotating, then all the water would flow to the poles, for the poles would then be point of lowest gravitational potential.

Of course, both situations are strictly hypothetical, for celestial bodies that are rotating around their own center of mass are always in dynamic equilibrium, ellipsoid in shape, such that the water and air is equally thick all over the planet.

However, the ellipsiod shape of the Earth creates an arena for physics taking place that is in simplified form modeled by a turntable with a parabolic surface. An object on a flat turntable would simply fly off. That is centrifugal effect, with just a smithering of Coriolis effect. The parabolic turntable sets up an environment where the centrifugal effect is cancelled, so that what remains is all Coriolis effect.
These are not ex cathedra statements, all of this is pretty elementary physics, readily verified by the reader.
I shall see if I can find appropriate quotes in the work of James F. Price, for reference. --Cleon Teunissen | Talk 05:59, 29 July 2005 (UTC)

The expression Coriolis effect is like the expression Doppler effect

One of the themes of the article is that the expression coriolis effect is to be understood as analogous to expressions like 'Doppler effect' and 'Casimir effect'. There is a Doppler effect, there is no "Doppler force", there is a Casimir effect, there is no "Casimir force". A belief that there actually is a "Coriois force" is a metaphysical belief. --Cleon Teunissen | Talk 05:59, 29 July 2005 (UTC)

The ice skater and keeping track of the causal chain

About the ice skater and conservation of angular momentum. Of course, conservation of ancgular momentum does apply in the case of the ice skater, but conservation of momentum is relatively unsuited for explaining the dynamics of a situation.

For example, when a cannon is fired the projectile is hurled away and the barrel recoils, as there is conservation of momentum. It sounds rather odd to say 'the projectile flies away because the barrel recoils'. That is rather unsatisfactory. A dynamic explanation focuses on what happens to the energy: the gunpowder explodes, chemical potential is converted to heat, expanding gases accelerate the projectile through the barrel. The general practice in physics is to look to the energy and to the forces. Energy is more associated with causality, because energy is associated with how processes develop over time, while momentum is more associated with symmetry of space.

In the Coriolis effect there is conversion of energy from one form to another. Potential-kinetic, kinetic-potential The general physics approach to understanding a phenomenon is to keep track of the energy conversions. --Cleon Teunissen | Talk 05:59, 29 July 2005 (UTC)

---

For example: in my copy of Berkeley Physics course, Mechanics, second edition, chapter 6 "Conservation of linear and angular momentum" there is also a discussion of "Angular acceleration accompanying contraction"

In general, angular momentum is conserved if there is no torque. This has not much explanatory strength, for angular momentum is also conserved if there is no force at all! On this site there is a java applet demo of angular momentum being conserved in uniform velocity; it is shown that Kepler's law of areas is satisfied. --Cleon Teunissen | Talk 06:18, 29 July 2005 (UTC)

The influence of gravity, redirected by the slope

In the article it is stated:

[...]air flowing from East to West is deflected because it is pulled towards the Earth's axis of rotation by the force of gravity.

William M Connolley has written:

AFAIK gravity plays no role at all.

You are misinformed, everything that is resting on the surface of the Earths is affected by gravity the way that is modeled in the parabolic turntable demonstrations.
I shall see if I can find appropriate quotes in the work of James F. Price., for reference. --Cleon Teunissen | Talk 05:59, 29 July 2005 (UTC)

Simply because fact A is true does not mean effect B is caused by it. Paul Beardsell 09:24, 30 July 2005 (UTC)

Unsatisfactory

I find all these responses quite unsatisfactory: you have simply avoided most of my points. The last most obviously displays an apparent ignorance of the physics that I find disturbing, since large parts of this article remain unsourced. I think that far too much of this article represents your personal POV. William M. Connolley 10:27:35, 2005-07-29 (UTC).

Let me repeat a question that you have not answered: In particular the section

Imagine a planet with the exact shape of the Earth, but non-rotating (this is in fact physically impossible, the force of gravity contracts a non-rotating celestial body to a near-perfect sphere.) Imagine the planet has no liquid on the surface and the surface is smooth. Imagine you are at the north pole, and you give a frictionless puck a large velocity away from the pole. The puck would soon return to the pole, because in moving away from the pole it is moving uphill.

I said: this seems dodgy (the corilois force is proportional to the speed, which is not true in this case. So... where did this text come from? Its unsourced. Is it just CTs own example, or what?

So: can you please clarify: do you accept that this example doesn't really work, because the force *isn't* proportional to the speed in the gravity case; and can you please clarify your source for this example: did you invent it, or did you find it in a book; if so, which? William M. Connolley 10:31:54, 2005-07-29 (UTC).

Also, I find

The Coriolis mass flow meter operating principle does not actually involve rotation. It works by inducing a vibration of the tube through which the fluid passes, and subsequently monitoring and analysing the inertial effects that occur in response to the combination of the induced vibration and the mass flow.

dubious. Reading the description of the instrument, it loks rather likely that the vibration of the tube is effectively rotation: back -and-forth rather than all the way round perhaps; but to state definitively (and sourcelessly) that it doesn't involve rot looks wrong. William M. Connolley 11:52:26, 2005-07-29 (UTC).

That can be rephrased. For example: "in: The Coriolis mass flow meter operating principle does not actually involve rotation through whole circle, but moving through part of a circle. (Of course the analogy between rotation and oscillation are well known, motion along an elliptical trajectory can be seen as a superposition of two oscillations, hence the animation in the article.)

Actually, the vibration of a coriolis flow meter is quite a small part of circle: you can't really see the vibration, but you can feel it when you put your finger on the tube. (This was told to me by someone wo works for the company that manufactures coriolis flow meters.)

I will address all your questions William, as soon as I can, but this one is just silly picking on a detail. --Cleon Teunissen | Talk 12:43, 29 July 2005 (UTC)

By the way, where did you read about the Coriolis flow meter? The wikipeida article is written by me.--Cleon Teunissen | Talk 12:46, 29 July 2005 (UTC)

I know that the article was written by you - I checked the history. This question is not nit-picking. The point is that the essential operating principle of the CFM *is* rotation (at least as far as I understand it, and as you seem to be saying above). Your rephrasing would made the article correct, which is good, but it means that the previous text was *wrong*. This, in a nutshell, is the problem I have with the current article: it contains too much of your own personal interpretation. William M. Connolley 14:34:50, 2005-07-29 (UTC).

Here is my reason for pointing out that coriolis flow meters operate by inducing a vibration rather than a complete rotation. It is sometimes suggested that "there is only a coriolis force in a rotating frame of reference". So what does that mean for a coriolis flow meter? When coriolis flow meters are designed, and as they are being used, then the context is always an inertial frame of reference. This shows that as far as the mechanical coriolis effect is concerned, it does not require some special choice of non-inertial frame of reference. It just happens, the coriolis flow meter simply works, without fuss about reference frames. However, I should rather be discussing your main questions, so I will let the coriolis flow meters rest. --Cleon Teunissen | Talk 21:12, 29 July 2005 (UTC)

That is simply an evasion. You need to address the point, not talk round it. And if there is only a coriolis force in a rotating frame of reference is supposed to be a quote from me, it isn't. I do, however, think that you ought to try addressing my other points. William M. Connolley 21:31:40, 2005-07-29 (UTC).

William, you are being evasive.
On this subpage of your User page there is a version of the Coriolis effect artcle that according to you presents correct information.
In this shadow-version of the article you emphasize that the Coriolis effect is to be understood as a change-of-coordinates acceleration. Your shadow-version of the article states:

In changing from one coordinate system rotating relative to another [...], a term appears in the equation of motion described by the formula for Coriolis acceleration:

The operating principle of the Coriolis flow meter does not involve changing from one coordinate system to another. The Coriolis flow meter simply works, no fuss with coordinates systems. You are contradicting yourself. I challenge you to face up to your selfcontradiction.
--Cleon Teunissen | Talk 06:57, 31 July 2005 (UTC)

Don't forget time!

In the bath tub example it is said that the system is small is the reason the coriolis effect is not seen. In the ballistics example the reason given for seeing the effect is that the distance is large. No, it is only partly dimension which is at issue: The other is time. If a very deep bath tub is drained through a small hole over a period of days the coriolis effect will make itself measurable. Similarly with a missile: Don't forget the time! The faster the missile the less the correction required. Paul Beardsell 09:21, 30 July 2005 (UTC)

Reply to the main question of William M Connolley

The goal of the following text is the question: if you have a planet that is non-rotating and that like Earth is an oblate spheroid, and you give a ball a push away from one of the poles, what will happen then, and what is the cause of what happens then. Click here to go straight to that part.

I'm putting it into a larger perspective, showing how it fits in with models of the coriolis effect in meteorology, giving the story in one go rather than drawn-out, fragmented discussion.

One of the problems is Babylonian confusion about the expression 'Coriolis effect'. There are two different phenomena, that are both called Coriolis effect.

Definition

Mechanical Coriolis effect

First a definition, I define as 'mechanical Coriolis effect' the effect that was described in the 1835 paper by Gaspard Gustave Coriolis. It is illustrated in the animation.
This can also be descibed as Angular Acceleration Accompanying Contraction, and of course its inverse: Angular Deceleration Accompanying Relaxation.

Angular Acceleration Accompanying Contraction.

Berkeley Physics Course, second edition, Mechanics, Chapter 6, page 193, discusses Angular Acceleration Accompanying Contraction, and shows that when there is rotation then contraction of the rotational motion requires doing work.

There is conservation of angular momentum, but there is not conservation of kinetic energy, work must be done, by a surplus of centripetal force. The explanation in terms of conservation of angular momentum is less suitable in the field of meteorology, the explanation in terms of energy conversion fits the requirements better, this has been shown by the meteorologist Anders Persson. I shall return to that in a future posting to this Talk page, first the main issues should be adressed.

Motion of a Ball in a Bowl

To prepare for discussing motion on a an oblate spheroid, I will discuss motion of a dry ice puck on a parabolical surface such as is used in the Massachusetts Institute for Technology fluid dynamics education

Non-rotating motion

Harmonic oscillation on parabolic surface

The animation shows a harmonic oscillation (simplified representation, neglecting factors that would slowly build up deviation from simple harmonic oscillation.)
The animation represents the puck going back and forth across the parabolic surface. The restoring force is proportional to the distance to the center of oscillation. The restoring force is doing work all the time. It is doing work when it is accelerating the puck to the center of oscillation, it is doing negative work (conversion of kinetic energy to gravitational potential energy) when the puck is moving away from the center of oscillation.
The restoring force is the force of gravity, redirected by the slope of the parabolical surface.
There is of course no Coriolis force involved.

Orbiting motion

Elliptical orbit
As seen from a non-rotating point of view

The animation shows an elliptical orbit of the puck on the parabolical surface. (The parabolical turntable is rotating now to reduce friction between the puck and the surface). In a real world demonstration the elliptical orbit of the puck on the rotating turntable would, because of friction, slowly be rounded into a concentric circular orbit, just as winds are slowed down by friction over the course of weeks.

Reference: General planetary spreadsheet Excel spreadsheet for calculating orbital dynamics By Micheal Fowler. Department of Physics, University of Virginia.
This spreadsheet allows a wide range of force laws, including a force that is proportional to the distance to the center of attraction, as well as the more familiar inverse square law.
direct link to the Excel orbital motion spreadsheet Try it! Start the proportional force simulation, and see how a difference in starting velocity leads to a difference in eccentricity of the elliptical orbit. It's fun!

An elliptical orbit can be seen as a superposition of two perpendicular harmonic oscillations. The restoring force in the case of the parabolic turntable is the force of gravity, redirected by the slope of the parabolical surface. In all there are two forces here: the force of gravity, and the force exerted by the surface perpendicular to the surface. The resultant force can be decomposed in a vertical component and a (horizontal) centripetal force, maintaining the elliptical orbit of the dry ice puck.

There is no Coriolis force involved.
I repeat, because exactly this is misunderstood by William M Connolley, in the animation 'Elliptical orbit' there is no Coriolis force involved The motion that occurs can be accounted for by assessing the effects of the centripetal force, no further assumption is needed.

The changes in the angular velocity of the puck are related to the mechanical coriolis effect: Angular Acceleration Accompanying Contraction, and its inverse: Angular Deceleration Acompanying Relaxation.

Motion of a Ball on an oblate Spheroid

Non-rotating oblate spheroid

Planet with big plane surface

Planet with big convex bowl

Oblate spheroid planet

On the images the brown ellipse represent a planet, a bit rust-coloured, no water on it, an oblate spheroid in shape and non-rotating (Of course this is strictly hypothetical, for a real celestial body that is non-rotating is drawn to a near-perfect sphere by its self-gravitation.)

The first image shows this planet with on the North pole a large planar surface. Nothing is rotating here, in this section the non-rotating situation is discussed. If a ball is released somewhere on this planar surface, and friction is low enough, it will move towards the point of lowest gravitational potential. If the friction is very low, but not zero, then the ball will oscillate back and forth over the North pole for a while, and will eventually come to rest on the North pole, because that point is closest to the center of gravity.

The second image shows this planet with on the North pole a convex bowl. It is effectively a bowl, because wherever a ball is placed, it will roll towards the pole. The pole is the point of lowest gravitational potential of the bowl, the point closest to the center of gravity.

The third image shows the the planet itself. Each hemisphere is effectively a bowl, because wherever a ball is placed, it will roll towards the nearest pole. The poles are points of of lowest gravitational potential of the bowl, the points closest to the center of gravity.

On a non-rotating oblate spheroid, the motion of a ball that is placed on the surface is influenced only by gravity. (More precisely: the resultant force of the force of gravity and the upwards force from the surface that the ball is resting on.) There is of course no Coriolis force involved. This situation has nothing to do with any Coriolis effect.

When a ball is pushed away from the North pole, then gravity will return it to the North pole. If the friction is very low, but not zero, then the ball will oscillate back and forth over the North pole for a while, and will eventually come to rest on the North pole, the point closest to the center of gravity. The oscillation will not be harmonic oscillation, for the restoring force is not proportional to the distance to the center of oscillation. The closer to the equator the weaker the force towards the pole.

Motion of a Ball, co-rotating on the surface of a Rotating oblate Spheroid

Imagine a planet with the same shape as the Earth, same gravity, same rotation rate, but perfectly smooth. At any latitude, the amount of centripetal force is the amount that is needed for objects at that latitude to remain in circular motion, circumnavigating the planet's axis of rotation.
A ball, laid down at any latitude of the rotating oblate spheroid, at rest with respect to the surface of the rotating oblate spheroid, will stay where it is laid down; at every latitude there is dynamic equilibrium.

On Earth, water does not tend to flow to the poles and it does not tend to flow to the equator because there is dynamic equilibrium at every latitude.

The situation of a rotating turntable with a parabolic surface and the rotating Earth are very similar, at all distances to the axis of rotation there is dynamic equilibrium. Thus, the atmospheric layer is equally thick at every latitude.

Elliptical orbit
As seen from a non-rotating point of view

Elliptical orbit
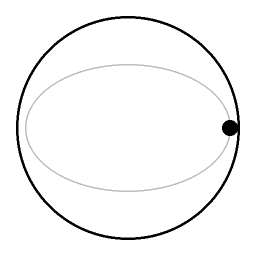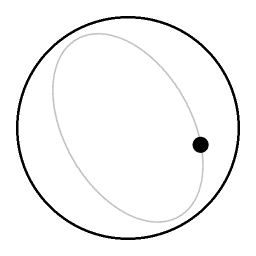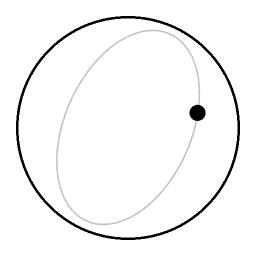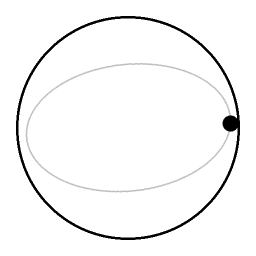
As seen from a co-rotating point of view

On the rotating turntable, there is not only concentric circular motion as a stable orbit, elliptical motion with respect to the turntable's axis of rotation is a stable orbit too. As seen from a co-rotating point of view the eccenticity of the elliptial orbit appears as oscillations with half the period of the overall rotation rate.

In oceanography these oscillations are known to occur widely, and they are called inertial oscillations, and they have a period of 12 hours, or longer, if it is further away from the poles. In meteorology these oscillations are called inertial wind.
Reference for the phenomenon of inertial oscillations: the references of the American Meteorological Society Glossary, the entry about inertial oscillations

The inertial oscillations of ocean water, and inertial wind, can be accounted for by assessing the effects of gravity; the oblate spheroid shape of the planet Earth allows fluids on the surface to follow elliptical orbits around the Earth's axis of rotation. (This is of course a simplified picture, I'm restricting the description to the bare essentials.)

Inertial oscillation of ocean water is started by a period of wind. This starting of an inertial oscillation is a shift from concentric circular orbit around the Earth's axis to elliptical orbit.

Recognizing that the inertial oscillations are in fact the eccentricity of an elliptical orbit is not a new discovery, of course. They are called 'inertial oscillations' exactly because of the analogy with eccentricity in the context of orbital dynamics.

Any questions left?

To answer any additional questions, I need to know exactly what the difficulty is. Please try to explain as clear as possible what exactly you don't understand, so that I can try and figure out a way to explain.

Thorougness

Yes, I wrote a very loooooooooong text again. Of course, if I would have written a terse reply William M Connolley would have said I was being evasive, or shallow, or whatever. There's no pleasing some people.
--Cleon Teunissen | Talk 04:01, 31 July 2005 (UTC)

It's not how long your reply is. It's whether is specifically addresses William's criticism, that matters. Plus you really shouldn't assume what William will say before he says it - (assume good faith and all that). Theresa Knott (a tenth stroke) 13:02, 31 July 2005 (UTC)

You still haven't answered the question!

That was a very long answer that as far as I can see totally misses the point. Let me repeat myself: you said:

Imagine a planet with the exact shape of the Earth, but non-rotating (this is in fact physically impossible, the force of gravity contracts a non-rotating celestial body to a near-perfect sphere.) Imagine the planet has no liquid on the surface and the surface is smooth. Imagine you are at the north pole, and you give a frictionless puck a large velocity away from the pole. The puck would soon return to the pole, because in moving away from the pole it is moving uphill.

I said: this seems dodgy (the coriolis force is proportional to the speed, which is not true in this case). So... where did this text come from? Its unsourced. Is it just CTs own example, or what?

So: can you please clarify: do you accept that this example doesn't really work, because the force *isn't* proportional to the speed in the gravity case; and can you please clarify your source for this example: did you invent it, or did you find it in a book; if so, which?

Please read the question carefully. I'm *not* doubting that a puck on a planet such as you describe would return to the pole: I'm doubting that this has any relevance at all to the coriolis article. The dynamics is simply different.

So: please: here are the questions:

• do you accept that in the ellipsoidal planet example, the dynamics is different, because the force on the puck is *not* proportional to its speed, as it would be on a rotating planet?
• please indicate where this example comes from. I now very strongly suspect that it is one that you have made up yourself. If it isn't, please tell us where it comes from.

Please don't give a long answer. Both these questions require short simple answers.

- William M. Connolley 10:26:13, 2005-07-31 (UTC).

Reply to the main question of William M Connolley - Replaced 'ellipsoid' with the correct 'oblate spheroid'... please: stop fiddling: answer the question instead. William M. Connolley 11:10:01, 2005-07-31 (UTC).

Clarifying: what the coriolis effect tendency is proportional to.

So: can you please clarify: [...] the force *isn't* proportional to the speed in the gravity case;

- William M. Connolley 10:26:13, 2005-07-31 (UTC).

I see where you have overlooked something.

You are becoming distinctly patronising and I am finding that rather irritating. It is becoming quite clear that you really don't understand the coriolis effect at all. William M. Connolley 15:14:58, 2005-07-31 (UTC).

My description of the gravity case is in the context of an inertial frame of reference, with a non-rotating planet. In my description of the gravity case the ball is not circumnavigating the axis of the planet, instead there is oscillation in a straight line. (This gravitational field is symmetrical with respect to the planet's axis, so it transforms without change to a rotating coordinates system)

In the formula for the Coriolis force, the velocity that you insert is the velocity with respect to the rotating coordinate system.

Gosh, is it really?

The formula for the Coriolis force can be used to model the dynamics on a rotating oblate spheriod, no friction, and the ball is on average co-rotating. Now the ball is circumnavigating the planet's axis, so it has a lot of rotational energy

Let's say the ball is on the northern hemisphere, at latitude 45 degrees. If the ball is given a velocity from east to west, then it is moving too slow to maintain latitude, and the force of gravity will pull it towards the pole.

If the ball is given a velocity from west to east, then it is speeding with respect to the Earth. The ball is speeding, so the ball will drift away from the North pole.

If you are driving your car, and you are taking a corner way too fast, then the tires cannot provide the amount of grip that you would need, and you drift outside. The higher your excess speed above the safe speed for that corner, the stronger your drift outside. The same goes for the ball on the planet. The slope is a given, so the amount of centripetal force is a given. The more excess speed of the ball in west to east direction, the stronger the tendency to drift towards the equator.

That is why for air mass moving with respect to the Earth the Coriolis effect tendency is proportional to the velocity with respect to the Earth.

That is sufficient. I won't elaborate about the north-south and south-north directions.
--Cleon Teunissen | Talk 12:20, 31 July 2005 (UTC)

This makes sense to me. Theresa Knott (a tenth stroke) 13:05, 31 July 2005 (UTC)

Theresa, I'd ask you to look more closely at CTs "argument". Basically, its a pile of words that doesn't mean much. The formula for coriolis is 2 * v cross omega. This (time m) is the force, as seen on a rotating planet. But in the case of a frictionless puck on a non-roating planet the formula is entirely different: essentially, its g * slope, directed back along the slope. This force is independent of the motion of the puck. The two formula are completely different.

Hmm it's true I didn't look closely enough.But Cleon isn't saying that the coriolis effect is caused by the non rotating system. Presumably you have to combine the motion independent force with the motion dependent centripetal force. Cleon if your approach is correct then you should be able to derive the formula, yes? Theresa Knott (a tenth stroke) 16:58, 31 July 2005 (UTC)

The problem is one the chasm between two paradigms. I recognize two different contexts, that need to be treated differently. William does not judge my words in terms of my paradigm, he judges them in terms of his own paradigm, in which they obviously don't fit. That is where his "gosh, is it really" comes from. William does not bother to look whether what I say is selfconsistent in terms of my paradigm. William is putting my words in the wrong context, and sure enough, then it doesn't add up.

So providing a derivation would not help, for it goes much deeper. William believes that the coriolis effect is to be understood as merely a coordinate transformation, he states that coordinate transformation does not alter any physics process, and William is also certain that the coriolis effect whips up cyclones in the atmosphere. William's belief system contains tremendoes selfcontradictions. --Cleon Teunissen | Talk 19:13, 31 July 2005 (UTC)

Sigh. CT still doesn't understand whats going on. I'll try to explain. But first, notice that (a) CT still hasn't provided the source for the non-rotating-ellipsoidal-earth thought experiment (its become pretty clear by now that the reason is that its his own pet idea) and (b) he still hasn't acknolwedged that the dynamics in that case is quite different to that of the coriolis effect (c) he has evaded TK's question re formula.

Now - on with the explanation. The Coriolis effect is a change-of-coordinates force (and thus bears intriguing similarities with gravity, whether this means anything or not is unclear). When you move from one coordinate system to one that is rotating with respect to the first, then the equations of motion for a particle acquire an extra term: the coriolis term (and one other of second order in omega but we'll neglect that). So: if you wish to formulate the equations of motion for air on the surface of the earth, it is natural do to it in a coordinate system stationary wrt the earths surface: in that case, you end up with a coriolis force. You could, if you wished, write the equations in a system non-rotating wrt the fixed stars (perhaps ignoring the rotation of the earth round the sun). In this system, there would be no coriolis effect and hence no coriolis force. However there would be a very rapidly moving lower boundary to account for and this would be deeply inconvenient. since the coriolis term is easy to incorporate, there is no particular reason to do this. But... this is only the coordinate system in which you choose to write your equations. It has no effect on the physics, and cyclones continue to rotate no matter which system you describe them in.

The coiolis effect is *not physics*: it is kinematics. William M. Connolley 19:51:33, 2005-07-31 (UTC).

(edit conflict) It would help me. I like to see mathematics, it makes me feel comforatble. Plus it would go a long way towards negating William's "it's all handwaving nonsense" view. Theresa Knott (a tenth stroke) 19:54, 31 July 2005 (UTC)

Coordinate transformation is irrelevant for meteorology

The coiolis effect is *not physics*: it is kinematics. William M. Connolley 19:51:33, 2005-07-31 (UTC).

BTW, note that CT's favourite ref - Persson - supports this: http://www.ap.cityu.edu.hk/Ap8813/References/Coriolis/Coriolis.pdf.

William, this is sheer dishonesty.

• Anders Persson does not see coordinate transformation as relevant at all in discussing meteorology.
• Is is not clear whether Anders Persson has the same thing in mind as you have when he uses the word 'kinematics'. I have tried finding a description of what 'kinematics' can mean. The descriptions are wide apart, there are multple, sometimes vastly different meanings of the word 'kinematics', so it can never be simply assumed that someone else has the same meaning in mind.
• As far as I can tell, Anders persson associates the word 'kinematics' with applying for example conservation laws to perform calculations. If you apply a conservation law then you do not look into the actual physical mechanism, you apply the knowledge that in the end certain quantities must be seen to have been conserved (such as angular momentum) Anders Persson contrasts that with what he refers to as dynamics, where the actual forces are traced. As far as I can tell, Anders Persson uses the word 'kinematics' in a very different way from your use of it.
• Anders Persson states that in meteorology, kinematic approach (in the meaning Anders Persson has in mind) and dynamic approach supplement each other.
• Anders Persson emphasizes that for understanding what physics is taking place kinematics is no good, to understand you must see what force acts when.

If you don't believe me, then by all means you should write an email to Anders Persson. (I'm reluctant to give his email adress here in public, I found his e-adres pretty quickly by googling). I challenge you to put your money where your mouth is. I propose to raise the stakes: if I am mistaken, I will withdraw from the coriolis article, If you are wrong, you will withdraw. Do you accept the challenge? Or do you back away from it? --Cleon Teunissen | Talk 20:29, 1 August 2005 (UTC)

Gravity does not act towards the axis of rotation.

This is wrong:

"In the case of the Coriolis effects in the atmosphere the cyclonic flow arises because the direction of flow of air masses is strongly affected by the force of gravity. For example: air flowing from east to west is deflected because it is pulled towards the Earth's axis of rotation by the force of gravity."

The direction of the force of gravity is to the centre of the earth. In the direction we commonly call "down". The axis of rotation is a line through the centre of the earth and through the north and south poles. An object is not pulled to the axis but to the centre of the earth. Gravity pulls to a point, not to an axis. Paul Beardsell 12:37, 31 July 2005 (UTC)

Air moving initially from east to west (in excess of the speed of rotation of the earth) in the northern hemisphere finds that the land over which it travels seems to turn right relative to the air itself. The air is no longer travelling west but a little south of west. Gravity has nothing to do with it. Paul Beardsell 12:44, 31 July 2005 (UTC)

About the direction of Gravity. If you stand on one of the poles, or on the equator, then then line that is perpendicualr to the local surface goes through the center of mass of the Earth. However, if you stand on, say 45 degrees latitude, then the line perpendicular to the local surface does not go through the Earth's center of mass. This is a geometric property of the ellips, which can be readily verified. It is only on a perfect sphere that on all point of the surface the line perpendicular to the local surface goes through the center of mass. The gravitational field of the Earth is not perfectly spherical. William M Connolley, while disagreeing with me on most matters in this will confirm these geometric properties of the oblate spheroid.

Of course, the deviation from pointing to the center of mass is small, but it provides the centipetal force that is necessary to keep everythig distributed evenly.

It is a bit hard to maintain consistent terminology in this. It is tempting to think in terms of a resultant force that is parallel to the local surface, for that is the plane in wich air mass actually moves. I find myself sometimes writing 'deflected towards the Earth's axis' and sometimes 'deflected towards the north'. It does not lead to ambiguity, I think, but it is not as consistent terminology as I would wish. --Cleon Teunissen | Talk 13:07, 31 July 2005 (UTC)

--Cleon Teunissen | Talk 13:07, 31 July 2005 (UTC)

OK, I acknowledge your point about down not being perpendicular to the surface. I neglected it for good reason. The eccentricity of the ellipse is small and its effect is much smaller than the effect I describe. It creates a false impression to characterise, as the article does, the coriolis effect as observed in the Earth's atmosphere being due mainly to the small eccentricity in the Earth's shape. That massive cyclone photographed is not due to the small eccentricity of the EarthPaul Beardsell 13:24, 31 July 2005 (UTC)

(edit conflict) Yes the force of gravity is towards the center of mass of the Earth. But the point Cleon is making is that for a non spherical Earth, the result of the Force towards the center (plus the vector sum of the normal reaction I suppose) leaves a net force towards the North or South Pole. I suppose it could be worded better though. Theresa Knott (a tenth stroke) 13:13, 31 July 2005 (UTC)

Terminology here is easily improved and so it should be. I am not sure I am the best one to do it. Paul Beardsell 13:24, 31 July 2005 (UTC)

---

There are other examples. Here's one:

"so the air mass will tend to slide away from the Earth's axis of rotation"

What direction is that? The only correct way to read that is "perpendicularly away from the axis" i.e. directly towards the astronomical equatorial plane. If you mean to say towards the pole, say that.

Paul Beardsell 15:25, 31 July 2005 (UTC)

The eccentricity sets up an arena for coriolis effects to occur

That massive cyclone photographed is not due to the small eccentricity of the EarthPaul Beardsell 13:24, 31 July 2005 (UTC)

The small eccentricity of the Earth is most certainly not the cause of any coriolis effect, I don't know where you got that idea.

I know! I never said that. It is being said here. And it is wrong! Paul Beardsell 14:48, 31 July 2005 (UTC)

The only thing that the eccentricity of the Earth does is that at every latitude the centrifugal tendency is canceled. With centrifugal effects out of the way, there is opportunity for coriolis effects.

No, if you are going to assume the (impossible) perfect sphere of the rotating earth I am then going to assume enough water / air to completely cover the surface. Then we will have cyclones. Due to the coriolis effect. The eccentricity of the earth's shape is caused by its rotation. As is the coriolis effect. The coriolis effect does not cause the eccentricity. Neither is the cause vice versa. Both are caused by the rotation. Paul Beardsell 14:48, 31 July 2005 (UTC)

If the Earth would be perfectly spherical, and rotating, then all the water and air would flow towards the equator, so that would be a situation of total dominance of centrifugal tendency, with no opportunity for coriolis effects.

I would say: "If the Earth would be perfectly spherical, and rotating, then all the water and air would flow towards the equator" to such an extent that the current geoid shape would be reconstructed. −Woodstone 14:21, July 31, 2005 (UTC)

Yes! Paul Beardsell 14:48, 31 July 2005 (UTC)

The eccentricity is not the cause, but is is vital in setting up an arene for coriolis effects to occur.

That is NOT TRUE. Both the eccentricity and the coriolis effect are caused by the rotation. Neither is the cause fo the other. Paul Beardsell 14:48, 31 July 2005 (UTC)

Compare a flat turntable and a parabolical turntable. Put a ball on a rotating flat turntable, it will immediately fly off. That is centrifugal effect, with just a smithering of coriolis effect. Make yourself a parabolical turntable, and then the centrifugal effect is cancelled, allowing the much weaker coriolis effect to take the center of the stage. --Cleon Teunissen | Talk 13:42, 31 July 2005 (UTC)

That fact A is true does not mean it causes effect B. Paul Beardsell 14:48, 31 July 2005 (UTC)

---

The above discussed subtleties are not even worth considering while the article says this:

"In the case of the Coriolis effects in the atmosphere the cyclonic flow arises because the direction of flow of air masses is strongly affected by the force of gravity. For example: air flowing from east to west is deflected because it is pulled towards the Earth's axis of rotation by the force of gravity."

I refer you to the first sentence. That is not correct. And if the earth's surface were a perfect sphere (this impossibility is being used in thought experiments elsewhere here) then the atmosphere would STILL exhibit cyclonic effects. Due to the coriolis effect. All the rest is interesting but peripheral detail.

Paul Beardsell 14:04, 31 July 2005 (UTC)

Well, in the hypothetical case of perfectly spherical rotating Earth, there would hardly be atmosphere for coriolis effects to occur in, all the water and air would gather in a ring around the equator, and the right gradient of wouldn't be there.

If you assume perfect sphere I will assume enough air to cover the surface. The depth of the air will vary because of rotation. There will be coriolis effects becuase of rotation. Eccentricity neither causes nor is caused by coriolis effect. Both are caused by the rotation. Paul Beardsell 14:48, 31 July 2005 (UTC)

About that first sentence, 'being strongly affected by the force of gravity'. An important factor is that there is behavior in the atmosphere that is analogous to Taylor columns in fluids. That has to do with the non-uniformity of the centripetal force, just as the force in a fluid towards the center of rotation is non-uniform.

I'm not interested in a complicated analogy which complicates unnecessarily. It is obfuscation. Paul Beardsell 14:48, 31 July 2005 (UTC)

But it should be rephrased, yes, for now it allows the incorrect assumption that the sentence refers to the component of the gravity towards the center of the Earth (which is the largest component) --Cleon Teunissen | Talk 14:32, 31 July 2005 (UTC)

Rephrased? Removed more likely! Paul Beardsell 14:48, 31 July 2005 (UTC)

Coriolis effects on a perfect sphere

If(!) the earth were a perfect sphere and(!) it had the same qty of air and water then there would still be coriolis effects. In the band of air and water about the equator. Point proven. Let's move on. Paul Beardsell 14:48, 31 July 2005 (UTC)

Well, I don't think it's provable. The fluid parts would distribute in such a way that there would be, in a limited area/volume something identical to an oblate spheroid. And of, course, on an oblate spheriod there is an arena for coriolis effects. Nah, it's too hypothetical anyway to really have physical meaning, I think. --Cleon Teunissen | Talk 15:02, 31 July 2005 (UTC)

It's called a thought experiment, Cleon. If(!) the Earth were a regular cube and(!) it had the same qty of air and water then there would still be coriolis effects. In the six pools at the centre of the faces. The geoid shape of the Earth and the coriolis effect are caused by the Earth's rotation. Neither causes the other. Paul Beardsell 15:14, 31 July 2005 (UTC)

Editing

Having finally lost patience with endless talk leading nowhere, I've started editing the article. William M. Connolley 15:22:03, 2005-07-31 (UTC).

There was so much dodgy stuff in this article. CT has been let loose on this for far too long without anyone checking. He never ever engages with the mathematics but always does handwaving "explanations" that clarify nothing. William M. Connolley 15:37:20, 2005-07-31 (UTC).

William M Connolley is contradicting himself

I am copying this from a section higher up on this same page.--Cleon Teunissen | Talk 20:56, 31 July 2005 (UTC)

William M. Connolley 19:51:33, 2005-07-31 (UTC). Now - on with the explanation. The Coriolis effect is a change-of-coordinates force (and thus bears intriguing similarities with gravity, whether this means anything or not is unclear). When you move from one coordinate system to one that is rotating with respect to the first, then the equations of motion for a particle acquire an extra term: the coriolis term (and one other of second order in omega but we'll neglect that). So: if you wish to formulate the equations of motion for air on the surface of the earth, it is natural do to it in a coordinate system stationary wrt the earths surface: in that case, you end up with a coriolis force. You could, if you wished, write the equations in a system non-rotating wrt the fixed stars (perhaps ignoring the rotation of the earth round the sun). In this system, there would be no coriolis effect and hence no coriolis force. However there would be a very rapidly moving lower boundary to account for and this would be deeply inconvenient. since the coriolis term is easy to incorporate, there is no particular reason to do this. But... this is only the coordinate system in which you choose to write your equations. It has no effect on the physics, and cyclones continue to rotate no matter which system you describe them in.

The coriolis effect is *not physics*: it is kinematics. William M. Connolley 19:51:33, 2005-07-31 (UTC). [end of copied section]

William M Connolley is simultaneously saying that the coriolis effect is physics, and that it isn't.

An article about the coriolis effect should explain the following:. If (hypotherically speaking) a low pressure area would form on a non-rotating planet, then the air would flow towards the low pressure area and the pressure gradient would very rapidly be leveled.

But that is not what is happening on Earth. On Earth, air starts flowing around a low pressure area, amazingly, it tends strongly to flow perpendigular to the pressure gradient, and it can take weeks for a low pressure area to become leveled again So there must be a physical mechanism that performs a restructuring of the direction of wind flow.

A general coordinate transform transforms the entire picture in one go, if you employ a coordinate transformaton to a rotating coordinate system on, say, a large grid, then the grid will turns around as a whole, with the whole picture remaining in a fixed form.

That is not what happens in the atmosphere! If you let go lots of weather balloons in the area of a low pressure area forming, then over time the positions of the balloons in the circling winds will be thoroughly mixed. The more central parts of the system rotate faster.

William says that the coriolis effect is to be understood as purely a cosmetic change: a coordinate transformation, that does not alter the physics taking place.

But Willam also believes the following: from: http://en.wikipedia.org/wiki/User:William_M._Connolley/Coriolis_effect

The Coriolis force plays a strong role in weather patterns, where it affects prevailing winds and the rotation of storms, as well as in the direction of ocean currents. Above the atmospheric boundary layer, friction plays a relatively minor role, as air parcels move mostly parallel to each other. Here, an approximate balance between pressure gradient force and Coriolis force exists, causing the geostrophic wind, which is the wind effected by these two forces only, to blow along isobars (along lines of constant geopotential height, to be precise). Thus a northern hemispheric low pressure system rotates in a counterclockwise direction, while northern hemispheric high pressure systems or cyclones on the southern hemisphere rotate in a clockwise manner, as described by Buys-Ballot's law.

This is why I recognize that there are two contexts, that both called 'coriolis effect'. One context is about physical mechanisms, the other is about coordinate transformation.
--Cleon Teunissen | Talk 20:56, 31 July 2005 (UTC)

CT, alas, is demonstrating how much he doesnt understand what is going on. There is no contradiction in my words, only in his understanding of them. My wife (who is reading this but sadly never comments) suggests that he is having problems with the concept of change-of-coordinates. Reading CT's William says that the coriolis effect is to be understood as purely a cosmetic change: a coordinate transformation, that does not alter the physics taking place. makes me think so. I agree with the sentence, but with purely a cosmetic change deleted. I never said that, and I wouldn't.

Of course, the physics on a rotating planet is different from the atmospheric physics on a non-rotating planet. But the physics (understood as the relative motion of parcels of air) on a rotating planet is the *same* whether you view that from the rotating system itself, or from outside the rotating system.

Let me try (for those with sufficient maths/physics) to grind through it explicitly:

In frame FR1 (inertial wrt the fixed stars, let us say) the equations of motion are (1) F1 = m * a1, where a1 is the acceleration measured in the frame FR1. F1 is then (slightly depending on how you view the equations of motion) defined by (1) and m and a1; or (if you think you know the various forces making up F1... electrostatic, gravitational, whatever) an assertion of identity between the forces and m * a1.

Then, basic kinematics (with no physics involved) states that in a second frame FR2, rotating with angular velocity w wrt FR1, the equations of motion are (2) F1 + m*2*w cross v2 + m*w cross (w cross x2) = m * a2, where a2 is the acceleration in FR2 and v2 is the velocity in FR2 and x2 the position in FR2 (plus or minus any sign errors I may have made).

If you're confused by the sudden appearence of w x (w x x2) then ignore it: its second order in w so small for the earth. It can be, and usually is, subsumed into the gravitational potential anyway and effectively disappears.

Happily this is all newtonian not relatavistic so we don't worry about m varying.

The term 2*w cross v2 is the coriolis acceleration.

Coming back to the physics of what happens on earth: you can, at your pleasure, use coordinate system FR1 or FR2. If you use FR2 you get a coriolis force term in the equations. If you use FR1 you don't, but you do get other hideous complications.

All clear now? William M. Connolley 21:33:43, 2005-07-31 (UTC).

What is the physical mechanism?

William, you have avoided the main question:
I will repeat it:

An article about the coriolis effect should explain the following:. If (hypotherically speaking) a low pressure area would form on a non-rotating planet, then the air would flow towards the low pressure area and the pressure gradient would very rapidly be leveled.

But that is not what is happening on Earth. On Earth, air starts flowing around a low pressure area, amazingly, it tends strongly to flow perpendigular to the pressure gradient, and it can take weeks for a low pressure area to become leveled again So there must be a physical mechanism that performs a restructuring of the direction of wind flow.

A general coordinate transform transforms the entire picture in one go, if you employ a coordinate transformaton to a rotating coordinate system on, say, a large grid, then the grid will turns around as a whole, with the whole picture remaining in a fixed form.

That is not what happens in the atmosphere! If you let go lots of weather balloons in the area of a low pressure area forming, then over time the positions of the balloons in the circling winds will be thoroughly mixed. The more central parts of the system rotate faster.

You fail to explain the restructuring, which is the real question.
--Cleon Teunissen | Talk 21:46, 31 July 2005 (UTC)

If you transform to a rotating coordinate system, the whole picture will stay the same, but the equations of motion change. The physics stay the same, but the formulas look different. The Coriolis force appearing in the equation is just a way to explain the physics. On a non-rotating planet, evidently, the Coriolis term in the equation would be zero. I brought back a few lines in the main article to address the effect without rotating coordinates. −Woodstone 22:02, July 31, 2005 (UTC)

Looking for an explanation

The Coriolis force appearing in the equation is just a way to explain the physics.Woodstone 22:02, July 31, 2005 (UTC)

You cannot be serious: it has been emphasized that a coordinate transformation does not alter the physics taking place (obviously). A coordinate transformation has no explanatory power. A coordinate transformation does not reveal something that you didn't know before.

The word explain says it nicely: to explain is to unfold, to reveal what was hidden. Only when your understanding is moved to a deeper level is it an explanation. --Cleon Teunissen | Talk 22:27, 31 July 2005 (UTC)

Consider the two animated gifs you made:

• Elliptical orbit As seen from a non-rotating point of view
• Elliptical orbit As seen from a co-rotating point of view

which are reconstructions of the MIT lab films. They are NOTHING BUT demonstrations of the coordinate transformation. And yet you must find it explains something ("to explain is to unfold, to reveal what was hidden...") , because you have copied them to several places. It "does not alter the physics taking place" (your words), yet it has great "explanatory power", it DOES "reveal something that you didn't know before". THe cyclones of weather are like "Elliptical orbit As seen from a co-rotating point of view". Your cognitive task is to imagine the cyclones from the other , nonrotating point of view. GangofOne 10:27, 1 August 2005 (UTC)

References

William M Connolley has suggested that I did not provide refernces because the ideas presente were inventions of my own inventions. Here are references, I have been reporting published work.

My main sources of information have been the articles by the meteorogist Anders Persson. Anders Persson is a widely published meteorologist. In 2000 the Royal Meteorological Society published a series of three articles about the coriolis effect. The article-titles of the issues of the year 2000

This article by Anders Persson, (PDF-file, 826 KB) On page 10 Anders Persson writes.
"For a stationary object on a rotating planet or a rotating parabolic surface the horizontal components of the centrifugal force is balanced by the horizontal component of the gravitational force of the planet and, on the turntable, the orizontal component of the weight of the body. In both cases the component of gravitation and gravity, perpendicular to the rotational axis, equals the centrifugal force."
[end quote]

This article by Anders Persson (PDF-file, 374 KB), published in the Bulletin of the American Meteorological Society describes the mechanism for the deflection of eastward movement and for westward movement:
Anders Persson writes:
Due to its ellipsoid shape, the gravitational forces balance the centrifugal force on any body, as long as it does not move When it moves, the balance is altered. For an eastward movement the centrifugal force is increased and the body is deflected toward the equator, to the right of the movement. For a westward movement the centrifugal force is weakened and can no longer balance the gravitational force, which is the physical force that moves the body in the poleward direction to the right of the movement (Durran 1993; Durran and Domonkos 1996).

Durran, D. R., 1993: Is the Coriolis force really responsible for the inertial oscillation? Bull. Amer. Meteor. Soc., 74, 2179– 2184; Corrigenda. Bull. Amer. Meteor. Soc., 75, 261
——, and S. K. Domonkos, 1996: An apparatus for demonstrating the inertial oscillation. Bull. Amer. Meteor. Soc., 77, 557–559.

Massachusetts instutute of technology
Physics of Atmospheres and Oceans
Inertial circles - visualizing the Coriolis force: GFD VI By John Marshall Inertial circles - visualizing the Coriolis force: GFD VI
Professor John Marshall, Dept. of Earth, Atmospheric, and Planetary Sciences has included experimenting with ar rotating parabolical turntable in the atmospheric sciences education, to provide understanding of what is taking place.
Professor John Marshall writes:
The circular trajectories – which are called ‘inertial circles’ – are commonly observed in the atmosphere and ocean.

Dr. D.I. Benn of the University of Saint Andrews in Scotland writes: Source of the quote from Dr D. I. Benn
Of all aspects of meteorology, the Coriolis effect is perhaps the most misunderstood, and generations of students have struggled with it. This is not because it is any harder than other aspects, but mainly because the explanations in many textbooks are misleading, if not downright wrong. For example, some books attempt to explain the Coriolis 'force' in terms of balls rolling over rotating turntables or similar analogies, but such explanations do not help, as the physics involved is completely different.
[...]
The best discussion of the Coriolis effect is in three recent articles by Anders Persson in the journal Weather . Understanding these still requires focused thought, but at least the explanations make sense (so it is a lot easier than trying to understand something that doesn't make sense!). The following discussion follows Persson. Ignore the stuff about rotating turntables and wandering polar bears in the textbooks. An excellent discussion (incorporating Persson's ideas) is given by Stull' in Meteorology for Scientists and Engineers. [end quote]

This concludes for now the list of my references.
--Cleon Teunissen | Talk 08:05, 1 August 2005 (UTC)

---

I repeat a paragraph you quote above: "Of all aspects of meteorology, the Coriolis effect is perhaps the most misunderstood, and generations of students have struggled with it. This is not because it is any harder than other aspects, but mainly because the explanations in many textbooks are misleading, if not downright wrong. For example, some books attempt to explain the Coriolis 'force' in terms of balls rolling over rotating turntables or similar analogies, but such explanations do not help, as the physics involved is completely different." But you have spent many paragraphs doing just this! You push pucks away from the pole of a stationary Earth. Countless examples. As it happens I disagree with this quote as it is possible to demonstrate the coriolis effect using solids if you have carefully crafted surfaces to cancel out the effects of stronger forces on the solids involved. But Benn disagrees with your approach, yet you quote him. Paul Beardsell 10:11, 1 August 2005 (UTC)

If one wants to construct a mechanical version of the coriolis effect without using fluids then what you described elsewhere on the talk page and what you quote may be good ways of doing this. But that does not support your assertion that the Earth must be a certain shape for the coriolis effect in the atmosphere to be noticeable. When one has fluids instead of balls or pucks the carefully crafted surface is no longer required as all is floating within the fluid. Any one cubic meter (or molecule or whatever) of air within the atmosphere is unaffected by the shape of the surface of the Earth far beneath it. Yet, the shape of the underlying earth is crucial according to you and you rely upon it in your descriptions of the coriolis effect in the atmosphere above it. Wrong. (It is as though you tried to persuade us the shape of the surface of a liquid depended upon the shape of the bottom of its container.) That you make this claim makes me examine anything else you write very sceptically. Paul Beardsell 10:11, 1 August 2005 (UTC)

A challenge to face up to selfcontradictions

Of course, the physics on a rotating planet is different from the atmospheric physics on a non-rotating planet. But the physics (understood as the relative motion of parcels of air) on a rotating planet is the *same* whether you view that from the rotating system itself, or from outside the rotating system. William M. Connolley 21:33:43, 2005-07-31 (UTC).

So, what is the difference between the physical mechanisms on a rotating planet and the physical mechanisms on a non-rotating planet?

I checked the what links here page. There are over 50 links to the coriolis effect article, many about meteorological articles, and they link to the coriolis effect article in the expectation that the coriolis effect article wil explain the physical mechanisms that are involved.

William, in your opinion the expression 'Coriolis effect' should be associated exclusively with coordinate transformation. Coordinate transformation is unrelated to the physical mechanism of what is taking place, the coordinate transformation is part of the mathematical toolbox, like a computer is part of the toolbox of meteorologists.

Following your logic, the article about the 'Coriolis effect' should be about coordinate transformation only, without going into any physics.

{User:Woodstone has added the remark:

Note that the Coriolis force is an apparent force, experienced in a rotating frame of coordinates.

Why would you want to write about meteorology in an article that is only about coordinate transformation?
Why would you wnat to write about the Coriolis flow meters in an article that is only about coordinate transformation?
You do write about meteorology, and you name 'coriolis force' as a factor that is physically influencing the direction of the wind.

You are contradicting yourself, and you are not facing up to it.
--Cleon Teunissen | Talk 09:48, 1 August 2005 (UTC)

Confusing para in Ballistics section

A paragraph in the Ballistics section reads:

"The fact that the rotation of the Earth needs to be taken into account in ballistics is commonly referred to as an example of the Coriolis effect. It should be noted that it is in fact not the same as the mechanics of the animations in the Mechanics section at the start of the article."

I cannot parse the second sentence. What does the 2nd "it" refer to? Paul Beardsell 10:33, 1 August 2005 (UTC)

"It" probably refers to 'the fact that the rotation of the Earth needs to be taken into account in ballistics'. I guess this is a good time to mention that the Coriolis effect in Mechanics section isn't about the Coriolis effect, as I understand it. The author seems to think there are 2 coriolis effects, or something, but I think not. (Edit conflict; the below appeared as I was trying to post this comment. )GangofOne 10:45, 1 August 2005 (UTC)

What Coriolis described in his 1835 paper is a mechanical effect, that is most often illustrated with the example of an ice-skater spinning faster and faster as she pulls in her arms. Try a google search with the combination "Ice skater" and "coriolis"

What the paragraph says is that what is happening in that animation, a ball being thrown over, is in terms of physical mechanism unrelated to the animations in the section with the weights sliding in and out. They're both called coriolis effect, and I wrote that they are different physics.

So the sentence is meant to say:
It should be noted that the 'coriolis effect' as it is taken int account in ballistics is in fact not the same as the mechanics of the animations in the Mechanics section at the start of the article.

--Cleon Teunissen | Talk 10:59, 1 August 2005 (UTC)

There is only one coriolis effect. Same physics. Paul Beardsell 12:27, 1 August 2005 (UTC)

CT is wrong. There is only one coriolis effect. There are two ways of thinking about it and he is mixing up the ways-of-thinking with the effect. William M. Connolley 12:34:30, 2005-08-01 (UTC).

Above, CT also said: "The figure skater, the ambassador of the coriolis effect. The example most often given in presenting the Physics of the Coriolis effect is the figure skater, spinning at a dazzling rate. A google search with the search terms "coriolis" and "figure skater" finds lots of them. As I described earlier, in the 'two incarnations' posting, there are actually two phenomena, that only have the theme of rotation in common, that are both referred to as "the coriolis effect". ... --Cleon Teunissen | Talk 18:19, 10 July 2005 (UTC)

I have taken up the challenge: "A google search with the search terms "coriolis" and "figure skater"" After actually reading many of the offerings, although all describe the figure skater--angular momentum converver, almost all DO NOT call that the Coriolis effect. The Coriolis effect is something else. Although Coriolis described the a.m. conservation in his paper, I'm sure. What is called "mechanical Coriolis effect" in the article with the rotating weights moving in and out, is not illustrating the Coriolis effect. I did find 2 examples via google of papers that seemed to say the figure skater illustrates the Coriolis effect, one was on helicopter lingo for laymen, which I discount, the other a physics-type lecture, but I think it is WRONG. Even A. Perrson says there are many wrong explanations. So I found one. Even A. Persson doesn't say the figure skater is examplifying Coriolis. Prove me wrong by pointing to some specfic text if you can. GangofOne 09:51, 2 August 2005 (UTC)

Actually, the combination of "ice skater" and "coriolis" gets more Google hits. I have just tried the following combination: "angular momentum" "conservation" and "coriolis" and I got 12.100 Google hits.

It seems to me that in different branches of science and engineering things have moved in different directions, and there just isn't a unity. Gaspard Gustave Coriolis evaluated purely the forces and the conversions of energy: how much force does it take and what will be the gain/loss of rotational energy? I'm not passing judgement, I will not say 'this is coriolis, and that isn't, I just try to be comprehensive. --Cleon Teunissen | Talk 10:32, 2 August 2005 (UTC)

Try actually READING the google hits. It is only natural that a page mentioning Coriolis effect would also discuss skater/a.m. but that doesn't mean the page in question is equating the two. Just that they are discussed on the same page. Find some google hits that say otherwise, if you can. The only reason it's an issue is this stuff we're writing is supposed to be a correct source of terminology, so I just trying to pin it down. GangofOne 10:53, 2 August 2005 (UTC)

OK, angular momentum conservation and coordinate transformation are very likely to be discussed in the same text. So the amount of Google hits is not informative. I must say, I had underestimated the magnitude of the shift in focus. Well, there is no arguing with sheer numbers. There has been a shift, and the original meaning of coriolis effect is now only used by a minority. Right or wrong, that is what has happened. I would prefer to present the full historical development, in order to address confusion. --Cleon Teunissen | Talk 11:27, 2 August 2005 (UTC)

Two coriolis effects?

Cleon asserts there are two different coriolis effects. I would appreciate a reference for this assertion. Paul Beardsell 10:40, 1 August 2005 (UTC)

The version of the coriolis effect I first learned was the spinning ice-skater version. I do not have a statement somewhere that I can quote, but some google searching will establish that the mechanics of an ice-skater spinning up is commonly referred to as 'coriolis effect' and we have that the term arising in a coordinate transformaton is referred to as 'coriolis effect' also. The difference is that in the mechanical coriolis effect there is a constant centripetal force, and the ball being thrown over is not being influenced by a force (not during its flight) --Cleon Teunissen | Talk 11:09, 1 August 2005 (UTC)

Cleon asserts there are two different coriolis effects. I would appreciate a reference for this assertion. Paul Beardsell 11:25, 1 August 2005 (UTC)

(pasted into this section in attempt to keep the various issues distinct and manageable:)

This is a bit of a problem. There are two phenomena, only superficially related, that are both commonly referred to as 'coriolis effect'. One is what happens when an ice-skater pulls in her arms, the other is that in ballistics the fact that the Earth rotates is taken into account. I register that. I will not pass judgement, ruling one to be "the real effect" and the other not. I will not say: that is an effect, and that isn't. But I cannot gloss over it.

I'm not saying there are two coriolis effects, I am more cautious than that. I say two phenomena, only superficially related, different physics. --Cleon Teunissen | Talk 12:03, 1 August 2005 (UTC)

I cannot see the problem. I cannot see two different coriolis effects. Are the "phenomena" real or are they effects? What is the meaning of the word phenomenon you would like to use? If you now think there is only one effect which manifests itself in two different ways then OK (but this differs from your position formerly) but I still don't see them. Fair enough! Persuade me, let's have the two different maths for the two different manifestations. Or, better still, a reference. Thanks. Paul Beardsell 12:14, 1 August 2005 (UTC)

I will call the example of the ice-skater spinning up 'Angular acceleration' (short for Angular Acceleration Accompanying Contraction) She needs to do work to pull in her arms, and her rotational energy increases. So, lots of dynamics taking place, and there is energy conversion. Rotational energy is measurable, you can measure the amount of energy stored in the spinning of a flywheel. Spinning up a flywheel is like charging up a battery, you can charge it up, you can deplete it to zero. In that sense, rotational energy resembles potential chemical energy.

By contrast: in ballistics, in the idealized situation of no friction, then the projectile just moves inertially. It's not rotating, no work is being done, no conversion of energy. It's just plain inertial motion, and the projectile does not have a particular kinetic energy, since kinetic energy is relative. --Cleon Teunissen | Talk 12:28, 1 August 2005 (UTC)

---

The coriolis effect is made apparent by the relative motion of a rotating and non-rotating body. The surface of the earth moves at different speeds at different latitudes, the ballistic missile trajectory appears to bend relative to the earth. It does not actually bend, of course. Hence the use of the word "effect". The coriolis "force" is the force that would be needed to bend the trajectory if the Earth were not rotating. But it is rotating so there is no force. And it can all be explained without ever invoking the coriolis effect, if you wish. It is just an effect. Not real. But at least it is apparent. Paul Beardsell 12:55, 1 August 2005 (UTC)

Where is the coriolis effect apparent with the skater? It is all explained much more simply by using the real forces involved. Where is the non-rotating body? What can be explained by to either the spectator or to the skater by invoking the coriolis effect? As the arms are withdrawn the hands are slowed down (linearly) by the application of a force the opposite of which increases the angular velocity. The coriolis effect, I suppose, is that which resists the pulling in of the arms along a radius - it tends to wind the thread of the arms around the spool of the body. Paul Beardsell 12:55, 1 August 2005 (UTC)

The difference between the ballistics and the skater is that in the ballistics no sideways force is actually applied: The missile travels in a line. A force equivalent to the coriolis force is applied on the hands by the skater to prevent her hands travelling in a real straight line when they are withdrawn so that they are withdrawn in a straight line relative to her point of view. In ballistics the apparent sideways force is not (can not!) be resisted. With the skater it is. It's otherwise all the same. Ballistics and skater. And winds! Paul Beardsell 12:55, 1 August 2005 (UTC)

Cleon, if this does not persuade you then I think you are lost: I really think you miss something fundamental. Paul Beardsell 12:55, 1 August 2005 (UTC)

Inertial circles

What's the issue here? Cleon provides a quote asserting that inertial circles exist. Who says differently? Paul Beardsell 10:44, 1 August 2005 (UTC)

The shape of the Earth's gravitational field

But that does not support your assertion that the Earth must be a certain shape for the coriolis effect in the atmosphere to be noticeable. Paul Beardsell 10:11, 1 August 2005 (UTC)

There is a misunderstanding here.
The physical shape of the Earth and the shape of the Earth's gravitational field are inseparable. Whenever I wrote: the shape of the Earth matters, I meant both: the shape of the Earth and the shape of the gravitational field.

When Dr Benn dismissed turntables he was presumably referring to flat turntables, for flat turntables are not a good model at all for coriolis effects in the atmosphere. Only a parabolical turntable is a good model (and of course when a parabolical turntable has been manufactured, it must later be used with the original rotation rate. Then fluid on the parabolic will form a layer that is equally thick everywhere, modeling the even thickness of the Earths atmosphere)

The way the oceans distribute over the Geoid does of course not depend on the specifice shape of the Geoid, it depends on the shape of the gravitational field of the Geoid. For example there is a location in the Indian ocean, where the density of the rock of the seabed kilometers below is so high, that locally there is stronger gravity than on average on that latitude. There, the deviation of the Geoid from the theoretical oblate spheroid is about a hundred meters.

If you have a rotating parabolic turntable, with a layer of fluid on it, then the pressure in the fluid is not the same everywhere. The pressure in the fluid is related to the slope of the turntable: steeper section means stronger horizontal component of gravity, more pressure. In a rotating fluid the pressure is not uniform, and that affects the physical behavior of the fluid, you get the fluid behavior called Taylor columns. ---Cleon Teunissen | Talk 10:53, 1 August 2005 (UTC)

---

Lots said. Lots true. Lots I cannot see the relevance. But you mix it all up. Let's try and settle one issue at a time. On my rotating cubic(!) Earth (a thought experiment) with enough air to cover it entirely, would there be observable coriolis effects? Yes. So the shape of the Earth (and the shape of its gravitational field) does not matter - there will be coriolis effects in the atmosphere. Agreed? Paul Beardsell 11:03, 1 August 2005 (UTC)

I don't think it is possible to derive information from this thought experiment, shape of mass and shape of gravitational field are inseparable. If you add enough atmosphere to cover all of the solid, then a large part of it would be pressed in to the density of liquid form, and you end up with effectively an oblate spheroid.

The issue is that you and I do not envision the same mechanism. The mechanism I recognize is with a centripetal force, I would guess that your vision more resembles the context of ballistics. I expect that air that has a velocity with respect to the Earth from east to west will tend to slide to the north (and if there is no obstruction it will slide north). I would guess that you expect air that has an east-to-west velocity will tend to follow what is called a great circle: the straightest path that follows the surface of a sphere. --Cleon Teunissen | Talk 11:28, 1 August 2005 (UTC)

I am fairly confident are not saying that coriolis effects only occur in 20% Oxygen 80% Nitrogen. And I haven't done the calculation to see if the pressures involved on my cubic Earth would liquify them. But, maybe. Let me additionally assume a Hydrogen atmosphere. That won't go to liquid. There will be coriolis effects? Yes! Agreed? Paul Beardsell 11:35, 1 August 2005 (UTC)

It's not whether it liquifies or not, Oxygen and Nitrogen won't liquify unless they're, like, 100 Kelvin. But they will be compressed to that degree of density. (Which is why I wrote "density of liquid form). As I see the coriolis effect on Earth, a gravitational field with an irregular shape is hard to think through. I would guess that you use the spherical world approximation anyway, so for you it would not matter. --Cleon

I'm confused. You concede coriolis effects will occur regradless the shape of the Earth (or its gravitational field)? Paul Beardsell 12:19, 1 August 2005 (UTC)

Which context do you have in mind? Angular acceleration accompanying contraction or ballistics? I'm not pulling your leg. I see a genuine difference there. --Cleon Teunissen | Talk 12:42, 1 August 2005 (UTC)

There is only one coriolis effect. But I suggest we talk about winds, in this context. Although I am happy to talk about an ice skater at the Cubic Global Ice Skating Championships or about a missle fired during the 3rd Cubic Global War. It's all the same coriolis effect. Paul Beardsell 13:03, 1 August 2005 (UTC)

Do not make physics more complicated than necessary

The coriolis effect is made apparent by the relative motion of a rotating and non-rotating body. [User:Psb777|Paul Beardsell] 12:55, 1 August 2005 (UTC)

I've read your response and it seems to me that you are making things unnecessarily complicated.

I don't think I have. I have explained both(!) of your coriolis effects in three short paragraphs above and I have done so without telling you a story about the gyrocompass. You continually complicate the coriolis effect. Please stop. Paul Beardsell 13:45, 1 August 2005 (UTC)

[Cleon continues with his story...]

I would like to tell you about a device called Gyrocompass

A Gyrocompass is a gyroscope that is mounted in such a way that when the gyroscope is spinning, the axis of the gyroscope will align himself with the axis of the Earth. It can for example be used in tunnelbuilding, to assist in maintainin direction. The gyrocompass is given a stable platform, the gyroscope of the device is spun up, and after about a quarter of an hour the spinning axis of the gyroscope will have aligned itself with the axis of the Earth.

How this can be is straightforward: a gyroscope has the property that when it is spun up it tends to maintain the same direction in space; if you do try to rotate it, it will precess. The Gyroscope is positioned on Earth, and the Earth is rotating in space, so it is exerting a force on the gyroscope, pulling it along in the rotation. The gyroscope will precess because of that. The precession is dampenen in such a way that the gyroscope brings itself in alignment with the Earth's axis.

There is a tension as long as the vector of the angular momentum is rotating with respect to space. When the spinning axis of the gyroscope has come in alignment with the Earth's axis the tension is resolved.

The gyrocompass is so reliable because it does not measure any outside thing, the gyrocompass works because a force is required to rotate it with respect to space.
A gyrocompass can for example be used by a submarine. The submarine settles down on the bottom, so that it will transfer the rotation of the Earth unperturbed, and then the gyrocompass can be spun up to determine the direction of the geographic north.

From the wikipedia article:
[...] true North is the only direction for which the gyroscope can remain on the surface of the earth and not be required to change. This is considered to be a point of minimum potential energy.

Since the operation of a gyrocompass crucially depends on its rotation on Earth, it won't function correctly if the vessel it is mounted on is fast moving, especially in East-West direction.

The gyrocompass was patented in 1885 by the Dutch Martinus Gerardus van den Bos; however, his device never worked properly. In 1903, the German Herman Anschütz-Kaempfe constructed a working gyrocompass and obtained a patent on the design. In 1908, the American inventor Elmer Ambrose Sperry patented a gyrocompass in the US. When he attempted to sell this device to the German navy in 1914, Anschütz-Kaempfe sued for patent infringement. Sperry argued that Anschütz-Kaempfe's patent was invalid because it did not significantly improve on the earlier van den Bos patent. Albert Einstein testified in the case, first agreeing with Sperry but then reversing himself and finding that Anschütz-Kaempfe's patent was valid and that Sperry had infringed by using a specific dampening method. Anschütz-Kaempfe won the case in 1915.

The Einstein Papers Project Caltech. The story of Einstein being consulted on the matter of the gyrocompass patent. The story of Einstein and the gyrocompass is about 3/5th down the page. I add the story about Einstein to show that he was not surprised. general relativity is was concieved to describe all aspects of motion, inclusing the behavior of gyrosocpes. Of course, Einstein knew about gyroscopes.
--Cleon Teunissen | Talk 13:37, 1 August 2005 (UTC)

By this story you have not shown either (i) there are two coriolis effects or (ii) were the Earth a different shape that coriolis effects would not occur. What have you shown? Paul Beardsell 13:49, 1 August 2005 (UTC)

The gyrocompass, and many other devices, show that is it unnecessarily complicated to struggle with only seeing relative rotation. It is straightforward to measure rotation relative to space. That is the big difference with linear motion. Linear motion is relative, so you can only measure relative linear motion. But you can measure rotation with respect to space, so it makes no sense to pretend that everything should be treated as relative rotation.

So the explanations become dead simple. The ice-skater, pulling in her arms starts spinning faster, with respect to space. Rotation should always be treated for what it is: rotation with respect to space.
Read the article about the Sagnac effect and ask the other contributor to that article, EMS. He will confirm that the Sagnac interferometer does what is described in the article.

In the case of the ice-skater there is real rotation, in a projectile flying in a straight line there is no rotation, so it is different physics, and no decree will alter that. --Cleon Teunissen | Talk 14:06, 1 August 2005 (UTC)

You do not invalidate anything I have written here. Paul Beardsell 14:31, 1 August 2005 (UTC)

Babylonian confusion

How much of the previous discussion was merely Babylonian confusion?

Angular Acceleration Accompanying Contraction

Apart from what name we use for the dynamics depicted in the image, it appears we do agree about what is happening in that picture. Pull on the string and the rotation rate of the other ball goes up.

Angular Acceleration Accompanying Contraction is the dynamics that Gaspard Gustave Coriolis described in his 1835 paper. Gaspard Gustave Coriolis was dealing with machines. That is the historical origin.

Anyway, it would of course be ridiculous to try and explain the dynamics of Angular Acceleration Accompanying Contraction in terms of coordinate transform.

Of course, in coordinate transformation to a rotating coordinate system only one coriolis term appears. There is only way to transform to a rotating coordinate system.

This is the history section of the coriolis effect article. Do you agree with it? It is well referenced. If you think the history section is wrong, please say so. --Cleon Teunissen | Talk 15:34, 1 August 2005 (UTC)

Gaspard described a number of things. All the things he described are not together the Coriolis effect. As I understand it the Coriolis effect is that now described by the 1st paragraph of the article. Paul Beardsell 16:00, 1 August 2005 (UTC)

apparent

William, I understand what you're doing. The deflection is real from the rotating frame of reference. And no frame of reference is any better than any other. But the observed deflection is only "apparent" from that frame of reference. A re-wording, just placing "apparent" elsewhere in the sentence should satisfy us both. What say you? Paul Beardsell 15:57, 1 August 2005 (UTC)

The two animated gifs Cleon made.

Elliptical orbit As seen from a non-rotating point of view

Elliptical orbit As seen from a co-rotating point of view

Consider the two animated gifs you made: Elliptical orbit As seen from a non-rotating point of view Elliptical orbit As seen from a co-rotating point of view

which are reconstructions of the MIT lab films. They are NOTHING BUT demonstrations of the coordinate transformation. And yet you must find it explains something ("to explain is to unfold, to reveal what was hidden...") , because you have copied them to several places. GangofOne 10:27, 1 August 2005 (UTC)

Hi GangofOne. Yes, the animations are meant to explain. The difference between the two animations is the coordinate transformation. I hadn't seen the MIT lab films, but before I made the animations I was imagining the animations, and when I made them it was quite exciting to actually see them just as I had envisioned them.

The coordinate transformation is just the coordinate transformation. The real explanation, the physical mechanism, is in both the animations: the elliptical orbit. the coordinate transformation just shows something about apparent motion.
Of course, it is yet another step from those animations to cyclonic flow. The animations correspond to what in meteorology is called 'inertial wind' and in oceanography, inertial oscillation.

On the northern Hemisphere, inertial wind will blow clockwise, and cyclonic flow blows counterclockwise. But it is straightforward to see why cyclones flow counterclockwise and inertial wind clockwise, so I didn't add to the length of the article by writing about that too. --Cleon Teunissen | Talk 16:17, 1 August 2005 (UTC)

The visual change involved in a coordinate transformation is not hard to imagine, for all the proportions remain the same; the picture as whole rotates, the content is not altered. Imagine you are in a space ship, on your way to Mars. You see the Earth rotating. Or you are on Mars itself, and Mars is at a point of shifting from "forward" motion to "regrograde" motion. Then you are statonary with respect to the Earth, and you will see it turning, and you will see one or more cyclones in the atmosphere, I imagine the cyclone's motion may then look a bit like the motion of a rolling wheel. What would the overall trajectory of a wheather balloon look like, if you would be able to track the motion for days? --Cleon Teunissen | Talk 17:56, 1 August 2005 (UTC)

Cleon, why not comment in place? Or cut'n'paste rather than copy'n'paste. We now have duplication of Gang1's comments. Paul Beardsell 17:38, 1 August 2005 (UTC)

You're right. In this case I should have moved Gang1's comment.--Cleon Teunissen | Talk 17:56, 1 August 2005 (UTC)

Cleon, one way to improve these 2 gifs is put a marker on the edge of the turntable so we can see it rotating or not, in the 2 cases. In the MIT films you see a dot attached to the edge, (probably the camera stand), that makes it clear right away which film is fixed viewpoint and which is corotating. GangofOne 23:35, 1 August 2005 (UTC)

Apparent

P and I disagree about the word apparent in the intro:

In physics, the Coriolis effect is the apparent deflection of a free moving object as observed from a rotating frame of reference. The Coriolis force is the force that is applied to a free moving body to make it appear to travel in a straight line from the perspective of a rotating frame of reference. The effect was first described by Gaspard-Gustave Coriolis, a French scientist, in 1835.

One example of the Coriolis effect is the apparent deflection from straight ahead of a ballistic missile as viewed from the Earth. Another is the cyclonic nature of large scale winds.

I don't think it should be there. This is not a question of whether the force is fictitious/apparent or not. The sentence is: the Coriolis effect is the apparent deflection of a free moving object as observed from a rotating frame of reference. But viewed from the rotating frame, the deflection is not apparent, it is quite real: within the rotating frame, the body does not move in a straight line as viewed in that frame. Hence, the word apparent is unneeded, and indeed wrong. William M. Connolley 18:43:57, 2005-08-01 (UTC).

I suppose the word apparent is appropriate when it is assumed that any observer who is aware he is looking at things from a rotating point of view should keep that in the back of his mind, and should at all times be also imagining the same motion as seen from a non-rotating point of view.

For example, suppose a satellite is brought into a circumpolar orbit, and the orbit is highly eccentric, going several Earth diameters away, and then almost skimming the Earth. As seen from a point of view that is stationary with respect to the Earth that would show up as a rather corkscrew shaped trajectory.

It seems like a good idea then, to keep in the back of one's mind that the natural celestial motion is motion in a plane. That is what celestial bodies orbiting other celestial bodies do. Astronomers find double stars, and those double star system are always seen to move in a plane. To my knowledge, Astronomers never have to transform to make it a plane, the doppler shifts they record are always consistent with two suns in planar orbit around each other. --Cleon Teunissen | Talk 19:10, 1 August 2005 (UTC)

In the rotating frame of reference, the deflection is quite real - there is nothing apparent about it. For the force, I've tried replacing "apparent force" which I hate with "The Coriolis force is the force that is needed in the equations of motion in the rotating frame to make the equations balance." which is definitely true, though may not be to everyones taste.

I find all these efforts to "explain" the coriolis force quite unhelpful. In the rotating frame, for the purposes of atmospheric motion, the force is fmv x k - what more do you need? William M. Connolley 20:25:56, 2005-08-01 (UTC).

What is needed is an explanation that works fine in all frames.

You just don't get it, do you? The equations of motion *do* work fine in all frames. And if you swap to a rotating frame you need to add a term that looks like the coriolis force. William M. Connolley 10:17:37, 2005-08-02 (UTC).

When the Apollo mission astronauts were on the Moon, they were watching the Earth from a frame that rotates with respect to the Earthfixed stars with a period of about 28 days, instead of 24 hours. Those astronauts made photopictures, shot films, they saw the cyclones and all of the atmosphere. It does not matter, rotating once per 28 days or once per 24 hours, you see the same cyclones. So: what is the proper explanation for the cyclones as seen from the Moon (or in general as seen from afar, and from a non-rotating point of view)? A coordinate transformation preserves everything, a coordinate transformation rotates the entire picture. --Cleon Teunissen | Talk 20:51, 1 August 2005 (UTC)

What do you see from the Moon?

Suppose that there is a huge gun, big enough to shoot something that shines so bright it can be seen from the Moon. This big gun is fired, a shot from the equator towards the north. The astronauts on the Moon see the projectile fly in a straight line. There is a whole battery of those guns, they are shooting a grid pattern. The astronauts see the entire grid of luminescent ammo travel along, in straigh line motion.

So: as far as ballistics is concerned there is a difference between seeing it from the Moon or seeing it from fixed-to-the-Earth. The astronauts on the Moon, rotation rate once per 28 days which is pretty close to zero, they do see the projectiles travel in straight lines, while an observer stationary with respect to the Earth sees a curvilinear trajectory. Interestingly, the observer on Earth sees curvilinear trajectories, but the arrangement, the grid of projectiles has the same form, no distortion of the grid. And of course: coordinate transformation is global, the whole picture is rotated. --Cleon Teunissen | Talk 21:47, 1 August 2005 (UTC)

This site presents a composite image, from satelite date, of the Earth as seen from the Moon One of the options is to change to a perspective as seen from the Moon. It would be interesting to make an animation of a sequence of images from that site. --Cleon Teunissen | Talk 05:36, 2 August 2005 (UTC)

The four guns

I have added a discussion of ballistics to the opening section of the article. Interestingly, if the square formation is very large, then tidal force from the Earth comes into the picture. The tidal force will tend to compact the formation of four projectiles, and this tightening of the formation would look the same from the Moon and from the Earth, for coordinate transformation is global: it preserves relative velocities. --Cleon Teunissen | Talk 06:52, 2 August 2005 (UTC)

I've removed:

Imagine four huge guns, pointed straight up, the four guns are on the four corners of a large square. The guns are fired simultaneously, and the projectiles are shining bright, so bright astronauts can see them from the Moon. Because the projectiles are fired straight up, the Earth's gravity does not bend their trajectory, it only decreases their velocity. Astronauts on the Moon will see the projectiles move in a straight line, and they see the Earth rotate underneath the projectiles. As seen from a point on the Earth, the projectiles follow a curvilinear trajectory. Both the astronauts and the viewers on the Earth see the projectiles in the same square formation all the time, for a rotation of the frame of reference is global; the relative positions in the formation are preserved.

In retrospect: the example can work, but not in the way that it is presented here. If the guns fire perpendicular to the surface of the Earth, (the projectile leaving the nozzle at a velocity higher than excape velocity) then their motion will be a hyperbolic trajectory with respect to the center of gravity of the Earth. But the reasoning presented in this 'four guns scenario' is one in which the projectiles are fired in such a way that the sideways velocity of the gun is compensated for; the reasoning of the escape-velocity-projectiles assumes the projectiles have been fired into perfectly non-orbital motion: a straight line as seen from a non-rotating point of view, with the Earth rotating away underneath the grid of projectiles. The example can work, but a repaired version would make the story too cumbersome. --Cleon Teunissen | Talk 06:26, 3 August 2005 (UTC)

It may well fit somewhere, but I don't think it belongs in the intro. I'm also doubtful about the physics: if the square is large, then the rotational velocities the bullets have on firing will be different and he square will deform. William M. Connolley 08:22:43, 2005-08-02 (UTC).

Yes, there will be a small shearing deformation. But it seems you have missed the point: at any point in time the viewers on Earth and the astronauts on the Moon see the same formation: the same relative positions in the formation. They see different trajectories, but at each point intime the same formation

Yes... of course. So what? William M. Connolley 10:14:41, 2005-08-02 (UTC)

So what kind of coriolis deflection of wind will astronauts see from the Moon? The diagram on the right has red arrows for the coriolis force. Are you saying that the astronauts on the Moon, looking at the Earth, will see exclusively effects from the pressure gradient force, and no other influence?

You can't *see* forces. This seems to be your fundamental misapprehension. You can only see things moving with respect to your coordinante system. William M. Connolley 10:14:41, 2005-08-02 (UTC).

William, you are not answering my question. You are changing what I say to something else, and then you reject that twisted version. I did not ask about forces, I asked what direction of flow they will see. What effects will they see. When astronauts on the Moon look at the Earth, will they recognize a cyclone (if a cyclone has formed on Earth) --Cleon Teunissen | Talk 10:52, 2 August 2005 (UTC)

The red arrows in the diagram stand for something. Whatever it is those red arrows stand for: it does something that contributes (together with pressure gradient force) to restructuring of the air flow, a restructuring that does not preserve relative positions.

But a coordinate transformation preserves the global picture. A coordinate transformations preserves the relative positions, and if there are relative velocities the coordinate transformations preserves the relative velocities.

Therefore the red arrows in the diagram on the right are unrelated to coordinate transformation.
--Cleon Teunissen | Talk 09:40, 2 August 2005 (UTC)

---

new comments not at the bottom, use history to find.GangofOne 09:54, 2 August 2005 (UTC)

Hi, GangofOone, you removed the comment, but it was not a new entry, I was completing something I had started myself. I forgot to sign that one, but you can see in the history that what I appended to was written by me, and it belongs together.--Cleon Teunissen | Talk 10:05, 2 August 2005 (UTC)

The meaning of the red arrows

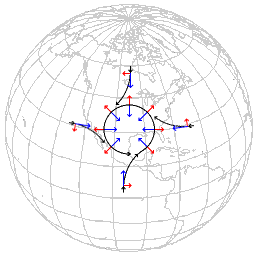
In the current article, Woodstone has written about the meaning of the red arrows in the diagram, as seen from a non-rotating point of view.

I would like to formulate it as follows: As seen from the non-rotating point of view, the small red arrow on the flow from south to north (pointing sideways) can be interpreted in terms of conservation of angular momentum. The radius of circular motion decreases, so that will be accompanied by an increase of angular velocity, showing up as increase of velocity with respect to the Earth.

In the case of the flow from west to east there is a situation that looks a bit like cars going round on a banked circuit. How high up the bank the car will go depends on the speed of the car, (the driver, once he has steered into the banked curve, pretty much has to follow where his momentum leads him, otherwise he'll likely lose grip). There may be a lane that is cleaner, and if the driver is going faster than the fastest possible speed for that clean lane, he will drift outside, not having enough grip.

Air that is flowing with respect to the Earth in west-to-east direction is in that kind of situation; it's speeding.

That is what the two diagrams on the right stand for.
The whole line of thinking that is looking at the overall angular momentum does not need to consider the coordinate transformation, for that line of thinking is looking at it from a non-rotating point of view in the first place. --Cleon Teunissen | Talk 12:25, 2 August 2005 (UTC)

Reference. The inertial oscillations are physics, unrelated to coordinate transformation

Homepage of Dale R. Durran

Dale R. Durran
Professor of Atmospheric Sciences
Adjunct Professor of Applied Mathematics
Atmospheric Sciences
University of Washington, Seattle

The physics of the inertial oscillations
Parabolic turntable

Opening paragraph of the article about inertial oscillations:
It is demonstrated that the inertial oscillation is not produced exclusively by "inertial forces", and that the inertial oscillation appears as oscillatory motion even when viewed from a nonrotating frame of reference. The component of true gravity parallel to the geopotential surfaces plays a central role in forcing the inertial oscillation, and in particular it is the only force driving the oscillation in the nonrotating reference frame.

From Section 2. The inertial oscilation as viewed from a nonrotating reference frame:
One consequence of the resulting equatorial bulge in the Earth's geopotential surfaces is that there exists a poleward component of true gravity parallel to the geopotential surfaces at all latitudes except 0 degrees and 90 degrees; or more simply, the equator is uphill.
[end quote from article]

The article by Dale R Durran describes an obvious observation: the pattern of motion that is known as inertial oscillations requires the presence of a physical force in order to occur. The occurrence of the inertial oscillations is unrelated to transformation of the equations of motion to a rotating frame of reference.

Due to the oblatenes of the Earth there is a component of gravitation parallel to the surface, pulling to the north. Because of this parallel to the surface gravitation-component, air mass that is stationary with respect to the Earth is kept from sliding over the surface of the Earth to the equator. Sliding to the equator is sliding uphill, sliding to the nearest pole is sliding downhill. The term for the geopotential height in the equation of motion used in meteorology, represents this gravitational potential. It is in significant respects analogous to a gravitaitonal well, such as the gravitational field that sustains planetary orbits.

A planetary orbit can be either circular or elliptical, both are stable. Air mass on the surface of the Earth that is stationary with respect to the Earth is moving along a trajectory that is a circle around the Earth's axis, staying on the same latitude. A large push will alter that circular motion into what is effectively an elliptical trajectory (most so close to the poles). An elliptical orbit can be seen as a circular orbit with an oscillation in the distance to the center of rotation. Using a parabolical turntable is a good model for understanding the physics of the inertial oscillations.
--Cleon Teunissen | Talk 12:02, 5 August 2005 (UTC)

I need to think about inertial osc a bit more, and read some more Gill, but let me point out that your favourite author Persson http://met.no/english/topics/nomek_2005/coriolis.pdf says quite clearly in section 2: The cross product indicates that F is perpendicular not only to the rotational axis but also to the relative motion. A moving body is therefore driven into a circular path, “inertia circle”.... He therefore ascribes the inertial circ to the coriolis term. You appear to have gone from is not produced exclusively in the ref above to your own The occurrence of the inertial oscillations is unrelated to transformation of the equations which your ref does not support. William M. Connolley 10:18:29, 2005-08-21 (UTC).

Comments from Coriolis' country...

I was interested in the article as it covers most of the issues related to the Coriolis force issue and shows animated pictures that ought to help the reader understand things in an innovative way.

I come from the country of Coriolis and Foucault and there may be some cultural difference between what we call the Coriolis force here nowadays and what they call it in the States. We actually prefer to speak about Coriolis acceleration.

I am puzzled by a number of things, though.

1. the first sentence leaves me unsatisfied, as an effect cannot be a force: these are different categories of things and they may be (they are indeed!) related, but please, explain how. I have seen in the discussion that the 'reality' of the Coriolis force is being questioned, which may be why the author feels more comfortable to talk about effect? But this business of whether the Coriolis force is real or not is an old debate, which has been around in high school physics classes for ever, comes up as well for the centrifugal force and can be raised also for gravity, if one think of it in the framework of general relativity ! This is actually non-issue, as forces are not universals, but rather "things" that are felt or measured within a special reference frame. If you are sitting on a merry-go-round, it is easy to feel the centrifugal force and you won't question its existence for long! If you are watching the merry-go-round from the outside, then you may question the force and consider it as a trick for engineers to balance the set of forces that is applied to the arm that hold the gondola to the rotating axis. But, this is not a very deep discussion any more!

2. the first definition of the Coriolis force given in the article is what we call here conservation of momentum: if a ice-skater stretches her arms or pulls them to her body while rotating on herself, her speed will change. Kinetic energy and momentum will be conserved. We don't connect this here to a Coriolis effect.

3. the only Coriolis effect that we speak about here is the effect related to the Coriolis force that arises when you analyze the forces that are applied to a mobile, when you use a non-uniformly moving reference frame. The sets of motion equations becomes a bit more complicated than in a Galilean frame (one that moves in straight line at constant speed) and a small term related to the vectorial velocity with respect to the frame comes up, which is the Coriolis force. This definition should somehow be given explicitly in the text, even if Wikipedia is not meant for pHDs, as this is taught to seniors in high schools now or to 1st year university students. Then every thing follows: there is no Coriolis force in a Galilean reference frame, it only shows up if the frame is accelerated. And you can develop all the nice and puzzling features that are shown in the article.

--Ifsteelman 06:57, 11 August 2005 (UTC)

What do you mean by 'showing up'?

there is no Coriolis force in a Galilean reference frame, it only shows up if the frame is accelerated. --Ifsteelman 06:57, 11 August 2005 (UTC)

Rotation (at constant angular velocity) is an acceleration. In physics. (And it is physics we are dealing with here - the words have precise meanings and the common sensical use of the words is often unhelpful - try telling a man holding a brick at arm's length that he is doing no work). The Coriolis effect is only to do with rotation. Any apparent deflection owing to other accelerations is NOT called the Coriolis effect! Please refer to the first paragraph of the article. Paul Beardsell 09:59, 20 August 2005 (UTC)

The above statement requires sharpening, I think:
Let us take the example of a mass spectrometer, with charged particles moving in a magnetic field, so that they are separated according to mass/charge ratio.

The natural frame to calculate the trajectories of the molecule fragments in the device is a Galilean reference frame. However, if I feel like overdoing things I might raise the bar and use some rotating coordinate system. No matter what coordinate system I use, the shape of the calculated trajectories will be the same; the point where the fragments will hit the detector will be the same.

So a sharp distinction must be made between force that physically shows up, like the magnetic field in the mass spectrometer, and terms that are added in the formulas for performing calculations.

If I am swinging around a pebble on the end of a rope then I am exerting a centripetal force on the pebble, and the pebble is exerting a force on me, in a direction that is the centrifugal direction for the pebble. Since I am much heavier than the pebble I am hardly moved, the pebble is in circular motion. So physically the centrifugal force is being exerted, on me, (but in the case of a pebble it doesn't move me appreciably) (Of course the pebble does not exert a centrifugal force on itself: there is no such thing as an object exerting a force on itself; an object may exert a force on another object)

In transforming to a rotating coordinate system, a term for a fictitious centrifugal force is introduced (along with a term for a fictitious coriolis force) That is not a physical force.

So there are two contexts, and in both the name 'centrifugal force' is used, but the two contexts are completely different.

All physical forces that are present in a situation remain the same under coordinate transformation, only the representation changes. When a physical force is present in the Galilean frame of reference then it is physically present in all other frames too, only the representation may be different. --Cleon Teunissen | Talk 14:19, 11 August 2005 (UTC)

It is indeed a non-issue

This is actually non-issue, [...] If you are sitting on a merry-go-round, it is easy to feel the centrifugal force and you won't question its existence for long! --Ifsteelman 06:57, 11 August 2005 (UTC)

It is indeed a non-issue.
If I am on the platform of a merry-go-round, and I have a good grip on a pole then, the pole is exerting a centripetal force on me, making me go in circular motion around the merry-go-round's axis of rotation, and I am exerting a force on the merry-go-round, (in a direction that is centrifugal direction for me), which does not affect the motion of the merry-go-round appreciably, since the merry-go-round is much heavier than me and securely attached to the ground.

I can hook a force-measuring device on the pole, and hold on to the measuring device rather than to the pole itself, and then the measuring device shows how much newtons of force the pole is exerting on me to maintain my circular motion.

That measuring device readout is the same in all frames of reference. --Cleon Teunissen | Talk 15:40, 11 August 2005 (UTC)

Extensive rewrite August 18th, the references

The extensive rewrite I did is based on the content of the following references, as listed at the bottom of the current coriolis effet article. The authors of the articles are prominent meteorologists. --Cleon Teunissen | Talk 19:41, 18 August 2005 (UTC)

The current Talk:coriolis_effect page is very large. I propose to archve it. I nobody object I will do that move in a couple of days. --Cleon Teunissen | Talk 19:41, 18 August 2005 (UTC)

• Durran, D. R., 1993: Is the Coriolis force really responsible for the inertial oscillation? Bull. Amer. Meteor. Soc., 74, 2179–2184; Corrigenda. Bulletin of the American Meteorological Society, 75, 261
  1200 KB PDF-file of the above article

• Durran, D. R., and S. K. Domonkos, 1996: An apparatus for demonstrating the inertial oscillation. Bulletin of the American Meteorological Society, 77, 557–559.
  1300 KB PDF-file of the above article

• Persson, A., 1998 How do we Understand the Coriolis Force? Bulletin of the American Meteorological Society 79, 1373-1385.
  374 KB PDF-file of the above article

• Norman Ph. A., 2000 An Explication of the Coriolis Effect. Bulletin of the American Meteorological Society: Vol. 81, No. 2, pp. 299–303.
  200 KB PDF-file of the above article

The animations by David H. McIntyre

David McIntyre has made number of animations that show how object would move (and appear to move) in the case of the approximation of the Earth as a perfeft sphere. David McIntyre's animations

David McIntyre animations show that under the assumption of a perfectly spherical Earth the pattern of motion called 'inertial oscillation' cannot possibly occur.

The animation on the right shows the pattern of motion of inertial oscillation, as seen from a non-rotating point of view. That is: that animation represents what you get if you transform the pattern of motion of atmospheric inertial oscillation from a co-rotating point of view to a non-rotating point of view.

All air mass on the Earth is subject to a poleward pull of a component of gravitation, each hemisphere to its own pole. In meterology that poleward pull must be taken into account, and of course it is taken into account in settting up the equations of motion, otherwise the computermodels wouldn't work at all.

If the equation of motion would be set up for a non-rotating coordinate system, it would contain a term of the form:
$2m(\omega \times v)$ * sin(latitude) because of the rotational dynamcis that is at play.
--Cleon Teunissen | Talk 20:14, 18 August 2005 (UTC)

Yeah, but this has nothing to do with the Coriolis effect in the atmosphere. The shape of the surface is only important for sliding pucks. the atmosphere is a fluid and finds its own level. If the earth were a cube there would still be the Coriolis effect. There would still be cyclonic weather systems. Paul Beardsell 09:52, 20 August 2005 (UTC)

Newtons first and second law of motion

The rewrite states:

"The result of the perpendicular to the Earth's axis component is that all objects that are co-rotating with the Earth remain on the same latitude, instead of sliding towards the equator."

That sounds wrong to me. I would expect the absence of a force parallel to the surface to have said effect.

In general I find the rewrite very repetitive and not well structured. I have a strong tendency to revert, but will study it better first. −Woodstone 20:09:28, 2005-08-18 (UTC)

BUT WRONG OR RIGHT IT IS NOTHING TO DO WITH THE CORIOLIS EFFECT. Paul Beardsell 09:53, 20 August 2005 (UTC)

Currently the rewrite is repetative because a lot of people do not accept the newtonian explanation of the rotational dynamics that is at play in the atmosphere. I wanted to make sure everything would be covered. Much repetition can be trimmed away.

I would like to point out Newtons first and second law of motion. Newtons first law states: When there is no force, or if the resultant force is zero, then an object will move in a straght line. An object smack on the equator is pulled along in rotating motion by the Earth gravitation. But what about object on higher latitudes? Newtons first law discribes that those object tend to move in a straight line, so while they would certainly not fly off the Earth (Gravitation is much to strong for that) they would slide over to the Equator if the proper centripetal force is not provided.

Your expectation with an absence of a force would apply in the case of a non-rotating planet. But the Earth is rotating, so everything on the planet is rotating, and that rotating motion of objects resting on the Earth will only persist with unchanging radius if the appropriate centripetal force is provided --Cleon Teunissen | Talk 20:29, 18 August 2005 (UTC)

Cause and effect is being mixed up. That the shape of the MIT oblate spheroid is very carefully created does not mean that the shape of the Earth has anything to do with the Coriolis effect in the atmosphere. All of the careful balancing of sideways forces on the MIT dish can be (MUST BE) neglected in a fluid! Someone at MIT said "wouldn't it be neat if we could demonstrate some consequences of the Coriolis effect on the atmosphere using solids" and ever since then people think the shape of the Earth is important when discussing the Coriolis effect. No, all is floating in the atmosphere. Paul Beardsell 01:25, 21 August 2005 (UTC)

Gravity and the Coriolis effect!

For the nth time: Gravity and the coriolis effect are unrelated! All of these pucks sliding on specially shaped surfaces are to demonstrate the coriolis force by eliminating the predominant forces (by balancing the force of gravity and the centrifugal "force") on the puck so as to allow the coriolis effect to be seen. This needs to be made plain in the article. In the atmosphere, with everything floating in everything else, those forces do not need to be balanced for the coriolis effect to be noticed. That's the nature of a fluid. And that is what the atmosphere is! Paul Beardsell 09:47, 20 August 2005 (UTC)

Shape of the Earth and the Coriolis effect in the atmosphere!

Also (and this follows simply from the previous section) the shape of the earth is unimportant when describing the Coriolis effect in the atmosphere. Were the Earth a cube cyclonic weather patterns would still occur: One way in the north, the other in the south BECAUSE the atmosphere is a fluid and, in a fluid, the shape of the container is immaterial. All of Cleon's arguments as to the sideways component of gravity owing to the particular shape of the Earth would still be irrelevant when discussing the Coriolis effect in the atmosphere. Sliding pucks? OK. Cyclones? No. Paul Beardsell 10:16, 20 August 2005 (UTC)

Shallow fluid flow on a slowly rotating planet!

This, once again, is NOTHING to do with the Coriolis effect. I am NOT saying that the relative shallowness has NO impact on meteorology. But the Coriolis effect is DIFFERENT AND SEPARATE. This article is not a general one about the weird stuff happening in the atmosphere. It is about the Coriolis effect ONLY. Paul Beardsell 20:32, 20 August 2005 (UTC)

Hmmm, I disagree somewhat: the equations are clearly related to the Coriolis effect. But... your point about this not being a general article about atmos dynamics is a good one. See my proposal at the end. William M. Connolley 10:36:06, 2005-08-21 (UTC).

I overstate my case perhaps. I worry that the shallow fluid discussion degenerates into a sliding puck argument thus falsely reintroducing the shape of the Earth as being relevant to the Coriolis effect. But a general point: that the maths of two phenomena are similar or identical does not make one phenomenum the other. Paul Beardsell 15:45, 21 August 2005 (UTC)

Re writes

Based on reading Gill, I've rewritten some of the equation sections. Some people may not like this... I've removed unneeded (and in my view unhelpful) references to "fictitious" forces.

I've clarified (I hope) the bit about gravitation/geopotential.

There is now quite a bit of centrifugal stuff in the coriolis article. Possibly, there should be a new article (shallow water equations) to contain all that stuff. It might make things clearer.

The history section appears to contain a lot of stuff about centrifiugal forces, and what Coriolis himself discovered, which isn't clearly relevant to a Coriolis effect page. Perpahs it should move to the Coriolis article instead.

I doubt that the "puck" sections about centripetal forces are helpful or relevant.

William M. Connolley 20:36:53, 2005-08-20 (UTC).

Two articles?

What is "Gill"? There seems to be some tension about what this article is about. Maybe there should be 2 articles. One called 'Coriolis force' ( I prefer that to "Coriolis effect") that deals with the general physics concept, formulas, turntables, animated illustrations, sliding pucks, tabletop fluid experements, molecular spectra, and a 2nd article called 'Coriolis and centrifugal forces in the atmosphere' that talks of the fluid approximations, cyclones, meteorology etc. GangofOne 21:34, 20 August 2005 (UTC)

Adrian Gill: the text is ref'd in the article. I think there is a split between the general coriolis effect, and the effect as it appears in meteorology: effectively, he shallow water equations. I may be brave enough to write a SWE page sometime. William M. Connolley 21:54:56, 2005-08-20 (UTC).

The more articles the merrier as far as I am concerned. But there must only be one article called "Coriolis effect". And to continue to confuse the Coriolis effect with elaborate experiments which are designed to demonstrate it is nothing but a nonsense. Dry ice pucks on oblate speroids is NOTHING to do with the Coriolis effect other than in finding a way to cancel out gravity and centripetral force so that the small Coriolis effect can be noticed amongst these other, larger forces. Also: The shape of the earth has nothing to do with the Coriolis effect. The Coriolis effect also exists independently of gravity! And independently of the "shallowness" of the atmosphere. The Coriolis effect depends on none of these things. In my view all that needs be said is said in the introduction, in the derivation of the cross product and in a rebuttal of the draining bathtub. Paul Beardsell 21:59, 20 August 2005 (UTC)

Coriolis is nothing to do with gravity, yes. Only one CE page, yes. I'm not very happy with the various "explanations" (e.g. in the air-round-a-low bit), so if you're arguing for a shorter article, yes. William M. Connolley 22:32:19, 2005-08-20 (UTC).

Derivation

There is no place for this in the article, but its fairly easy, so: the velocity in a rotating frame is related to the fixed frame by:

d(x_f)/dt = d(x_r)/dt + omega cross x_r

repeating this,

d2(x_f)/dt2 = d/dt (d(x_r)/dt + omega cross x_r) + omega cross (d(x_r)/dt + omega cross x_r)

ie:

d2(x_f)/dt2 = d2(x_r)/dt2 + 2 omega cross d(x_r)/dt + omega cross (omega cross x_r)

ie:

a_f = a_r + 2 omega cross v_r + omega cross (omega cross x_r)

Also, for those who care, the last term omega cross (omega cross x_r) which is the centrifugal bit can be re written through the magic of vector algebra as

omega cross (omega cross x_r) = 1/2 grad(|omega cross x_r|^2)

and since gravity is g.grad(1/r), the centrifugal term gets absorbed into the gravity term.

William M. Connolley 21:25:12, 2005-08-20 (UTC).

Proposal

I am (I think) somewhat in agreement with PB that there is too much extraneous stuff in this article. I am guitly of adding some of it.

I suggest (yet another, sigh) rewrite, this time to a much shorter article that is *just* about the Coriolis effect. This could be regarded as a start, and more material could be added later.

I have created such an article here: /proposed. It reflects some of my biases. I have cut out pretty well everything extraneous (I think). This has removed some valuable material that can go back in later perhaps.

William M. Connolley 11:03:05, 2005-08-21 (UTC).

Good start. I especially like the way you immediately discard the vertical components! Indeed these are tiny compared to gravity and cannot be acted on, because Earth is in the way. It might be helpful to add a small computation about the actual size of the acceleration (at gale of 100 km/h, only max 0.04% of gravity). You might avoid the word "force" even more than already done. −Woodstone 12:37:24, 2005-08-21 (UTC)

---

I really appreciate all the hard work and so I feel awkward pointing out any flaws. Esp as one has been a little controversial in the past i.e. The deflection is not real needs to be made explicit. You have:

In physics, the Coriolis effect is the deflection of a freely moving object as observed from a rotating frame of reference. The Coriolis force is used in balancing the equations of motion when expressing them in a rotating frame of reference. The effect is named after Gaspard-Gustave Coriolis, a French scientist, who discussed it in 1835, though the term appeared in the tidal equations of Laplace in 1778.

Someone who is looking at this for the first time could think that a freely moving object has actually been deflected (i.e. was not for a moment freely moving). That the deflection is apparent, not real, and only "observed" we all agree. I am in favour of saying "apparent deflection".

Also "the term appeared" refers of course to the formula and not to the name of the effect.

That the Coriolis effect is an every day occurrence with ordinary winds might be obscured by using a hurricane as the canonical example.

Paul Beardsell 16:04, 21 August 2005 (UTC)

Point away, any such comments are welcome. But there is a phrasing problem here. The previous version was:

In physics, the Coriolis effect is the apparent deflection of a freely moving object as observed from a rotating frame of reference.

(bold on apparent). This is *wrong*: in the rotating frame (as the sentence says) the deflection is real, not apparent (by deflection I at least mean "failure to move in a straight line). So I deleted it. How about ...the Coriolis effect is the deflection, as obsered from a rotating frame of reference, of a freely moving object.?

Hurricanes: yes, fair point.

William M. Connolley 17:44:50, 2005-08-21 (UTC)

I think all we need to do is consult a dictionary. "Apparent" is what appears to be, that which is observed, but which is not necessarily real. You say the deviation is "real" to those in the rotating frame of reference. Yes, but they are mistaken. There is no deviation. If there was there would be a force, and we all agree there is no force to account for the deflection observed by those rotating. So the deflection is not real. Paul Beardsell 17:54, 21 August 2005 (UTC)

This is getting a bit semantic, and we have quite enough discussion here already, so I'm going to drop this after trying once more to convince you. *As measured in the rotating frame* things don't move in straight lines. This is true, actual, and quite real. The people observing this are not mistaken: in their coordinate system the things really aren't moving in straight lines. I also dislike fictitious - I think its meaningless - but again: thats for another time. William M. Connolley 18:33:43, 2005-08-21 (UTC).

Well, yes. semantics. That's why I said "dictionary". It's all we have, in the end, the meaning of the words we use. As to what is and is not "real" (assuming we agree on what real means) the point is, as I discuss below, rotation is not relative. It is absolute. The rotating observer knows he is rotating. He knows the deviation of the free moving object is merely an illusion. That is what "illusion" means: Looks real but not real. But please do not give up on me! Paul Beardsell 18:45, 21 August 2005 (UTC)

In view of the above, I do not like the widening of the formulas to apply between rotating frames of reference. It introduces an unnecesssary complication. We should stay with a rotating frame relative to an inertial frame. −Woodstone 20:08:50, 2005-08-21 (UTC)

Added new section to proposal

Having had a bit of time whilst swimming to ponder this, I have re-added a version of the turntable section, cut down and reworded, but with the nice orange liquid pic retained. I have explained (clearly I hope) what gravity is doing in that example. I could add the equation relating g and the turntable rate.

I'm going to re-add the Inertial circles/Taylor columns bit because I have discovered (I think) a form of phrasing that explains it properly.

William M. Connolley 17:44:50, 2005-08-21 (UTC).

Ahem. I have added the Inertial circles back in, because this is pure Coriolis effect dynamics, and because I think the version I've added is OK. taylor columns probably deserve their own article.

William M. Connolley 18:50:42, 2005-08-21 (UTC).

Rotation is absolute not relative, deviation of free moving object is not real

Special relativity: Unlike linear velocity, angular velocity is not relative. Two people moving linearly relative to one another can not say who is at rest. But two people rotating relative to each other can soon determine which is the one who is really rotating. Throw a ball from one to the other and the rotating one will observe the Coriolis effect. Therefore the deflection observed is not *real*. Paul Beardsell 18:06, 21 August 2005 (UTC)

Thirty articles about meteorolical subjects link to the coriolis effect articel

I did a count of articles in the what links here pages of the coriolis effect article. About thirty of them are about meterological subjects; all those articles expect the coriolis effect article to deliver the goods.

There has been a suggestion for a split into two articles. One article would concentrate on the the meaning of the expression 'coriolis effect' in ballistics, as displayed in the illustration. A second article would be about what happens in the atmosphere.

I support organizing such a split.

I retrospect it would have been helpful if the rotational dynamics that is taken into account in meteorology would be called 'Ferrel effect' since he was the first to correctly described it.

I don't particularly mind what name is used for this or that, as long as the physics is described correctly. --Cleon Teunissen | Talk 18:12, 21 August 2005 (UTC)

---

No! There is only one Coriolis effect. That Mr Coriolis described several things does not make all those things the Coriolis effect. But, in particular, the ballistic and atmospheric manifestations of the Coriolis effect are exactly the same. But I am glad the ice skater no longer features in Cleon's list. Paul Beardsell 18:21, 21 August 2005 (UTC)

Ballistics and rotational dynamics

But I am glad the ice skater no longer features in Cleon's list. Paul Beardsell 18:21, 21 August 2005 (UTC)

Hi Paul,

The Ice skater is as ever a good example to illustrate certain aspect of the rotational dynamics that is taken into account in meteorology. But first things first.

There is a matter that has so far been avoided.

I had mentioned an airship and the vomit comet.
Recapitulating:
For the duration of the process of creating weightlessness inside, the vomit comet is following an elliptical trajectory, with the center of gravity of the Earth at one focus of that ellipse. That elliptical trajectory is, like all satellite orbit trajectories, a planar orbit. During weightlessness creating flight, the vomit comet is obviously moving inertially.

What will the flight path of the vomit comet look like as seen from the Earth? Specifically: what will it look like when the vomit comet starts its parabolic path parallel to a latitude line, in east-to-west direction?

The actual path of the aircraft takes it towards the equator, in its planar elliptical orbit. As seen from the point of view of an observer stationary with respect to the Earth, the path of the vomit comet appears non-planar with the deflection depending on relative velocity. All trajectories as seen by the Earth stationary observer, cross the equator.

On the other hand, when an airship allows itself to be swept along by inertial wind that is at some point in time moving from east-to-west, then that airship, moving along with the wind, is seen to deflect to the north.

That is the issue that has so far been avoided.
That issue has (implicitly) been adressed by Norman A Phillips, Dale R Durran, and Anders Persson. --Cleon Teunissen | Talk 19:31, 21 August 2005 (UTC)

The new proposed article (which I'm going to swap in quite soon...) clearly distinhusihes between inertial osc and hurricane dynamics, which are different, though they involve the same coriolis force (just that they are balanced by different things). I'm just going to fiddle a bit more, to add an explanation from the looking-from-outside POV. William M. Connolley 20:55:28, 2005-08-21 (UTC)

Actually I'm *not* going to add the new text, but it was going to be something like:

If a particle, initially at rest with respect to the rotating turntable, is given a small velocity, then the Coriolis effect will cause it to move in small circles (as seen from the rotating turntable), as described in the "inertial oscillations" section. It is also possible to understand this motion (though harder to quantify it) by considering the view from outside the rotating frame. From the outside, the "stationary" particle is rotating round in a large circle with the turntable, and the centrifugal force of this is balanced by the gravitational component tangenital to the surface. A small velocity away from the axis of rotation pushes the particle up away from the rotation axis, where its speed is now less than the turntable speed, so it falls backward: from the point of view of the turntable, it is thus deflected...

But I don't really like that wording. I would like to make the point that viewing it as a Coriolis deflection (in the rotating frame) or as above (but worded better) from outside, which is how CT prefers to view it. William M. Connolley 21:07:34, 2005-08-21 (UTC).

---

The ice skater rotates. Therefore (s)he has rotational dynamics. By definition. The same as any other rotating body. And a good way to demonstrate the coriolis effect would be if a flea were to jump off his/her rotating body. But otherwise what is going on is best not described as the Coriolis effect. Enough said? Paul Beardsell 19:45, 21 August 2005 (UTC)

Actually the ice skater can be perfectly explained by the same Coriolis effect. Using the same formulas, moving an arm inwards, creates an (apparent) force on the arm in the direction of rotation. The combined force on both arms (which are fixed to the body) creates a torque, making the skater rotate faster. In a non-rotational frame this looks like conservation of angular momentum. But I agree it's better to leave this out of the article, especially because in this example the rotation does not maintain constant velocity, so the formulas may change. −Woodstone 20:04:23, 2005-08-21 (UTC)

As I said, "best not described". Better described as conservation of momentum. Paul Beardsell 20:12, 21 August 2005 (UTC)

---

Presumably you refer to the northern hemisphere. And you refer to air flows occurring without pressure difference. Because, whereas the pressure difference is necessary to get things moving, the pressure difference has nothing to do with the Coriolis effect. Agreed? Paul Beardsell 19:45, 21 August 2005 (UTC)

Yes, I am thinking northern hemisphere all the time. Pressure gradient force is (of course) not involved in coriolis effect. I am talking about the wind that is called 'inertial wind'; the wind that can remain some time (eventually friction dissipates the energy) without a pressure gradient.

It is important to be able to visualise the motion of air parcels in the inertial oscillations, both from a co-rotating point of view, and from a non-rotating point of view. It is equally important to be able to visualise satellite orbits, both from an stationary-with-respect-to-Earth point of view and from a non-rotating point of view.
--Cleon Teunissen | Talk 20:34, 21 August 2005 (UTC)

Track the vomit comet and track the inertial wind, starting with motion that is east-to-west with respect to the Earth. Their motion is different --Cleon Teunissen | Talk 20:34, 21 August 2005 (UTC)

---

If you don't travel fast enough the Coriolis effect prevents you crossing the equator (or reaching the pole) and you end up moving in a circle (from the viewpoint of the rotating frame of reference). That is the difference between the slow balloon and the ballistic vomit comet. Paul Beardsell 03:08, 23 August 2005 (UTC)

What the referenced articles say

You appear to have gone from is not produced exclusively in the ref above to your own The occurrence of the inertial oscillations is unrelated to transformation of the equations which your ref does not support. William M. Connolley 10:18:29, 2005-08-21 (UTC).

Unfortunately, Anders Persson is contradicting himself on that specific point. It is not possible to have it both ways, like it is impossible to have pi simultaneously be a transcendent number, and the ratio of 355 and 113.

All of Persson's articles are breathing the notion that the inertial oscillations are to be understood as oscillations in radial distance and tangential velocity in a gravitational potential. That is to be seen as the physical cause. If you ask Anders Persson he will confirm that. --Cleon Teunissen | Talk 20:25, 21 August 2005 (UTC)

Cleon, I am unsure if you think you're still talking about the Coriolis effect. But if you are, the Coriolis effect and gravity are not dependent on one another. If Anders Persson is unreliable I suggest you choose another reference. Paul Beardsell 20:34, 21 August 2005 (UTC)

Anders Persson is talking all the time about the rotational dynamics that is influencing the winds. I know that in your opinion the expression 'coriolis effect' ought to be used exclusively to refer to ballistics. I know that in your opinion Anders Persson is using the expression 'coriolis effect' inappropiately. My impression is that most meteorologists know the expression 'coriolis effect' only in a meteorological context, and there it means roughly: "that which causes winds to not flow straight down a pressure gradient". It is necesseary to reoognize that the authors Dale R Durran, Anders Persson and Norman A Phillips are using that much looser meaning of coriolis, so some translation is needed there.

I translated my version of the article, I changed the wording into 'rotational dynamics' whereever that was appropriate.

As I said, I don't particularly mind what name is given to what, as long as the physics is described correctly. --Cleon Teunissen | Talk 05:01, 22 August 2005 (UTC)

Thin fluid layer dynamics on a parabolic rotating turntable

William M Connolley is considering the following approach:

If a particle, initially at rest with respect to the rotating turntable, is given a small velocity, then the Coriolis effect will cause it to move in small circles (as seen from the rotating turntable), as described in the "inertial oscillations" section. It is also possible to understand this motion (though harder to quantify it) by considering the view from outside the rotating frame. From the outside, the "stationary" particle is rotating round in a large circle with the turntable, and the centrifugal force of this is balanced by the gravitational component tangenital to the surface. A small velocity away from the axis of rotation pushes the particle up away from the rotation axis, where its speed is now less than the turntable speed, so it falls backward: from the point of view of the turntable, it is thus deflected...

But I don't really like that wording. I would like to make the point that viewing it as a Coriolis deflection (in the rotating frame) or as above (but worded better) from outside, which is how CT prefers to view it. William M. Connolley 21:07:34, 2005-08-21 (UTC).

Yeah, I prefer the outside view, for that is the most general view.

No it isn't. The two views are equivalent. But the outside view is hard to do quantitatively. William M. Connolley 08:28:56, 2005-08-22 (UTC).

Paul Beardsell and Woodstone have pointed out that interpreting in terms of coriolis effect is suitable if the difference in angular velocity is constant. In the case of ballistics there is the motion of the ballistic projectile moving in inertial space, and there is the rotating coordinate system, rotating at constant angular velocity.

In the case of motion of a thin fluid layer on a parabolic rotating turntable, you get that whenever the distance of a fluid parcel to the rotation axis changes, the angular velocity of that fluid parcel changes (conservation of angular momentum).
The angular velocity at any point in time is subject to overall rotational dynamics, making it cumbersome to interpret the fluid dynamics in terms of ballistics-coriolis-effect.

In ballistics, the projectile is moving in a straight line in inertial space. In fluid dynamics of a thin fluid layer on a parabolic rotating turntable inertial oscillation of any fluid parcel is complicated curvilinear motion as seen from the outside point of view, and coordinate transformation to a rotating coordinate system transforms that to another curvilinear motion. --Cleon Teunissen | Talk 06:25, 22 August 2005 (UTC)

You have misunderstood. In all cases (inc fluid dyn) the rotation rate - omega - is constant (or at least, in all cases considered here). William M. Connolley 08:28:56, 2005-08-22 (UTC).

You have misunderstood. In the case of thin fluid layer dynamics on a parabolic rotating turntable, there is the constant angular velocity of the turntable, and there is the angular velocity of fluid parcels with respect to the axis of rotation of the turntable. In the case of inertial oscillation of part of the fluid layer, fluid parcels are changing in angular velocity with respect to the axis of rotation of the turntable (conservation of angular momentum). The angular velocity with respect to the axis of rotation of the turntable is oscillating then.

Apart from that one can define a local angular velocity of the inertial oscillation, a local angular velocity as seen from a co-rotating point of view. In the idealized case that angular velocity is constant.

In real experiments with parabolic turntables the vertical component of the motion of the surface of the parabolic turntable also comes into play, making the local angular velocity non-constant. In meterology there is that latitudinal variation in the magnitude of the rotational dynamics effect causes mass to be transported westward, the socalled $\beta$-effect --Cleon Teunissen | Talk 08:47, 22 August 2005 (UTC)

The fundamental criterium of distinguishablilty

Yeah, I prefer the outside view, for that is the most general view. --Cleon Teunissen | Talk 06:25, 22 August 2005 (UTC)

No it isn't. The two views are equivalent. But the outside view is hard to do quantitatively. William M. Connolley 08:28:56, 2005-08-22 (UTC).

The two views are not equivalent, for they are distinguishable.
There is a fundamental difference in the physics of linear velocity and the physics of angular velocity.
Recapitulating the principle of relativity of inertial motion:
That inertial motion is relative is illustrated by the following. An observer is in a spacecraft, moving inertially, and he performs a number of experiments (chemical, dynamic, electromagnetic) Then he accelerates for a period of time, stops accelerating, and then he performs the same experiments again. He will then see that the outcomes of the experiments will be the same.

This pattern of observation is not to be seen in angular velocity. If the spacecraft goes through some angular acceleration, then when the rotation rate is constant you can measure exactly how fast you are rotating: it is physically distinguishable. Angular velocity is not relative

It is of course perfectly possible to transform the equation of motion to a rotating frame of reference. This equation of motion for the rotating frame of reference needs an input that is not needed in the equation of motion for the non-rotating frame of reference. It needs the rotation with respect to the non-rotating frame of reference.

So while it is mathematically possible to transform, you are inevitably referring to the non-rotating frame of reference in order to perform a calculation at all.

An observer with knowledge of physics can from observed trajectories infer a particular point of view is actually rotating, so then he will mentally transform the observed trajectory back to the motion in inertial space. The motion in inertial space is the most general view. --Cleon Teunissen | Talk 09:14, 22 August 2005 (UTC)

This is all wrong. GR tells you that all frames are equivalent for describing the laws of physics. Its just that you get change-of-coordinates forces included. I don't see any of this helping at all to write a decent Coriolis effect article. Lets stick to the point. William M. Connolley 10:33:59, 2005-08-22 (UTC).

What you are referring to is that in the general theory of relativity a full set of transformations is available to transform to any choice of frame of reference. You are confusing that with pysical equivalence.

Ask EMS. He will confirm that GR is not about proving physical equivalence. EMS will confirm that angular velocity is not relative. EMS will confirm that a ring laser interferometer measures absolute velocity.

On a deeper level: The general theory of relativity does raise deep philosophical questions regarding the nature of motion. John D. Norton has written a fine article about those issues. General Covariance and the Foundations of General Relativity: Eight Decades of Dispute

But it is very unhelpful to jam a fragment of a GR concept into newtonian dynamics. You end up with a hybrid that is both quasi-newtonian and quasi-GR. A discussion of physics must be either consistently in terms of newtonian dynamcis, or consistently in terms of GR, for the two theories are different paradigms. --Cleon Teunissen | Talk 11:51, 22 August 2005 (UTC)

I really don't think you know what is going on. Persuade EMS to comment if you can, since I think he does know. But all this is irrelevant to writing a CE article. William M. Connolley 12:56:35, 2005-08-22 (UTC).

You are vacillating. You were the one to bring up GR, now you state that bringing up GR is irrelevant. You need to make up your mind about that. --Cleon Teunissen | Talk 13:36, 22 August 2005 (UTC)

New version inserted

I have inserted my proposed new version (with the sole change of removing "fictitious", but I won't care if someone re-adds it) as discussed above.

Is it time to archive all the talk on this page?

William M. Connolley 11:09:41, 2005-08-22 (UTC).

Yes the Talk page is humongous. It badly needs to be archived. --Cleon Teunissen | Talk 11:19, 22 August 2005 (UTC)

The version of William M Connolley

William M Connolley has performed a peculiar move. He has copied my explanation of the inertial oscillations (only put in other words), but now he suddenly says they are due to the coriolis effect.

Let's have a look at that.

We have the rotating turntable of the MIT demonstrations, about one meter in diameter. Have a little puck co-rotating with that and have a little mechanism shoot a pea up in the air. There is no way that pea is going to follow a trajectory that looks like an inertial oscillation as can occur in a layer of fluid on a parabolic rotating turntable. The pea will follow a parabolic trajectory with the horizontal velocity it had when it was "launched". The parabolic turntable is rotating underneath it, and that is an example of the ballistic-coriolis-effect. If the pea is launched in west-to-east direction it will move towards the rim. If it is launched in east-to-west direction it will move towards the rim too.

An actual inertial oscillation in the thin fluid layer on the parabolic rotating turntable is being deflected towards the center of rotation twice every rotation of the turntable.

Does William M Connolley claim that both these patterns of deflection must be understood as forms of the ballistic coriolis effect?

I'm not totally sure about this, but I think that if you shoot the pea in the air then you lose the gravitational force that is counteracting the centrifugal force (of the overall turntable). So your example is no good. William M. Connolley 14:24:31, 2005-08-22 (UTC).

Interestingly, you are actually confirming the version of the article that I wrote. As long as the object is resting on the sloping surface it is subject to a centripetal force. The centripetal force is provided by the Earth's gravitation, redirected by the slope of the surface. For an object that has been launched the decomposition into component perpendicular to the surface and component perpendicular to the central axis of rotation does not apply.

So what according to you is the case with ballistics? The example of the pea being shot up into the air is ballistics. As seen from the rotating point of view the horizontal component of the pea's motion is not a straight line. What name would you give to that apparent deflection? --Cleon Teunissen | Talk 15:15, 22 August 2005 (UTC)

Later, William M Connolley writes:

In an inertial circle, the force balance is between the centrifugal force (directed outwards) and the Coriolis force (directed inwards).

How is the reader to understand that? As seen from a co-rotating point of view there is motion along an inertial circle. What we call 'centrifugal force' is inertia: a centripetal force is required to maintain circular motion. Likewise, if you have a rotating disk, with a straight line drawn from the center to rim of the disk, and you want to run over that straight line, you will experience the fact that a force must be provided to change the velocity of an object: you experience inertia.

So what is the story of inertia tugging outwards (centrifugal) and inwards (coriolis) simultaneously? (in the case of inertial oscillation) Does William M Connolley believe that inertia is tugging at the parcel of fluid in two opposite directions simultaneously? Does William M Connolley believe there are two kinds of inertia that can oppose each other?--Cleon Teunissen | Talk 14:07, 22 August 2005 (UTC)

Its what the equations say. Learn to love the equations. Fewer words, more maths, less confusion. BTW, in the interests of previty, "WMC" is fine. William M. Connolley 14:24:31, 2005-08-22 (UTC).

Not every equation that is written down has physical meaning. One of the finest examples of that is the initial suspicion against integration and differentiation. How can a continuous process be calculated with a procedure that divides the process in little steps? It is very common to have mathematical procedures that "lift" a calculation over a mathematical difficulty. The end result of the calculation is correct then, but the mathematical "bridging" has no physical meaning.

Another example: the mathematical procedures to calculate oscillations in electric circuits. That procedure involves solving second order differential equations, and to solve those a procedure involving imaginary numbers is used. The procedure is fine, and at the end of the calculation the real part of the solution space is retained, being the correct solution to the engineering problem, and the imaginary part of the solution space is discarded.

It is important to discern when the equations represent real physics, and when the equations represent a technique to speed up calculations. --Cleon Teunissen | Talk 15:15, 22 August 2005 (UTC)

Hey!

I really like this new version. Great improvement. Long live the one Coriolis effect. Paul Beardsell 02:48, 23 August 2005 (UTC)

Confusion

I am attempting to understand Cleon's assertion that there are two different types of Coriolis force. In the northern hemisphere the floating balloon circles to the left whereas a ballistic missile turns to the right. But that is because the winds are spiralling towards the low pressure. Note what happens to high pressure regions: The winds circle in the other direction (the same way as the ballistic missile!). Adding the Coriolis effect prolongs the duration of the low pressure region and shortens the duration of high pressure region. Paul Beardsell 03:35, 23 August 2005 (UTC)

No, there are not two types of coriolis force!

I now call that which is taken into account in meteorology 'rotational dynamics'. In retrospect, a name such as 'Ferrel-effect', to distinguish it from coriolis effect would have been fitting.

Inertial wind should not be confused with wind patterns that occur in the presence of pressure gradients. The very definition of 'inertial wind' is that there is no pressure gradient

Inertial wind is rare, but it does occur. On the northern hemisphere, inertial wind flows clockwise. Probably, inertial wind is a wind pattern that lingers on after a high pressure area has leveled out. So you must stop thinking in terms of pressure gradient in the case of inertial wind.

The difference between inertial wind and a ballistic trajectory is this: when a ballistic trajectory starts in east-to-west direction with respect to the Earth, starting at, say, 45 degrees latitude, then no matter how large or small the horizontal component of the ballistic missile's velocity, it will never go further away from the equator than 45 degrees latitude. (It is like a satellite orbit with such a tilt with respect to the Earth's equator that it's highest latitude is 45 degrees, northern and southern hemisphere, (for that is symmetric for a satellite orbit)) On the other hand: inertial oscillation in the atmosphere that is at some point flowing in east-to-west direction is deflecting to the North.

You are confused in thinking that anomaly can be solved by tweaking the velocity.

You can make the velocity component (of the ballistic missile) parallel to the surface very small by making the velocity component perpendicular to the surface very high. No matter how small the velocity component of the missile parallel to the surface, if launched in east-to-west direction, it will continue towards the equator.

Inertial wind is not spiralling towards or from, something. Friction eventually dissipates the energy of inertial wind, but the wind does not move up or down a pressure gradient, for there is no presure gradient

William M Connolley now believes that the MIT demonstrations with a parabolic rotating turntable provide a good model of inertial oscillations. The single dry ice puck represents the motion of a balloon, being swept along with inertial wind. If there would be a thin fluid layer on the parabolic rotating turntable then an eddy can be set in motion in that fluid that is an inertial oscillation. When an eddy is flowing in such an inertial oscillation then there is no water level difference, no water level gradient.

William M Connolley now believes that gravity must be present in order for the meteorological-coriolis-effect (Ferrel-effect) to occur at all.

WMC has written:

If the turntable has a bowl shape, then the component of gravity tangential to the bowl surface will tend to counteract the centrifugal force.

Conclusion: William now believes that a component of gravity must be acting as a centripetal force in order for the meteorological-coriolis-effect (Ferrel-effect) to occur. The earth's gravitation, redirected by the slope of the surface, provides this centripetal force.
--Cleon Teunissen | Talk 04:27, 23 August 2005 (UTC)

CT, this is hopeless. I really resent you putting words into my mouth when you don't understand what is going on. The article clearly states that the force balance in inertial circles, and in lows, is different. This is why they go different ways round. Its not because the coriolis effect is different, its because the other force - centrifugal, or pressure gradient - is different. You remain confused by the turntable: as it clearly states there, the gravitational component is being used to balance the centrifugal (err, and notice this is centrifugal-from-the-axis-of-rotation, not centrifugal-from-the-inertial-circle-centre) force to allow the coriolis dynamics to be seen. In the atmos, the same term does not appear. And *please* give up this meteorological-coriolis-effect (Ferrel-effect). There is one and only one coriolis effect, and starting a one man campaign to rename it will not work. William M. Connolley 08:24:15, 2005-08-23 (UTC).

Confusion (2)

[...] In the northern hemisphere the floating balloon circles to the left whereas a ballistic missile turns to the right.[...] Paul Beardsell 03:35, 23 August 2005 (UTC)

A balloon on the northern hemisphere that is being swept along with inertial wind is at all times being deflected to the right. On the northern hemisphere, inertial wind flows clockwise. On the northern hemisphere, cyclonic flow flows counter-clockwise. (Cyclonic flow is flow around a low-pressure area, the pressure gradient force deflecting the wind towards lowest pressure.)

Cyclonic flow occurs most often, inertial wind is rare, but inertial wind is interesting because it represents the most basic case.

On the Northern hemisphere, if a ballistic missile is launched in east-to-west direction then as seen by an observer who is stationary with respect to the Earth the ballistic missile appears to deflect somewhat to its right, but this deflection to the right is smaller than the overall motion of the ballistic missile towards the equator.

No matter what the velocity of the ballistic projectile is, no matter how slow: if it is launched without a north-directed velocity component then it will not land further north than the latitude of the launch platform.

At 45 degrees latitude, the tangential velocity corresponding to co-rotating with the Earth is about 330 meters per second. If the ballistic missile is launched with a east-to-west velocity with respect to the launching platform of 330 meters per second, then the apparent deflection of the ballistic missile (as seen by an observer who is stationary with respect to the Earth) is the strongest, and the missile will land on about the same latitude as where it was launched from. For all other velocities higher or lower than 330 meters per second with respect to the launching platform, the apparent deflection to the right will be less, and the missile will land closer to the equator than the latitude of the launching platform.
--Cleon Teunissen | Talk 05:52, 23 August 2005 (UTC)

Just as a step toward clarification, all these present examples are assuming the missile is launched in the plane of the great circle, right? Not, say, in the plane of the latitude line. GangofOne 06:30, 23 August 2005 (UTC)

Yes, launched in the plane of the great circle, not launched in the plane of the latitude circle. --Cleon Teunissen | Talk 06:46, 23 August 2005 (UTC)

Ballistic trajectories and great circles

Satellite orbits and ballistic trajectories are part of one and the same group of trajectories.

Satellite orbits can be circular or elliptical, if the satellite orbit is elliptical then the Earth is at one focus of the ellipse.

The parabolic trajectory of the vomit comet during weightlessness creating flight is in actual fact an elliptical trajectory with the Earth at one focus of the ellipse. In the case of the vomit comet the elliptical trajectory is smaller than the radius of the Earth, so the duration of weighlessness is limited, but during the period of weightlessness the mechanics for the people inside the airplane is the same as for astronauts on a space station: the orbital mechanics of satellite orbit.

A satellite orbit is always planar, and the center of gravitation of the Earth is in the plane of the orbit. If you intersect the plane of the satellite orbit with the surface of the Earth you get a great circle.

If you have a circular satellite orbit, tilted with respect to the Earth's equator, then as seen by an observer who is stationary with respect to the Earth, the satellite is oscillating, with the equator as the midpoint. As seen from Earth the satellite is from time to time moving parallel to a latitude line. Motion parallel to a latitude line, either east-to-west or west-to-east, is always followed by moving towards the equator, to the other hemisphere. Any ballistic trajectory has the property that when it is projected on the surface of the Earth, the projected line is a section of a great circle --Cleon Teunissen | Talk 06:34, 23 August 2005 (UTC)

The basics of meterology

Adding the Coriolis effect prolongs the duration of the low pressure region and shortens the duration of high pressure region. Paul Beardsell 03:35, 23 August 2005 (UTC)

This statement is incorrect.

On the northern hemisphere, flow around a low pressure area is counter-clockwise, flow around a high pressure area is clockwise. The meteorological-coriolis-effect (Ferrel-effect) prolongs the duration of both low-pressure areas and high pressure areas in equal measure.

It's pretty clear now that you are unfamiliar with even the most basic concepts of meteorology. --Cleon Teunissen | Talk 07:23, 23 August 2005 (UTC)