Catalan surface

This article is about the ruled surfaces. For the minimal surface, see Catalan's minimal surface.

A Catalan surface.

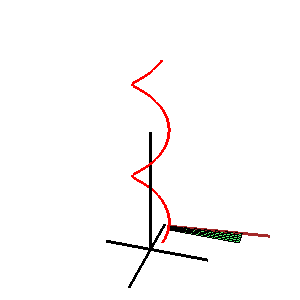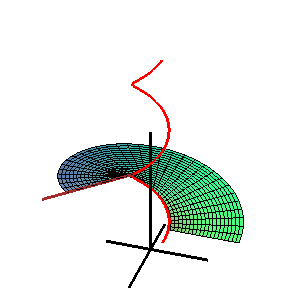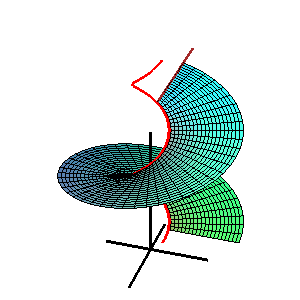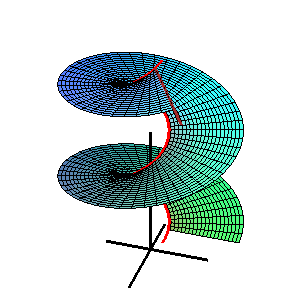
In geometry, a Catalan surface, named after the Belgian mathematician Eugène Charles Catalan, is a ruled surface all of whose generators are parallel to a fixed plane.

Equations

The vector equation of a Catalan surface is given by

r = s(u) + v L(u),

where r = s(u) is the space curve and L(u) is the unit vector of the ruling at u = u. All the vectors L(u) are parallel to the same plane, called the directrix plane of the surface. This can be characterized by the condition: the mixed product [L(u), L' (u), L" (u)] = 0.[1]

The parametric equations of the Catalan surface are [2]

$x=f(u)+vi(u),\quad y=g(u)+vj(u),\quad z=h(u)+vk(u)\,$

Special cases

If all the generators of a Catalan surface intersect a fixed line, then the surface is called a conoid.

Catalan proved that the helicoid and the plane were the only ruled minimal surfaces.

See also

• Generalized helicoid

References

• A. Gray, E. Abbena, S. Salamon, Modern differential geometry of curves and surfaces with Mathematica, 3rd ed. Boca Raton, Florida:CRC Press, 2006. [3] (ISBN 978-1-58488-448-4)
• "Catalan surface", Encyclopedia of Mathematics, EMS Press, 2001 [1994]
• V. Y. Rovenskii, Geometry of curves and surfaces with MAPLE [4] (ISBN 978-0-8176-4074-3)