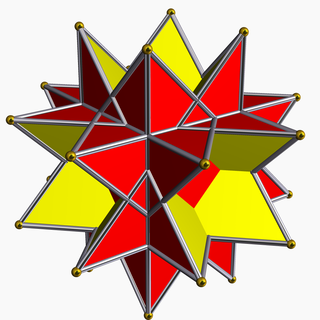
Stellated truncated hexahedron
| Stellated truncated hexahedron | |
|---|---|
 |
|
| Type | Uniform star polyhedron |
| Elements | F = 14, E = 36 V = 24 (χ = 2) |
| Faces by sides | 8{3}+6{8/3} |
| Wythoff symbol | 2 3 | 4/3 |
| Symmetry group | Oh, [4,3], *432 |
| Index references | U19, C66, W92 |
| Bowers acronym | Quith |
 3.8/3.8/3 (Vertex figure) |
 Great triakis octahedron (dual polyhedron) |
In geometry, the stellated truncated hexahedron (or quasitruncated hexahedron) is a uniform star polyhedron, indexed as U19. It is represented by Schläfli symbol t0,1{4/3,3}, and Coxeter-Dynkin diagram, 
. It is sometimes called quasitruncated hexahedron because it is related to the truncated cube, , except that the square faces become inverted into {8/3} octagrams.
The Stellated truncated hexahedron has 24 vertices, 36 edges, and 14 faces (8{3}+6{8/3}). The vertex configuration is 3.8/3.8/3. Its symmetry group is Oh, [4,3], *432, its Wythoff symbol is 2 3 | 4/3, and its Euler characteristic is χ=2.
Its uniform index number is U19, its Kaleido index is K24, its number in Wenninger's Polyhedron Models is 92, and it was given the number 66 in Coxeter's 1954 paper, which first gave the complete list of the uniform polyhedra.
Note that stellated truncated hexahedron is not a true stellation of the truncated hexahedron; its convex 'core' is nonuniform.
[edit] Related polyhedra
It shares the vertex arrangement with three other uniform polyhedra: the convex rhombicuboctahedron, the small rhombihexahedron, and the small cubicuboctahedron.
 Rhombicuboctahedron |
 Small cubicuboctahedron |
 Small rhombihexahedron |
Stellated truncated hexahedron |
[edit] See also
[edit] External links
 |
This polyhedron-related article is a stub. You can help Wikipedia by expanding it. |
