Jump to content

Chronology of computation of π

From Wikipedia, the free encyclopedia

This is an old revision of this page, as edited by InternetArchiveBot (talk | contribs) at 07:54, 24 November 2016 (Rescuing 1 sources and tagging 0 as dead. #IABot (v1.2.7.1)). The present address (URL) is a permanent link to this revision, which may differ significantly from the current revision.

The table below is a brief chronology of computed numerical values of, or bounds on, the mathematical constant pi (π). For more detailed explanations for some of these calculations, see Approximations of π.

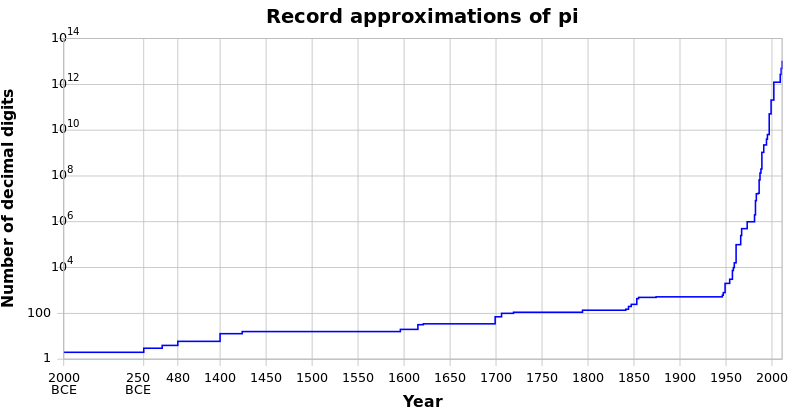
Graph showing how the record precision of numerical approximations to pi measured in decimal places (depicted on a logarithmic scale), evolved in human history. The time before 1400 is compressed.

Before 1400

Date Whom Formulation Value of pi Decimal places
(world records
in bold)
2000? BCЕ Ancient Egyptians[1] 4*(8/9)^2 3.16045... 1
2000? BCЕ Ancient Babylonians[1] 3+1/8 3.125 1
1200? BCЕ China[1] 3 1
550? BCЕ Bible (1 Kings 7:23)[1] "...a molten sea, ten cubits from the one brim to the other: it was round all about,... a line of thirty cubits did compass it round about" 3 1
434 BCE Anaxagoras attempted to square the circle[2] compass and straightedge Anaxagoras didn't offer any solution 0
350? BCЕ Sulbasutras[3][4] (6/(2+√2))^2 3.088311 … 1
c. 250 BCE Archimedes[1] 223/71 < π < 22/7 3.140845... < π < 3.142857...
3.1418 (ave.)		 3
15 BCE Vitruvius[3] 25/8 3.125 1
5 Liu Xin[3] the exact method is unknown 3.1457 2
130 Zhang Heng (Book of the Later Han)[1] √10 = 3.162277...
730/232 		3.146551... 1
150 Ptolemy[1] 377/120 3.141666... 3
250 Wang Fan[1] 142/45 3.155555... 1
263 Liu Hui[1] 3.141024 < π < 3.142074
3927/1250 		3.14159 5
400 He Chengtian[3] 111035/35329 3.142885... 2
480 Zu Chongzhi[1] 3.1415926 < π < 3.1415927
Zu's ratio 355/113 		3.1415926 7
499 Aryabhata[1] 62832/20000 3.1416 4
640 Brahmagupta[1] √10 3.162277... 1
800 Al Khwarizmi[1] 3.1416 4
1150 Bhāskara II[3] 3927/1250 and 754/240 3,1416 3
1220 Fibonacci[1] 3.141818 3
1320 Zhao Youqin[3] 3.1415926 7

From 1400 onwards

Date Whom Note Decimal places
(world records in bold)
All records from 1400 onwards are given as the number of correct decimal places.
1400 Madhava of Sangamagrama Probably discovered the infinite power series expansion of π,
now known as the Leibniz formula for pi[5] 		10
1424 Jamshīd al-Kāshī[6] 17
1573 Valentinus Otho 355/113 6
1579 François Viète[7] 9
1593 Adriaan van Roomen[8] 15
1596 Ludolph van Ceulen 20
1615 32
1621 Willebrord Snell (Snellius) Pupil of Van Ceulen 35
1630 Christoph Grienberger[9][10] 38
1665 Isaac Newton[1] 16
1681 Takakazu Seki[11] 11
16
1699 Abraham Sharp[1] Calculated pi to 72 digits, but not all were correct 71
1706 John Machin[1] 100
1706 William Jones Introduced the Greek letter 'π'
1719 Thomas Fantet de Lagny[1] Calculated 127 decimal places, but not all were correct 112
1722 Toshikiyo Kamata 24
1722 Katahiro Takebe 41
1739 Yoshisuke Matsunaga 51
1748 Leonhard Euler Used the Greek letter 'π' in his book Introductio in Analysin Infinitorum and assured its popularity.
1761 Johann Heinrich Lambert Proved that π is irrational
1775 Euler Pointed out the possibility that π might be transcendental
1789 Jurij Vega Calculated 143 decimal places, but not all were correct 126
1794 Jurij Vega[1] Calculated 140 decimal places, but not all were correct 136
1794 Adrien-Marie Legendre Showed that π² (and hence π) is irrational, and mentioned the possibility that π might be transcendental.
Late 18th century Anonymous manuscript Turns up at Radcliffe Library, in Oxford, England, discovered by F. X. von Zach, giving the value of pi to 154 digits, 152 of which were correct 152
1841 William Rutherford[1] Calculated 208 decimal places, but not all were correct 152
1844 Zacharias Dase and Strassnitzky[1] Calculated 205 decimal places, but not all were correct 200
1847 Thomas Clausen[1] Calculated 250 decimal places, but not all were correct 248
1853 Lehmann[1] 261
1855 Richter 500
1874 William Shanks[1] Took 15 years to calculate 707 decimal places, but not all were correct (the error was found by D. F. Ferguson in 1946) 527
1882 Ferdinand von Lindemann Proved that π is transcendental (the Lindemann–Weierstrass theorem)
1897 The U.S. state of Indiana Came close to legislating the value 3.2 (among others) for π. House Bill No. 246 passed unanimously. The bill stalled in the state Senate due to a suggestion of possible commercial motives involving publication of a textbook.[12] 1
1910 Srinivasa Ramanujan Found several rapidly converging infinite series of π, which can compute 8 decimal places of π with each term in the series. Since the 1980s, his series have become the basis for the fastest algorithms currently used by Yasumasa Kanada and the Chudnovsky brothers to compute π.
1946 D. F. Ferguson Desk calculator 620
1947 Ivan Niven Gave a very elementary proof that π is irrational
January 1947 D. F. Ferguson Desk calculator 710
September 1947 D. F. Ferguson Desk calculator 808
1949 D. F. Ferguson and John Wrench Desk calculator 1,120

The age of electronic computers (from 1949 onwards)

Date Whom Implementation Time Decimal places
(world records in bold)
All records from 1949 onwards were calculated with electronic computers.
1949 John Wrench, and L. R. Smith Were the first to use an electronic computer (the ENIAC) to calculate π (also attributed to Reitwiesner et al.) [13] 70 hours 2,037
1953 Kurt Mahler Showed that π is not a Liouville number
1954 S. C. Nicholson & J. Jeenel Using the NORC [14] 13 minutes 3,093
1957 George E. Felton Ferranti Pegasus computer (London), calculated 10,021 digits, but not all were correct [15] 7,480
January 1958 Francois Genuys IBM 704 [16] 1.7 hours 10,000
May 1958 George E. Felton Pegasus computer (London) 33 hours 10,021
1959 Francois Genuys IBM 704 (Paris)[17] 4.3 hours 16,167
1961 Daniel Shanks and John Wrench IBM 7090 (New York)[18] 8.7 hours 100,265
1961 J.M. Gerard IBM 7090 (London) 39 minutes 20,000
1966 Jean Guilloud and J. Filliatre IBM 7030 (Paris) 28 hours {?) 250,000
1967 Jean Guilloud and M. Dichampt CDC 6600 (Paris) 28 hours 500,000
1973 Jean Guilloud and Martin Bouyer CDC 7600 23.3 hours 1,001,250
1981 Kazunori Miyoshi and Yasumasa Kanada FACOM M-200 2,000,036
1981 Jean Guilloud Not known 2,000,050
1982 Yoshiaki Tamura MELCOM 900II 2,097,144
1982 Yoshiaki Tamura and Yasumasa Kanada HITAC M-280H 2.9 hours 4,194,288
1982 Yoshiaki Tamura and Yasumasa Kanada HITAC M-280H 8,388,576
1983 Yasumasa Kanada, Sayaka Yoshino and Yoshiaki Tamura HITAC M-280H 16,777,206
October 1983 Yasunori Ushiro and Yasumasa Kanada HITAC S-810/20 10,013,395
October 1985 Bill Gosper Symbolics 3670 17,526,200
January 1986 David H. Bailey CRAY-2 29,360,111
September 1986 Yasumasa Kanada, Yoshiaki Tamura HITAC S-810/20 33,554,414
October 1986 Yasumasa Kanada, Yoshiaki Tamura HITAC S-810/20 67,108,839
January 1987 Yasumasa Kanada, Yoshiaki Tamura, Yoshinobu Kubo and others NEC SX-2 134,214,700
January 1988 Yasumasa Kanada and Yoshiaki Tamura HITAC S-820/80 201,326,551
May 1989 Gregory V. Chudnovsky & David V. Chudnovsky CRAY-2 & IBM 3090/VF 480,000,000
June 1989 Gregory V. Chudnovsky & David V. Chudnovsky IBM 3090 535,339,270
July 1989 Yasumasa Kanada and Yoshiaki Tamura HITAC S-820/80 536,870,898
August 1989 Gregory V. Chudnovsky & David V. Chudnovsky IBM 3090 1,011,196,691
19 November 1989 Yasumasa Kanada and Yoshiaki Tamura HITAC S-820/80 1,073,740,799
August 1991 Gregory V. Chudnovsky & David V. Chudnovsky Homemade parallel computer (details unknown, not verified) [19] 2,260,000,000
18 May 1994 Gregory V. Chudnovsky & David V. Chudnovsky New homemade parallel computer (details unknown, not verified) 4,044,000,000
26 June 1995 Yasumasa Kanada and Daisuke Takahashi HITAC S-3800/480 (dual CPU) [20] 3,221,220,000
1995 Simon Plouffe Finds a formula that allows the nth digit of pi to be calculated without calculating the preceding digits.
28 August 1995 Yasumasa Kanada and Daisuke Takahashi HITAC S-3800/480 (dual CPU) [21] 4,294,960,000
11 October 1995 Yasumasa Kanada and Daisuke Takahashi HITAC S-3800/480 (dual CPU) [22] 6,442,450,000
6 July 1997 Yasumasa Kanada and Daisuke Takahashi HITACHI SR2201 (1024 CPU) [23] 51,539,600,000
5 April 1999 Yasumasa Kanada and Daisuke Takahashi HITACHI SR8000 (64 of 128 nodes) [24] 68,719,470,000
20 September 1999 Yasumasa Kanada and Daisuke Takahashi HITACHI SR8000/MPP (128 nodes) [25] 206,158,430,000
24 November 2002 Yasumasa Kanada & 9 man team HITACHI SR8000/MPP (64 nodes), Department of Information Science at the University of Tokyo in Tokyo, Japan [26] 600 hours 1,241,100,000,000
29 April 2009 Daisuke Takahashi et al. T2K Open Supercomputer (640 nodes), single node speed is 147.2 gigaflops, computer memory is 13.5 terabytes, Gauss–Legendre algorithm, Center for Computational Sciences at the University of Tsukuba in Tsukuba, Japan[27] 29.09 hours 2,576,980,377,524
Date Whom Implementation Time Decimal places
(world records in bold)
All records from Dec 2009 onwards are calculated on home computers with commercially available parts.
31 December 2009 Fabrice Bellard
  • Core i7 CPU at 2.93 GHz
  • 6 GiB (1) of RAM
  • 7.5 TB of disk storage using five 1.5 TB hard disks (Seagate Barracuda 7200.11 model)
  • 64 bit Red Hat Fedora 10 distribution
  • Computation of the binary digits: 103 days
  • Verification of the binary digits: 13 days
  • Conversion to base 10: 12 days
  • Verification of the conversion: 3 days
  • Verification of the binary digits used a network of 9 Desktop PCs during 34 hours, Chudnovsky algorithm, see [28] for Bellard's homepage.[29]
 131 days 2,699,999,990,000
2 August 2010 Shigeru Kondo[30]
  • using y-cruncher[31] by Alexander Yee
  • the Chudnovsky formula was used for main computation
  • verification used the Bellard & Plouffe formulas on different computers, both computed 32 hexadecimal digits ending with the 4,152,410,118,610th.
  • with 2 x Intel Xeon X5680 @ 3.33 GHz – (12 physical cores, 24 hyperthreaded)
  • 96 GB DDR3 @ 1066 MHz – (12 × 8 GB – 6 channels) – Samsung (M393B1K70BH1)
  • 1 TB SATA II (Boot drive) – Hitachi (HDS721010CLA332), 3 × 2 TB SATA II (Store Pi Output) – Seagate (ST32000542AS) 16 x 2 TB SATA II (Computation) – Seagate (ST32000641AS)
  • Windows Server 2008 R2 Enterprise x64
  • Computation of binary digits: 80 days
  • Conversion to base 10: 8.2 days
  • Verification of the conversion: 45.6 hours
  • Verification of the binary digits: 64 hours (primary), 66 hours (secondary)
  • Verification of the binary digits were done simultaneously on two separate computers during the main computation.[32]
 90 days 5,000,000,000,000
17 October 2011 Shigeru Kondo[33]
  • using y-cruncher by Alexander Yee
  • Verification: 1.86 days and 4.94 days
 371 days 10,000,000,000,050
28 December 2013 Shigeru Kondo[34]
  • using y-cruncher by Alexander Yee
  • with 2 x Intel Xeon E5-2690 @ 2.9 GHz - (16 physical cores, 32 hyperthreaded)
  • 128 GB DDR3 @ 1600 MHz - 8 x 16 GB - 8 channels
  • Windows Server 2012 x64
  • Verification: 46 hours
 94 days 12,100,000,000,050
8 October 2014 "houkouonchi"[35]
  • using y-cruncher by Alexander Yee
  • with 2 x Xeon E5-4650L @ 2.6 GHz
  • 192 GB DDR3 @ 1333 MHz
  • 24 x 4 TB + 30 x 3 TB
  • Verification: 182 hours
 208 days 13,300,000,000,000
11 November 2016 Peter Trueb[36][37]
  • using y-cruncher by Alexander Yee
  • with 4 x Xeon E7-8890 v3 @ 2.50 GHz (72 cores, 144 threads)
  • 1.25 TB DDR4
  • 20 x 6 TB
  • Verification: 28 hours[38]
 105 days 22,459,157,718,361[39]

See also

Part of a series of articles on the
mathematical constant π
3.1415926535897932384626433...
Uses
Properties
Value
People
History
In culture
Related topics


References

  1. ^ a b c d e f g h i j k l m n o p q r s t u v w x "The quest for pi" (PDF). Mathematical Intelligencer. 19 (1): 50–57. 1997. {{cite journal}}: Unknown parameter |authors= ignored (help)
  2. ^ https://www.math.rutgers.edu/~cherlin/History/Papers2000/wilson.html
  3. ^ a b c d e f "Birth, growth and computation of pi to ten trillion digits". Advances in Difference Equations. 2013: 100. 2013. doi:10.1186/1687-1847-2013-100. {{cite journal}}: Unknown parameter |authors= ignored (help)CS1 maint: unflagged free DOI (link)
  4. ^ https://books.google.com/books?id=DHvThPNp9yMC&lpg=PA18&ots=Aoy0T2r3Qz&dq=Shulba%20Sutras%20date%20of%20creation&hl=de&pg=PA18#v=onepage&q=Shulba%20Sutras%20date%20of%20creation&f=false
  5. ^ Bag, A. K. (1980). "Indian Literature on Mathematics During 1400–1800 A.D." (PDF). Indian Journal of History of Science. 15 (1): 86. π ≈ 2,827,433,388,233/9×10−11 = 3.14159 26535 92222…, good to 10 decimal places.
  6. ^ approximated 2π to 9 sexagesimal digits. Al-Kashi, author: Adolf P. Youschkevitch, chief editor: Boris A. Rosenfeld, p. 256 O'Connor, John J.; Robertson, Edmund F., "Ghiyath al-Din Jamshid Mas'ud al-Kashi", MacTutor History of Mathematics Archive, University of St Andrews. Azarian, Mohammad K. (2010), "al-Risāla al-muhītīyya: A Summary", Missouri Journal of Mathematical Sciences 22 (2): 64–85.
  7. ^ Viète, François (1579). Canon mathematicus seu ad triangula : cum adpendicibus (in Latin).
  8. ^ Romanus, Adrianus (1593). Ideae mathematicae pars prima, sive methodus polygonorum (in Latin).
  9. ^ Grienbergerus, Christophorus (1630). Elementa Trigonometrica (PDF) (in Latin).
  10. ^ Hobson, Ernest William (1913). "Squaring the Circle": a History of the Problem (PDF). p. 27.
  11. ^ Yoshio, Mikami; Eugene Smith, David (April 2004) [January 1914]. A History of Japanese Mathematics (paperback ed.). Dover Publications. ISBN 0-486-43482-6.
  12. ^ Lopez-Ortiz, Alex (February 20, 1998). "Indiana Bill sets value of Pi to 3". the news.answers WWW archive. Department of Information and Computing Sciences, Utrecht University. Retrieved 2009-02-01.
  13. ^ G. Reitwiesner, "An ENIAC determination of Pi and e to more than 2000 decimal places," MTAC, v. 4, 1950, pp. 11–15"
  14. ^ S. C, Nicholson & J. Jeenel, "Some comments on a NORC computation of x," MTAC, v. 9, 1955, pp. 162–164
  15. ^ G. E. Felton, "Electronic computers and mathematicians," Abbreviated Proceedings of the Oxford Mathematical Conference for Schoolteachers and Industrialists at Trinity College, Oxford, April 8–18, 1957, pp. 12–17, footnote pp. 12–53. This published result is correct to only 7480D, as was established by Felton in a second calculation, using formula (5), completed in 1958 but apparently unpublished. For a detailed account of calculations of x see J. W. Wrench, Jr., "The evolution of extended decimal approximations to x," The Mathematics Teacher, v. 53, 1960, pp. 644–650
  16. ^ F. Genuys, "Dix milles decimales de x," Chiffres, v. 1, 1958, pp. 17–22.
  17. ^ This unpublished value of x to 16167D was computed on an IBM 704 system at the Commissariat à l'Energie Atomique in Paris, by means of the program of Genuys
  18. ^ [1] "Calculation of Pi to 100,000 Decimals" in the journal Mathematics of Computation, vol 16 (1962), issue 77, pages 76–99.
  19. ^ Bigger slices of Pi (determination of the numerical value of pi reaches 2.16 billion decimal digits) Science News 24 August 1991 http://www.encyclopedia.com/doc/1G1-11235156.html
  20. ^ ftp://pi.super-computing.org/README.our_last_record_3b
  21. ^ ftp://pi.super-computing.org/README.our_last_record_4b
  22. ^ ftp://pi.super-computing.org/README.our_last_record_6b
  23. ^ ftp://pi.super-computing.org/README.our_last_record_51b
  24. ^ ftp://pi.super-computing.org/README.our_last_record_68b
  25. ^ ftp://pi.super-computing.org/README.our_latest_record_206b
  26. ^ http://www.super-computing.org/pi_current.html
  27. ^ http://www.hpcs.is.tsukuba.ac.jp/~daisuke/pi.html
  28. ^ "Fabrice Bellard's Home Page". bellard.org. Retrieved 28 August 2015.
  29. ^ http://bellard.org/pi/pi2700e9/pipcrecord.pdf
  30. ^ "PI-world". calico.jp. Retrieved 28 August 2015.
  31. ^ "y-cruncher - A Multi-Threaded Pi Program". numberworld.org. Retrieved 28 August 2015.
  32. ^ "Pi - 5 Trillion Digits". numberworld.org. Retrieved 28 August 2015.
  33. ^ "Pi - 10 Trillion Digits". numberworld.org. Retrieved 28 August 2015.
  34. ^ "Pi - 12.1 Trillion Digits". numberworld.org. Retrieved 28 August 2015.
  35. ^ "y-cruncher - A Multi-Threaded Pi Program". numberworld.org. Retrieved 28 August 2015.
  36. ^ "pi2e". pi2e.ch. Retrieved 15 November 2016.
  37. ^ "y-cruncher - A Multi-Threaded Pi Program". numberworld.org. Retrieved 15 November 2016.
  38. ^ "Hexadecimal Digits are Correct! - pi2e trillion digits of pi". pi2e.ch. Retrieved 15 November 2016.
  39. ^ 22,459,157,718,361 is πe*1012 rounded down.
Retrieved from "https://en.wikipedia.org/w/index.php?title=Chronology_of_computation_of_π&oldid=751240200"