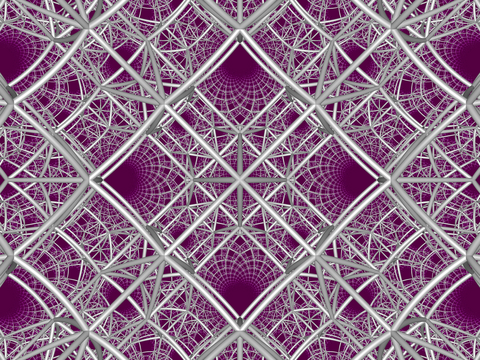
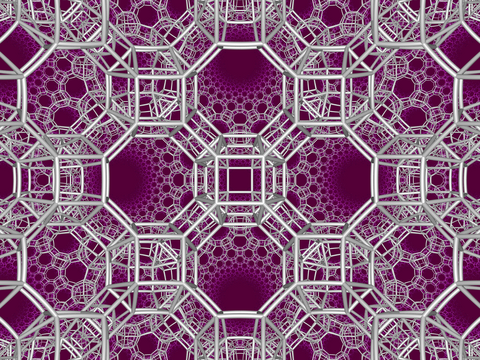
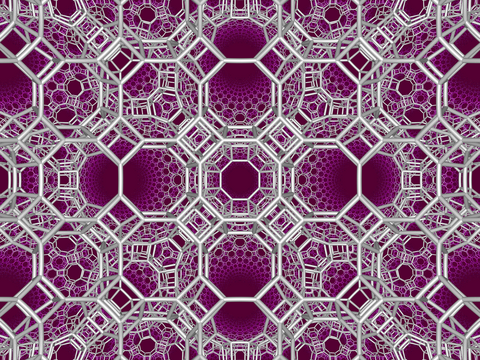
Square tiling honeycomb
| Square tiling honeycomb | |
|---|---|
| |
| Type | Hyperbolic regular honeycomb Paracompact uniform honeycomb |
| Schläfli symbols | {4,4,3} r{4,4,4} {41,1,1} |
| Coxeter diagrams |       ↔      ↔    ↔  
|
| Cells | {4,4}   
|
| Faces | Square {4} |
| Edge figure | Triangle {3} |
| Vertex figure |  cube, {4,3} |
| Dual | Order-4 octahedral honeycomb |
| Coxeter groups | [4,4,3] [41,1,1] ↔ [4,4,3*] |
| Properties | Regular |
In the geometry of hyperbolic 3-space, the square tiling honeycomb, is one of 11 paracompact regular honeycombs. It is called paracompact because it has infinite cells, whose vertices exist on horospheres and converge to a single ideal point at infinity. Given by Schläfli symbol {4,4,3}, has three square tilings, {4,4} around each edge, and 6 square tilings around each vertex in an cubic {4,3} vertex figure.[1]
A geometric honeycomb is a space-filling of polyhedral or higher-dimensional cells, so that there are no gaps. It is an example of the more general mathematical tiling or tessellation in any number of dimensions.
Honeycombs are usually constructed in ordinary Euclidean ("flat") space, like the convex uniform honeycombs. They may also be constructed in non-Euclidean spaces, such as hyperbolic uniform honeycombs. Any finite uniform polytope can be projected to its circumsphere to form a uniform honeycomb in spherical space.
Rectified order-4 square tiling
It is also seen as a rectified order-4 square tiling honeycomb, r{4,4,4}:
| {4,4,4} | r{4,4,4} = {4,4,3} |
|---|---|
|
=
|
 |
|
Symmetry
It has three reflective symmetry constructions, as a regular honeycomb, a half symmetry ↔ and lastly ↔ with 3 types (colors) of checkered square tilings. [4,4,3*] ↔ [41,1,1], index 6, and a final radial subgroup [4,(4,3)*], index 48, with a right dihedral angled octahedral fundamental domain, and 4 pairs of ultraparallel mirrors: .
This honeycomb contains 
that tile 2-hypercycle surfaces, similar to this paracompact tiling, 
:
Related polytopes and honeycombs
It is one of 15 regular hyperbolic honeycombs in 3-space, 11 of which like this one are paracompact, with infinite cells or vertex figures.
| 11 paracompact regular honeycombs | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
 {6,3,3} |
 {6,3,4} |
 {6,3,5} |
 {6,3,6} |
{4,4,3} |
{4,4,4} | ||||||
 {3,3,6} |
 {4,3,6} |
 {5,3,6} |
 {3,6,3} |
 {3,4,4} | |||||||
There are fifteen uniform honeycombs in the [4,4,3] Coxeter group family, including this regular form, and its dual, the order-4 octahedral honeycomb, {3,4,4}.
| {4,4,3}
|
r{4,4,3}
|
t{4,4,3}
|
rr{4,4,3}
|
t0,3{4,4,3}
|
tr{4,4,3}
|
t0,1,3{4,4,3}
|
t0,1,2,3{4,4,3}
|
|---|---|---|---|---|---|---|---|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
| {3,4,4}
|
r{3,4,4}
|
t{3,4,4}
|
rr{3,4,4}
|
2t{3,4,4}
|
tr{3,4,4}
|
t0,1,3{3,4,4}
|
t0,1,2,3{3,4,4}
|
| [4,4,4] family honeycombs | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| {4,4,4}
|
r{4,4,4}
|
t{4,4,4}
|
rr{4,4,4}
|
t0,3{4,4,4}
|
2t{4,4,4}
|
tr{4,4,4}
|
t0,1,3{4,4,4}
|
t0,1,2,3{4,4,4}
| |||
|
|
|
|
|
|
|
|
|
| |||
This honeycomb is related to the 24-cell, {3,4,3}, with a cubic vertex figure.
It is a part of a sequence of honeycombs with square tiling cells:
| {4,4,p} honeycombs | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Space | E3 | H3 | |||||||||
| Form | Affine | Paracompact | Noncompact | ||||||||
| Name | {4,4,2} | {4,4,3} | {4,4,4} | {4,4,5} | {4,4,6} | ...{4,4,∞} | |||||
Coxeter
|
|
|
|
 
|
   
|
  
| |||||
| Image |
|
|
|
|
| ||||||
| Vertex figure |
 {4,2}
|
 {4,3}
|
{4,4}
|
 {4,5}
|
 {4,6}
|
 {4,∞}
| |||||
Rectified square tiling honeycomb
| Rectified square tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb Semiregular honeycomb |
| Schläfli symbols | r{4,4,3} or t1{4,4,3} 2r{3,41,1} r{41,1,1} |
| Coxeter diagrams | ↔ ↔   ↔
|
| Cells | {4,3}  r{4,4} 
|
| Faces | square {4} |
| Vertex figure | 
|
| Coxeter groups | [4,4,3] [41,1,1] ↔ [4,4,3*] |
| Properties | Vertex-transitive, edge-transitive |
The rectified square tiling honeycomb, t1{4,4,3}, has cube and square tiling facets, with a triangular prism vertex figure.
It is similar to the 2D hyperbolic uniform triapeirogonal tiling, r{∞,3}, with triangle and apeirogonal faces.
Truncated square tiling honeycomb
| Truncated square tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | t{4,4,3} or t0,1{4,4,3} |
| Coxeter diagrams | ↔ ↔
|
| Cells | {4,3} t{4,4} 
|
| Faces | square {4} octagon {8} |
| Vertex figure | 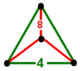 triangular pyramid |
| Coxeter groups | [4,4,3] |
| Properties | Vertex-transitive |
The truncated square tiling honeycomb, t{4,4,3}, has cube and truncated square tiling facets, with a triangular pyramid vertex figure.
Bitruncated square tiling honeycomb
| Bitruncated square tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | 2t{4,4,3} or t1,2{4,4,3} |
| Coxeter diagrams |
|
| Cells | t{4,3}  t{4,4}
|
| Faces | triangular {3} square {4} octagon {8} |
| Vertex figure |  digonal disphenoid |
| Coxeter groups | [4,4,3] |
| Properties | Vertex-transitive |
The bitruncated square tiling honeycomb, 2t{4,4,3}, has truncated cube and truncated square tiling facets, with a digonal disphenoid vertex figure.
Cantellated square tiling honeycomb
| Cantellated square tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | rr{4,4,3} or t0,2{4,4,3} |
| Coxeter diagrams | ↔
|
| Cells | r{4,3}  rr{4,4} 
|
| Faces | triangular {3} square {4} |
| Vertex figure | 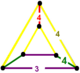 triangular prism |
| Coxeter groups | [4,4,3] |
| Properties | Vertex-transitive |
The cantitruncated square tiling honeycomb, rr{4,4,3}, has cube and truncated square tiling facets, with a triangular prism vertex figure.
Cantitruncated square tiling honeycomb
| Cantitruncated square tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | tr{4,4,3} or t0,1,2{4,4,3} |
| Coxeter diagrams |
|
| Cells | t{4,3} tr{4,4}  {}x{3} 
|
| Faces | triangular {3} square {4} octagon {8} |
| Vertex figure | 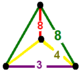 tetrahedron |
| Coxeter groups | [4,4,3] |
| Properties | Vertex-transitive |
The cantitruncated square tiling honeycomb, tr{4,4,3}, has truncated cube and truncated square tiling facets, with a tetrahedron vertex figure.
Runcinated square tiling honeycomb
| Runcinated square tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | t0,3{4,4,3} |
| Coxeter diagrams | ↔
|
| Cells | {3,4}  {4,4}  {}x{4} {}x{3}
|
| Faces | triangle {3} square {4} |
| Vertex figure |  triangular antiprism |
| Coxeter groups | [4,4,3] |
| Properties | Vertex-transitive |
The runcinated square tiling honeycomb, t0,3{4,4,3}, has octahedron, triangular prism, cube, and square tiling facets, with a triangular antiprism vertex figure.
Runcitruncated square tiling honeycomb
| Runcitruncated square tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | t0,1,3{4,4,3} s2,3{3,4,4} |
| Coxeter diagrams | 
|
| Cells | rr{4,3}  t{4,4} {}x{3} {}x{8} 
|
| Faces | triangle {3} square {4} |
| Vertex figure |  trapezoidal pyramid |
| Coxeter groups | [4,4,3] |
| Properties | Vertex-transitive |
The runcitruncated square tiling honeycomb, t0,1,3{4,4,3}, has rhombicuboctahedron, octagonal prism, triangular prism and truncated square tiling facets, with a trapezoidal pyramid vertex figure.
Omnitruncated square tiling honeycomb
| Omnitruncated square tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | t0,1,2,3{4,4,3} |
| Coxeter diagrams |
|
| Cells | {4,4} {}x{6}  {}x{8} tr{4,3} 
|
| Faces | Square {4} Hexagon {6} Octagon {8} |
| Vertex figure | 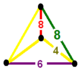 tetrahedron |
| Coxeter groups | [4,4,3] |
| Properties | Vertex-transitive |
The omnitruncated square tiling honeycomb, t0,1,2,3{4,4,3}, has truncated square tiling, truncated cuboctahedron, hexagonal prism, octagonal prism facets, with a tetrahedron vertex figure.
Omnisnub square tiling honeycomb
| Omnisnub square tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | h(t0,1,2,3{4,4,3}) |
| Coxeter diagrams |
|
| Cells | sr{4,4}  sr{2,3}  sr{2,4}  sr{4,3} 
|
| Faces | Triangular {3} Square {4} |
| Vertex figure | |
| Coxeter group | [4,4,3]+ |
| Properties | Vertex-transitive |
The alternated omnitruncated square tiling honeycomb (or omnisnub square tiling honeycomb), h(t0,1,2,3{4,4,3}), has snub square tiling, snub cube, triangular antiprism, square antiprism, and tetrahedron cells, with an irregular vertex figure.
Alternated square tiling honeycomb
| Alternated square tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb Semiregular honeycomb |
| Schläfli symbols | h{4,4,3} |
| Coxeter diagrams |  ↔  ↔  ↔   ↔ ↔
|
| Cells | (4.4.4.4) (4.4.4) |
| Faces | Square {4} |
| Vertex figure | (4.3.4.3) |
| Coxeter groups | [4,4,3] |
| Properties | vertex-transitive, edge-transitive, quasiregular |
The alternated square tiling honeycomb is a paracompact uniform honeycomb in hyperbolic 3-space, composed of cube, and square tiling facets in a cuboctahedron vertex figure.
Alternated rectified square tiling honeycomb
| Alternated rectified square tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | hr{4,4,3} |
| Coxeter diagrams | ↔
|
| Cells | |
| Faces | |
| Vertex figure | |
| Coxeter groups | [4,1+,4,3] = [∞,3,3,∞] |
| Properties | Vertex-transitive |
The alternated rectified square tiling honeycomb is a paracompact uniform honeycomb in hyperbolic 3-space.
See also
References
- ^ Coxeter The Beauty of Geometry, 1999, Chapter 10, Table III
- Coxeter, Regular Polytopes, 3rd. ed., Dover Publications, 1973. ISBN 0-486-61480-8. (Tables I and II: Regular polytopes and honeycombs, pp. 294–296)
- The Beauty of Geometry: Twelve Essays (1999), Dover Publications, LCCN 99-35678, ISBN 0-486-40919-8 (Chapter 10, Regular Honeycombs in Hyperbolic Space) Table III
- Jeffrey R. Weeks The Shape of Space, 2nd edition ISBN 0-8247-0709-5 (Chapter 16-17: Geometries on Three-manifolds I,II)
- Norman Johnson Uniform Polytopes, Manuscript
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. Dissertation, University of Toronto, 1966
- N.W. Johnson: Geometries and Transformations, (2015) Chapter 13: Hyperbolic Coxeter groups
- Norman W. Johnson and Asia Ivic Weiss Quadratic Integers and Coxeter Groups PDF Canad. J. Math. Vol. 51 (6), 1999 pp. 1307–1336