Chamfer (geometry)
In geometry, chamfering or edge-truncation is a topological operator that modifies one polyhedron into another. It is similar to expansion: it moves the faces apart (outward), and adds a new face between each two adjacent faces; but contrary to expansion, it maintains the original vertices. (Equivalently: it separates the faces by reducing them, and adds a new face between each two adjacent faces; but it only moves the vertices lower.) For a polyhedron, this operation adds a new hexagonal face in place of each original edge.
In Conway polyhedron notation, chamfering is represented by the letter "c". A polyhedron with e edges will have a chamfered form containing 2e new vertices, 3e new edges, and e new hexagonal faces.
Chamfered Platonic solids[edit]
In the chapters below, the chamfers of the five Platonic solids are described in detail. Each is shown in a version with edges of equal lengths, and in a canonical version where all edges touch the same midsphere. (They look noticeably different only for solids containing triangles.) The shown dual polyhedra are dual to the canonical versions.
| Seed Platonic solid |
  {3,3} |
 {4,3} |
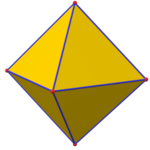 {3,4} |
 {5,3} |
 {3,5} |
|---|---|---|---|---|---|
| Chamfered Platonic solid |
 
|

|

|

|

|
Chamfered tetrahedron[edit]
| Chamfered tetrahedron | |
|---|---|
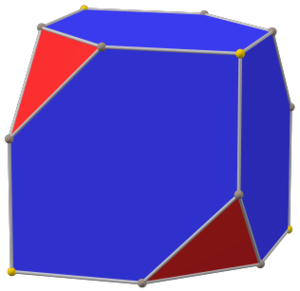 (equilateral-faced form) | |
| Conway notation | cT |
| Goldberg polyhedron | GPIII(2,0) = {3+,3}2,0 |
| Faces | 4 congruent equilateral triangles 6 congruent hexagons (equilateral for a certain chamfering depth) |
| Edges | 24 (2 types: triangle-hexagon, hexagon-hexagon) |
| Vertices | 16 (2 types) |
| Vertex configuration | (12) 3.6.6 (4) 6.6.6 |
| Symmetry group | Tetrahedral (Td) |
| Dual polyhedron | Alternate-triakis tetratetrahedron |
| Properties | convex, equilateral-faced (for a certain chamfering depth) |
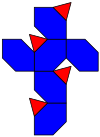 Net | |
The chamfered tetrahedron (or alternate truncated cube) is a convex polyhedron constructed by alternately truncating a cube, replacing 4 of its 8 vertices with congruent triangular faces, or by chamfering a regular tetrahedron, replacing its 6 edges with congruent flattened hexagons.
The chamfered tetrahedron is the Goldberg polyhedron GIII(2,0), containing triangular and hexagonal faces.


 chamfered tetrahedron (canonical form) |
 dual of the tetratetrahedron |
 chamfered tetrahedron (canonical form) |
 alternate-triakis tetratetrahedron |
 tetratetrahedron |
 alternate-triakis tetratetrahedron |
Chamfered cube[edit]
| Chamfered cube | |
|---|---|
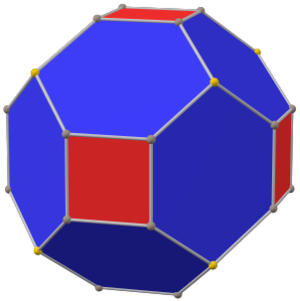 (equilateral-faced form) | |
| Conway notation | cC = t4daC |
| Goldberg polyhedron | GPIV(2,0) = {4+,3}2,0 |
| Faces | 6 congruent squares 12 congruent hexagons (equilateral for a certain chamfering depth) |
| Edges | 48 (2 types: square-hexagon, hexagon-hexagon) |
| Vertices | 32 (2 types) |
| Vertex configuration | (24) 4.6.6 (8) 6.6.6 |
| Symmetry | Oh, [4,3], (*432) Th, [4,3+], (3*2) |
| Dual polyhedron | Tetrakis cuboctahedron |
| Properties | convex, equilateral-faced (for a certain chamfering depth) |
 Net (3 zones are shown by 3 colors for their hexagons — each square is in 2 zones —.) | |
The chamfered cube is constructed as a chamfer of a cube: the squares are reduced in size and new hexagonal faces are added in place of all the original edges. The chamfered cube is a convex polyhedron with 32 vertices, 48 edges, and 18 faces: 6 congruent (and regular) squares, and 12 congruent flattened hexagons which are equilateral for a certain depth of chamfering. Its dual is the tetrakis cuboctahedron.
It is also inaccurately called a truncated rhombic dodecahedron, although that name rather suggests a rhombicuboctahedron. It can more accurately be called a tetratruncated rhombic dodecahedron, because only the (6) order-4 vertices of the rhombic dodecahedron are truncated.
The hexagonal faces are equilateral but not regular. They are congruent partly truncated rhombi, have 2 internal angles of and 4 internal angles of while a regular hexagon would have all internal angles.
Because all its faces have an even number of sides and are centrally symmetric, it is a zonohedron. It is also the Goldberg polyhedron GPIV(2,0) or {4+,3}2,0, containing square and hexagonal faces.
The chamfered cube is the Minkowski sum of a rhombic dodecahedron and a cube of side length 1 when the eight order-3 vertices of the rhombic dodecahedron are at and its six order-4 vertices are at the permutations of
A topological equivalent with pyritohedral symmetry and rectangular faces can be constructed by chamfering the axial edges of a pyritohedron. This occurs in pyrite crystals.
|
Pyritohedron and its axis truncation |
Historical crystallographic models of axis truncation and axis light truncation of pyritohedron |

 chamfered cube (canonical form) |
 rhombic dodecahedron |
 chamfered octahedron (canonical form) |
 tetrakis cuboctahedron |
 cuboctahedron |
 triakis cuboctahedron |
Chamfered octahedron[edit]
| Chamfered octahedron | |
|---|---|
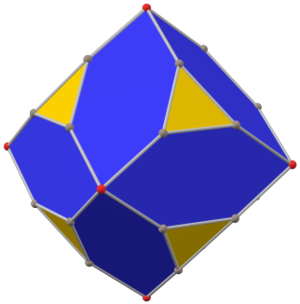 (equilateral-faced form) | |
| Conway notation | cO = t3daO |
| Faces | 8 congruent equilateral triangles 12 congruent hexagons (equilateral for a certain chamfering depth) |
| Edges | 48 (2 types: triangle-hexagon, hexagon-hexagon) |
| Vertices | 30 (2 types) |
| Vertex configuration | (24) 3.6.6 (6) 6.6.6.6 |
| Symmetry | Oh, [4,3], (*432) |
| Dual polyhedron | Triakis cuboctahedron |
| Properties | convex, equilateral (for a certain chamfering depth) |
In geometry, the chamfered octahedron (or tritruncated rhombic dodecahedron) is a convex polyhedron constructed by truncating the 8 order-3 vertices of the rhombic dodecahedron. These truncated vertices become congruent equilateral triangles, and the original 12 rhombic faces become congruent flattened hexagons.
For a certain depth of truncation, all final edges have same length; then, the hexagons are equilateral, but not regular).

Chamfered dodecahedron[edit]
| Chamfered dodecahedron | |
|---|---|
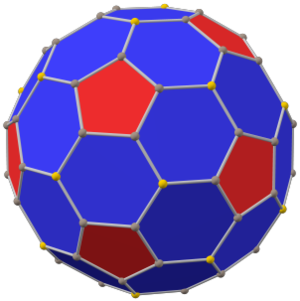 (with equal edge length) | |
| Conway notation | cD = t5daD = dk5aD |
| Goldberg polyhedron | GV(2,0) = {5+,3}2,0 |
| Fullerene | C80[2] |
| Faces | 12 pentagons 30 hexagons |
| Edges | 120 (2 types) |
| Vertices | 80 (2 types) |
| Vertex configuration | (60) 5.6.6 (20) 6.6.6 |
| Symmetry group | Icosahedral (Ih) |
| Dual polyhedron | Pentakis icosidodecahedron |
| Properties | convex, equilateral-faced |
The chamfered dodecahedron is a convex polyhedron with 80 vertices, 120 edges, and 42 faces: 30 hexagons and 12 pentagons. It is constructed as a chamfer of a regular dodecahedron. The pentagons are reduced in size and new hexagonal faces are added in place of all the original edges. Its dual is the pentakis icosidodecahedron.
It is also inaccurately called a truncated rhombic triacontahedron, although that name rather suggests a rhombicosidodecahedron. It can more accurately be called a pentatruncated rhombic triacontahedron because only the order-5 vertices are truncated.

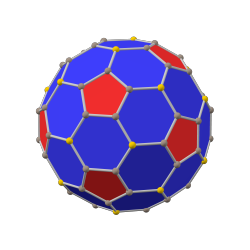 chamfered dodecahedron (canonical) |
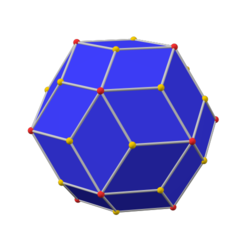 rhombic triacontahedron |
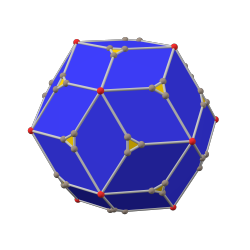 chamfered icosahedron (canonical) |
 pentakis icosidodecahedron |
 icosidodecahedron |
 triakis icosidodecahedron |
Chamfered icosahedron[edit]
| Chamfered icosahedron | |
|---|---|
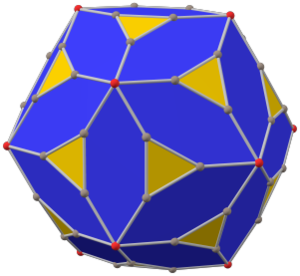 (with equal edge length) | |
| Conway notation | cI = t3daI |
| Faces | 20 triangles 30 hexagons |
| Edges | 120 (2 types) |
| Vertices | 72 (2 types) |
| Vertex configuration | (24) 3.6.6 (12) 6.6.6.6.6 |
| Symmetry | Ih, [5,3], (*532) |
| Dual polyhedron | Triakis icosidodecahedron |
| Properties | convex |
In geometry, the chamfered icosahedron is a convex polyhedron constructed from the rhombic triacontahedron by truncating the 20 order-3 vertices. The hexagonal faces can be made equilateral but not regular.
It can also be called a tritruncated rhombic triacontahedron, a truncation of the order-3 vertices of the rhombic triacontahedron.
Chamfered regular tilings[edit]
 Square tiling, Q {4,4} |
 Triangular tiling, Δ {3,6} |
 Hexagonal tiling, H {6,3} |
 Rhombille, daH dr{6,3} |

|

|

|

|
| cQ | cΔ | cH | cdaH |
Relation to Goldberg polyhedra[edit]
The chamfer operation applied in series creates progressively larger polyhedra with new hexagonal faces replacing edges from the previous one. The chamfer operator transforms GP(m,n) to GP(2m,2n).
A regular polyhedron, GP(1,0), create a Goldberg polyhedra sequence: GP(1,0), GP(2,0), GP(4,0), GP(8,0), GP(16,0)...
| GP(1,0) | GP(2,0) | GP(4,0) | GP(8,0) | GP(16,0)... | |
|---|---|---|---|---|---|
| GPIV {4+,3} |
 C |
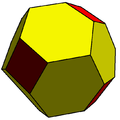 cC |
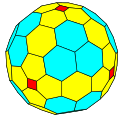 ccC |
 cccC |
|
| GPV {5+,3} |
 D |
 cD |
 ccD |
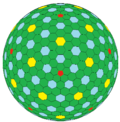 cccD |
 ccccD |
| GPVI {6+,3} |
 H |
 cH |
 ccH |
cccH |
ccccH |
The truncated octahedron or truncated icosahedron, GP(1,1) creates a Goldberg sequence: GP(1,1), GP(2,2), GP(4,4), GP(8,8)...
| GP(1,1) | GP(2,2) | GP(4,4)... | |
|---|---|---|---|
| GPIV {4+,3} |
 tO |
 ctO |
 cctO |
| GPV {5+,3} |
 tI |
 ctI |
 cctI |
| GPVI {6+,3} |
 tH |
 ctH |
cctH |
A truncated tetrakis hexahedron or pentakis dodecahedron, GP(3,0), creates a Goldberg sequence: GP(3,0), GP(6,0), GP(12,0)...
| GP(3,0) | GP(6,0) | GP(12,0)... | |
|---|---|---|---|
| GPIV {4+,3} |
 tkC |
 ctkC |
cctkC |
| GPV {5+,3} |
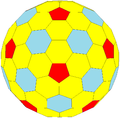 tkD |
 ctkD |
cctkD |
| GPVI {6+,3} |
 tkH |
 ctkH |
cctkH |
Chamfered polytopes and honeycombs[edit]
Like the expansion operation, chamfer can be applied to any dimension. For polygons, it triples the number of vertices. For polychora, new cells are created around the original edges. The cells are prisms, containing two copies of the original face, with pyramids augmented onto the prism sides.
See also[edit]
References[edit]
- ^ Spencer 1911, p. 575, or p. 597 on Wikisource, CRYSTALLOGRAPHY, 1. CUBIC SYSTEM, TETRAHEDRAL CLASS, FIGS. 30 & 31.
- ^ "C80 Isomers". Archived from the original on 2014-08-12. Retrieved 2014-08-09.
Sources[edit]
- Goldberg, Michael (1937). "A class of multi-symmetric polyhedra". Tohoku Mathematical Journal. 43: 104–108.
- Joseph D. Clinton, Clinton’s Equal Central Angle Conjecture [1]
- Hart, George (2012). "Goldberg Polyhedra". In Senechal, Marjorie (ed.). Shaping Space (2nd ed.). Springer. pp. 125–138. doi:10.1007/978-0-387-92714-5_9. ISBN 978-0-387-92713-8.
- Hart, George (June 18, 2013). "Mathematical Impressions: Goldberg Polyhedra". Simons Science News.
- Antoine Deza, Michel Deza, Viatcheslav Grishukhin, Fullerenes and coordination polyhedra versus half-cube embeddings, 1998 PDF [2] (p. 72 Fig. 26. Chamfered tetrahedron)
- Deza, A.; Deza, M.; Grishukhin, V. (1998), "Fullerenes and coordination polyhedra versus half-cube embeddings", Discrete Mathematics, 192 (1): 41–80, doi:10.1016/S0012-365X(98)00065-X.
- Spencer, Leonard James (1911). . In Chisholm, Hugh (ed.). Encyclopædia Britannica. Vol. 07 (11th ed.). Cambridge University Press. pp. 569–591.
External links[edit]
- Chamfered Tetrahedron
- Chamfered Solids
- Vertex- and edge-truncation of the Platonic and Archimedean solids leading to vertex-transitive polyhedra Livio Zefiro
- VRML polyhedral generator (Conway polyhedron notation)
- VRML model Chamfered cube
- 3.2.7. Systematic numbering for (C80-Ih) [5,6] fullerene
- Fullerene C80
- How to make a chamfered cube

















