Wrapped exponential distribution
|
Probability density function 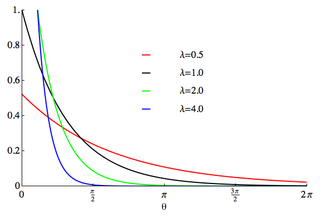 The support is chosen to be [0,2π] | |||
|
Cumulative distribution function  The support is chosen to be [0,2π] | |||
| Parameters | |||
|---|---|---|---|
| Support | |||
| CDF | |||
| Mean | (circular) | ||
| Variance | (circular) | ||
| Entropy | where (differential) | ||
| CF | |||
In probability theory and directional statistics, a wrapped exponential distribution is a wrapped probability distribution that results from the "wrapping" of the exponential distribution around the unit circle.
Definition[edit]
The probability density function of the wrapped exponential distribution is[1]
for where is the rate parameter of the unwrapped distribution. This is identical to the truncated distribution obtained by restricting observed values X from the exponential distribution with rate parameter λ to the range . Note that this distribution is not periodic.
Characteristic function[edit]
The characteristic function of the wrapped exponential is just the characteristic function of the exponential function evaluated at integer arguments:
which yields an alternate expression for the wrapped exponential PDF in terms of the circular variable z=e i (θ-m) valid for all real θ and m:
where is the Lerch transcendent function.
Circular moments[edit]
In terms of the circular variable the circular moments of the wrapped exponential distribution are the characteristic function of the exponential distribution evaluated at integer arguments:
where is some interval of length . The first moment is then the average value of z, also known as the mean resultant, or mean resultant vector:
The mean angle is
and the length of the mean resultant is
and the variance is then 1-R.
Characterisation[edit]
The wrapped exponential distribution is the maximum entropy probability distribution for distributions restricted to the range for a fixed value of the expectation .[1]
See also[edit]
References[edit]
- ^ a b Jammalamadaka, S. Rao; Kozubowski, Tomasz J. (2004). "New Families of Wrapped Distributions for Modeling Skew Circular Data" (PDF). Communications in Statistics - Theory and Methods. 33 (9): 2059–2074. doi:10.1081/STA-200026570. Retrieved 2011-06-13.













![{\displaystyle {\begin{aligned}f_{WE}(z;\lambda )&={\frac {1}{2\pi }}\sum _{n=-\infty }^{\infty }{\frac {z^{-n}}{1-in/\lambda }}\\[10pt]&={\begin{cases}{\frac {\lambda }{\pi }}\,{\textrm {Im}}(\Phi (z,1,-i\lambda ))-{\frac {1}{2\pi }}&{\text{if }}z\neq 1\\[12pt]{\frac {\lambda }{1-e^{-2\pi \lambda }}}&{\text{if }}z=1\end{cases}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/95e9059afd3d0d8d75dd6cb3cbe6e0acf8cb11b5)








