Mirror symmetry (string theory): Difference between revisions
Polytope24 (talk | contribs) added section on homological mirror symmetry |
Polytope24 (talk | contribs) →References: added reference |
||
| Line 122: | Line 122: | ||
* {{cite journal |last1=Lian |first1=Bong |last2=Liu |first2=Kefeng |last3=Yau |first3=Shing-Tung |year=2000 |title=Mirror principle, IV |journal=Surveys in Differential Geometry |pages=475--496}} |
* {{cite journal |last1=Lian |first1=Bong |last2=Liu |first2=Kefeng |last3=Yau |first3=Shing-Tung |year=2000 |title=Mirror principle, IV |journal=Surveys in Differential Geometry |pages=475--496}} |
||
* {{cite journal| author=Moore, Gregory | title=What is... a Brane?| journal=Notices of the AMS| year=2005 | url=http://www.ams.org/notices/200502/what-is.pdf |format=PDF| accessdate=June, 2013 |page=214| volume=52}} |
|||
* {{cite journal |last1=Sakai |first1=Norisuke |last2=Senda |first2=Ikuo |year=1986 |title=Vacuum energies of string compactified on torus |journal=Progress of Theoretical Physics |volume=75 |issue=3 |pages=692--705}} |
* {{cite journal |last1=Sakai |first1=Norisuke |last2=Senda |first2=Ikuo |year=1986 |title=Vacuum energies of string compactified on torus |journal=Progress of Theoretical Physics |volume=75 |issue=3 |pages=692--705}} |
||
Revision as of 18:49, 1 September 2013
| String theory |
|---|
 |
| Fundamental objects |
| Perturbative theory |
| Non-perturbative results |
| Phenomenology |
| Mathematics |
In mathematics and theoretical physics, mirror symmetry is a relationship between between geometric objects called Calabi-Yau manifolds. It can happen, for two such six-dimensional manifolds, that their shapes look very different geometrically, but nevertheless they are equivalent if they are employed as hidden dimensions of string theory.
Mirror symmetry was originally discovered by physicists. Mathematicians became interested in mirror symmetry after physicists showed that it could be used to solve problems in a branch of mathematics called enumerative algebraic geometry. Although the original approach to mirror symmetry was based on nonrigorous ideas from theoretical physics, mathematicians have gone on to rigorously prove some of the predictions of mirror symmetry.
Today mirror symmetry is a major research topic in pure mathematics, and mathematicians are working to develop a mathematical understanding of mirror symmetry based on physicists' intuition. Major approaches to mirror symmetry include homological mirror symmetry and the Strominger-Yau-Zaslow conjecture.
Overview
The idea of mirror symmetry
Mirror symmetry is a particular example of what physicists call a duality. In physics, the term duality refers to a situation where two seemingly different physical systems turn out to be equivalent in a nontrivial way. If two theories are related by a duality, it means that one theory can be transformed in some way so that it ends up looking just like the other theory. The two theories are then said to be dual to one another under the transformation. Put differently, the two theories are mathematically different descriptions of the same phenomena.[1]
Like many of the dualities studied in theoretical physics, mirror symmetry was discovered in the context of string theory. In string theory, particles are modeled not as zero-dimensional points but as one-dimensional extended objects called strings. One of the peculiar features of string theory is that it requires extra dimensions of spacetime for its mathematical consistency. In superstring theory, the version of the theory that incorporates worldsheet supersymmetry, there are six extra dimensions of spacetime in addition to the four that are familiar from everyday experience.[2]

In most realistic model of physics based on string theory, the extra dimensions are eliminated from the theory at low energies by a process known as compactification. This produces a theory in which spacetime has effectively a lower number of dimensions and the extra dimensions are "curled up" into complex shapes called Calabi-Yau manifolds. A standard analogy for this is to consider multidimensional object such as a garden hose.[3] If the hose is viewed from a sufficient distance, it appears to have only one dimension, its length. However, as one approaches the hose, one discovers that it contains a second dimension, its circumference. Thus, an ant crawling inside it would move in two dimensions. In certain models based on string theory, the Calabi-Yau manifolds play a role analogous to the circumference of the hose.
In the late 1980s, it was noticed that given such a compactification of string theory, it is not possible to uniquely reconstruct a corresponding Calabi-Yau manifold. Instead, one finds that there are two Calabi-Yau manifolds that give rise to the same physics. These manifolds are said to be "mirror" to one another. Although the full duality is still only a conjecture, there is a version of mirror symmetry in the context of topological string theory, a simplified version of string theory introduced by Edward Witten, and this version has been rigorously proven by mathematicians. In the context of topological string theory, mirror symmetry states that two theories, the A-model and B-model, are equivalent in a certain precise sense.
Regardless of whether these Calabi-Yau compactifications of string theory provide a correct description of nature, the existence of the mirror symmetry relationship between different Calabi-Yau manifolds has significant mathematical consequences.[4] The Calabi-Yau manifolds used in string theory are of interest in pure mathematics, and mirror symmetry allows mathematicians to solve many problems in enumerative algebraic geometry by solving equivalent problems for the mirror Calabi-Yau. Today mirror symmetry is an active area of research in mathematics, and mathematicians are still working to develop a mathematical understanding of mirror symmetry based on physicists' intuition.[5]
Complex geometry
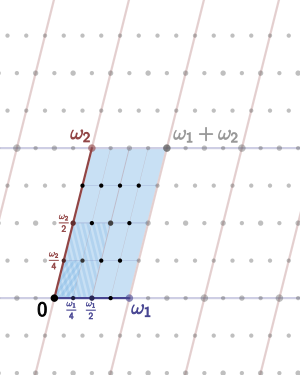
To understand the kind of geometry that appears on one side of the mirror duality, we will consider here a construction of a torus, a closed surface with a single hole like a donut, by identifying points of the complex plane. To construct this torus, we first choose a pair of complex numbers and such that the quotient is not real. This last condition ensures that these points are not collinear. Then the chosen points determine a parallelogram whose other vertices are 0 and . By identifying opposite sides of this parallelogram, we obtain the desired torus.
The tori obtained in this way are all equivalent in the sense that we can continuously deform one torus into another. On the other hand, they have an additional structure which allows us to distinguish them. Namely, the tori constructed in this way have a complex structure, meaning that a neighborhood of any point on such a torus looks just like a region in the complex plane.
If in the construction of a torus we had instead used a pair of complex numbers and related to the original pair by rescaling by a common factor (that is, and for some complex number ), then we would get an equivalent torus. It is therefore more convenient to parametrize the collection of tori by the ratio , which does not change when we rescale the . One can assume, without loss of generality, that this parameter has positive imaginary part so that takes values in the upper half plane. One can also show that the parameters , , and correspond to the same torus.
If two tori correspond to genuinely different values of , then they have inequivalent complex structures. One can think of the parameter as describing the "shape" of a torus constructed by identifying opposite sides of a parallelogram. As explained above, mirror symmetry relates two physical theories, the A- and B-models of topological string theory. In this duality, the topological B-model depends only on the complex structure of spacetime. Thus, if we consider the theory in which "spacetime" is a torus, the theory will depend continuously only on the parameter .[6]
Symplectic geometry
Another aspect of the geometry of a torus is the size of the torus. More precisely, we can talk view a torus as the surface obtained by identifying opposite sides of a unit square, and the area of the torus is specified by an area element on this square. By integrating this area element over the unit square, we obtain the area of the corresponding torus. These concepts can be generalized to higher dimensions, and the area element is generalized by the notion of a symplectic form. The study of spaces equipped with a symplectic form is called symplectic geometry.
In mirror symmetry, the A-model of topological string theory is a theory that depends only on the symplectic geometry of spacetime. Thus, if we consider the theory in which "spacetime" is a torus, then the A-model depends continuously only on the parameter .[7]
T-duality
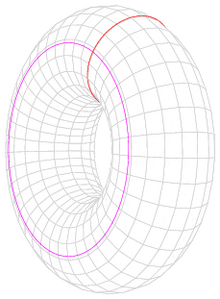
We have now seen how a torus can be obtained by identifying opposite sides of a parallelogram in the complex plane. A particularly simple example is where the complex numbers and lie on the real and imaginary axes, respectively. In this case, we can write and where and are real numbers. As discussed above, the complex structure on the torus obtained in this way is characterized by the number .
We have explained how the symplectic structure of the torus is determined by an area element. We can choose coordinates and on our parallelogram so that each side of the parallelogram spanned by the chosen complex numbers has length 1. Then the area element of our torus is which integrates to on the unit square. We define the symplectic parameter to be the product .
Note that the torus can also be considered as a cartesian product of two circles. This means that at each point of an equatorial circle on the torus (illustrated in pink), there is a longitudinal circle (illustrated in red).
Let us now imagine that the torus represents the "spacetime" for a physical theory. The fundamental objects of this theory will be strings propagating through the spacetime according to the rules of quantum mechanics. One of the basic dualities of string theory is T-duality, which states that a string propagating around a circle of radius is equivalent to a string propagating around a circle of radius in the sense that all observable quantities in one description are identified with quantities in the dual description.[8] For example, the momentum of a string in one description takes discrete values and is equal to the number of times the string winds around the circle in the dual description.[9] Applying T-duality to the longitudinal circle on the torus, we find that there is an equivalent description in which spacetime is represented by a different torus. The duality changes to , and thus it interchanges the complex and symplectic parameters:
- .
In general, mirror symmetry is an equivalence of two physical theories that translates problems in complex geometry into problems in symplectic geometry. The torus considered here is the only compact Calabi-Yau manifold of (real) dimension two and is therefore the simplest example of mirror symmetry.[10] In applications to string theory, one usually considers a six-dimensional Calabi-Yau manifold, where the six dimensions correspond to the six unobserved dimensions of spacetime.
Homological mirror symmetry
In string theory and related theories such as supergravity theories, a brane is a physical object that generalizes the notion of a point particle to higher dimensions.[11] For example, a point particle can be viewed as a brane of dimension zero, while a string can be viewed as a brane of dimension one. It is also possible to consider higher dimensional branes. The word brane comes from the word "membrane" which refers to a two-dimensional brane.
In string theory, a string may be open (forming a segment with two endpoints) or closed (forming a closed loop). D-branes are an important class of branes that arise when one considers open strings. As an open string propagates through spacetime, its endpoints are required to lie on a D-brane. The letter "D" in D-brane refers to the fact that we impose a certain mathematical condition on the system known as the Dirichlet boundary condition.
Mathematically, branes can be described using the notion of a category. This is a mathematical structure consisting of objects and for any pair of objects, a set of morphisms between them. In most examples, the objects are mathematical structures of some kind (for example, sets, vector spaces, or topological spaces) and the morphisms are given by functions between these structures. We can also consider categories where the objects are D-branes and the morphisms between two branes and are wavefunctions of open strings stretched between and .
In the B-model of topological string theory, the category of D-branes is constructed from the complex geometry of the Calabi-Yau manifold on which the strings propagate. In mathematical language, it is known as the derived category of coherent sheaves on the Calabi-Yau. On the other hand, the category of D-branes in the A-model is constructed from the symplectic geometry of the mirror Calabi-Yau. It is known in mathematics as the Fukaya category. The homological mirror symmetry conjecture of Maxim Kontsevich states that these two categories of branes are equivalent.
History
The idea of mirror symmetry can be traced back to the mid-1980s when it was noticed that a string propagating on a circle of radius is physically equivalent to a string propagating on a circle of radius in appropriate units.[12] This phenomenon is now known as T-duality and is understood to be closely related to mirror symmetry.
In a paper from 1985, Philip Candelas, Gary Horowitz, Andrew Strominger, and Edward Witten showed that by compactifying string theory on a Calabi-Yau manifold, one obtains a theory roughly similar to the standard model of particle physics.[13] Following this development, many physicists began studying Calabi-Yau compactifications, hoping to construct realistic models of particle physics based on string theory. It was noticed that given such a physical model, it is not possible to uniquely reconstruct a corresponding Calabi-Yau manifold. Instead, one finds that there are two Calabi-Yau manifolds that give rise to the same physics.[14]
In 1990, Edward Witten introduced topological string theory,[15] a simplified version of string theory, and physicists showed that there is a version of mirror symmetry for topological string theory.[16] This statement about topological string theory is usually taken as the definition of mirror symmetry in the mathematical literature.[17] In 1995, mathematician Maxim Kontsevich presented a new mathematical conjecture based on the physical idea of mirror symmetry in topological string theory. Known as homological mirror symmetry, this conjecture formalizes mirror symmetry as an equivalence of two mathematical structures: the derived category of coherent sheaves on a Calabi-Yau manifold and the Fukaya category of its mirror.[18]
In the years from 1996 to 2000, Alexander Givental, Bong Lian, Kefeng Liu, and Shing-Tung Yau showed how some of Kontsevich's ideas could be refined to effectively count rational curves.[19] These results form what is now considered to be the mathematical proof of mirror symmetry.
See also
Notes
- ^ Hori et al. 2003, p. xiv
- ^ Zwiebach 2009, p.8
- ^ This analogy is used for example in Greene 2000, p.186
- ^ Zaslow 2008, p.523
- ^ Hori et al. 2003, p. xix
- ^ Zaslow 2008, p.531
- ^ Zaslow 2008, p.531
- ^ Zaslow 2008, p.532
- ^ Zaslow 2008, p.532
- ^ Zaslow 2008, p.533
- ^ Moore 2005
- ^ This was first observed in Kikkawa and Yamasaki 1984 and Sakai and Senda 1986.
- ^ Candelas et al. 1985
- ^ This was observed in Dixon 1988 and Lerche, Vafa, and Warner 1989.
- ^ Witten 1990
- ^ Vafa 1992; Witten 1992
- ^ Hori et al. 2003, p. xviii
- ^ Kontsevich 1995
- ^ Givental 1996, 1998; Lian, Liu, Yau 1997, 1999, 2000
References
- Candelas, Philip; Horowitz, Gary; Strominger, Andrew; Witten, Edward (1985). "Vacuum configurations for superstrings". Nuclear Physics B. 258: 46--74.
- Dixon, Lance (1988). "Some world-sheet properties of superstring compactifications, on orbifolds and otherwise". ICTP Ser. Theoret. Phys. 4: 67--126.
- Givental, Alexander (1996). "Equivariant Gromov-Witten invariants". International Mathematics Research Notices. 1996 (13): 613--663.
- Givental, Alexander (1998). "A mirror theorem for toric complete intersections". Topological field theory, primitive forms and related topics: 141--175.
- Greene, Brian (2000). The Elegant Universe: Superstrings, Hidden Dimensions, and the Quest for the Ultimate Theory. Random House.
- Hori, Kentaro; Katz, Sheldon; Klemm, Albrecht; Pandharipande, Rahul; Thomas, Richard; Vafa, Cumrun; Vakil, Ravi; Zaslow, Eric, eds. (2003). Mirror Symmetry (PDF). American Mathematical Society. ISBN 0821829556.
- Kikkawa, Keiji; Yamasaki, Masami (1984). "Casimir effects in superstring theories". Physics Letters B. 149 (4): 357--360.
- Kontsevich, Maxim (1995). "Homological algebra of mirror symmetry". Proceedings of the International Congress of Mathematicians: 120--139.
- Lerche, Wolfgang; Vafa, Cumrun; Warner, Nicholas (1989). "Chiral rings in superconformal theories". Nuclear Physics B. 324 (2): 427--474.
- Lian, Bong; Liu, Kefeng; Yau, Shing-Tung (1997). "Mirror principle, I". Asian Journal of Math. 1: 729--763.
- Lian, Bong; Liu, Kefeng; Yau, Shing-Tung (1999). "Mirror principle, II". Asian Journal of Math. 3: 109--146.
- Lian, Bong; Liu, Kefeng; Yau, Shing-Tung (1999). "Mirror principle, III". Asian Journal of Math. 3: 771--800.
- Lian, Bong; Liu, Kefeng; Yau, Shing-Tung (2000). "Mirror principle, IV". Surveys in Differential Geometry: 475--496.
- Moore, Gregory (2005). "What is... a Brane?" (PDF). Notices of the AMS. 52: 214. Retrieved June, 2013.
{{cite journal}}: Check date values in:|accessdate=(help)
- Sakai, Norisuke; Senda, Ikuo (1986). "Vacuum energies of string compactified on torus". Progress of Theoretical Physics. 75 (3): 692--705.
- Vafa, Cumrun (1992). "Topological mirrors and quantum rings". Essays on mirror manifolds: 96--119.
- Witten, Edward (1990). "On the structure of the topological phase of two-dimensional gravity". Nuclear Physics B. 340: 281--332.
- Witten, Edward (1992). "Mirror manifolds and topological field theory". Essays on mirror manifolds: 121--160.
- Zaslow, Eric (2008), "Mirror Symmetry", in Gowers, Timothy (ed.), The Princeton Companion to Mathematics
- Zwiebach, Barton (2009). A First Course in String Theory. Cambridge University Press.

































