6: Difference between revisions
Undid revision 353195988 by 86.40.195.80 (talk) (vandalism) |
mNo edit summary |
||
| Line 408: | Line 408: | ||
*[[Six Degrees of Kevin Bacon]] is a game that can be played with the names of actors and actresses |
*[[Six Degrees of Kevin Bacon]] is a game that can be played with the names of actors and actresses |
||
*Before TV signals switched to all digital, the audio component of any [[Terrestrial television|broadcast]] channel 6 was located at 87.7 MHz. This meant that people could listen to TV stations such as [[WSYX]] using an FM radio (if it could tune to that frequency) when a television was not nearby |
*Before TV signals switched to all digital, the audio component of any [[Terrestrial television|broadcast]] channel 6 was located at 87.7 MHz. This meant that people could listen to TV stations such as [[WSYX]] using an FM radio (if it could tune to that frequency) when a television was not nearby |
||
*6 is one of the stitch punks in the 2009 film 9. |
|||
==In other fields== |
==In other fields== |
||
[[File:ICS Six.svg|right|thumb|66px|[[International maritime signal flags|International maritime signal flag]] for 6]] |
[[File:ICS Six.svg|right|thumb|66px|[[International maritime signal flags|International maritime signal flag]] for 6]] |
||
Revision as of 04:12, 3 April 2010
| 6 | |
|---|---|
| Template:Numbers (digits) | |
| Cardinal | 6 six |
| Ordinal | 6th sixth |
| Numeral system | senary |
| Factorization | |
| Divisors | 1, 2, 3, 6 |
| Roman numeral | VI |
| Roman numeral (Unicode) | Ⅵ, ⅵ |
| Arabic | ٦,6 |
| Arabic (Urdu) | ۶ |
| Amharic | ፮ |
| Bengali | ৬ |
| Chinese numeral | 六,陆 |
| Devanāgarī | ६ |
| Hebrew | ו (Vav) |
| Khmer | ៦ |
| Thai | ๖ |
| Tamil | ௬ |
| prefixes | hexa-/hex- (from Greek) |
| Binary | 110 |
| Octal | 6 |
| Duodecimal | 6 |
| Hexadecimal | 6 |
6 (six) is the natural number following 5 and preceding 7.
The SI prefix for 10006 is exa (E), and for its reciprocal atto (a).
In mathematics
Six is the second smallest composite number, its proper divisors being 1, 2 and 3. Since six equals the sum of these proper divisors, six is the smallest perfect number. As a perfect number, 6 is related to the Mersenne prime 3, since 21(22 - 1) = 6. (The next perfect number is 28.) It is the only even perfect number that is not the sum of successive odd cubes.[1] Being perfect six is the root of the 6-aliquot tree, and is itself the aliquot sum of only one number; the square number, 25. Unrelated to 6 being a perfect number, a Golomb ruler of length 6 is a "perfect ruler."[2] Six is a congruent number.
Six is the first discrete biprime (2.3) and the first member of the (2.q) discrete biprime family.
Six is the only number that is both the sum and the product of three consecutive positive numbers.[3]
Six is a unitary perfect number, a harmonic divisor number and a highly composite number. The next highly composite number is 12.
5 and 6 form a Ruth-Aaron pair under either definition.
The smallest non-abelian group is the symmetric group S3 which has 3! = 6 elements.
S6, with 720 elements, is the only finite symmetric group which has an outer automorphism. This automorphism allows us to construct a number of exceptional mathematical objects such as the S(5,6,12) Steiner system, the projective plane of order 4 and the Hoffman-Singleton graph. A closely related result is the following theorem: 6 is the only natural number n for which there is a construction of n isomorphic objects on an n-set A, invariant under all permutations of A, but not naturally in 1-1 correspondence with the elements of A. This can also be expressed category theoretically: consider the category whose objects are the n element sets and whose arrows are the bijections between the sets. This category has a non-trivial functor to itself only for n=6.
6 similar coins can be arranged around a central coin of the same radius so that each coin makes contact with the central one (and touches both its neighbors without a gap), but seven cannot be so arranged. This makes 6 the answer to the two-dimensional kissing number problem. The densest sphere packing of the plane is obtained by extending this pattern to the hexagonal lattice in which each circle touches just six others.
6 is the largest of the four all-Harshad numbers.
A six-sided polygon is a hexagon, one of the three polygons capable of tiling the plane. Figurate numbers representing hexagons (including six) are called hexagonal numbers. Six is also an octahedral number. It is a triangular number and so is its square (36).
There are six basic trigonometric functions.
There are six convex regular polytopes in four dimensions.
Six is the four-bit binary complement of number nine:
6 = 0110 9 = 1001
The six exponentials theorem guarantees (given the right conditions on the exponents) the transcendence of at least one of a set of exponentials.
In numeral systems
| Base | Numeral system | |
|---|---|---|
| 2 | binary | 110 |
| 3 | ternary | 20 |
| 4 | quaternary | 12 |
| 5 | quinary | 11 |
| 6 | senary | 10 |
| over 6 (decimal, hexadecimal) | 6 | |
In base 10, 6 is a 1-automorphic number.
List of basic calculations
| Multiplication | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | 25 | 50 | 100 | 1000 | |||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 6 | 12 | 18 | 24 | 30 | 36 | 42 | 48 | 54 | 60 | 66 | 72 | 78 | 84 | 90 | 96 | 102 | 108 | 114 | 120 | 126 | 132 | 138 | 144 | 150 | 300 | 600 | 6000 |
| Division | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 6 | 3 | 2 | 1.5 | 1.2 | 1 | 0.75 | 0.6 | 0.5 | 0.4 | |||||||
| 0.5 | 1 | 1.5 | 2 | 2.5 |
| Exponentiation | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 6 | 36 | 216 | 1296 | 7776 | 46656 | 279936 | 1679616 | 10077696 | 60466176 | 362797056 | 2176782336 | 13060694016 | ||
| 1 | 64 | 729 | 4096 | 15625 | 46656 | 117649 | 262144 | 531441 | 1000000 | 1771561 | 2985984 | 4826809 |
Evolution of the glyph
The evolution of our modern glyph for 6 appears rather simple when compared with that for the other numerals. Our modern 6 can be traced back to the Brahmins of India, who wrote it in one stroke like a cursive lowercase e rotated 90 degrees clockwise. Gradually, the upper part of the stroke (above the central squiggle) became more curved, while the lower part of the stroke (below the central squiggle) became straighter. The Ghubar Arabs dropped the part of the stroke below the squiggle. From there, the European evolution to our modern 6 was very straightforward, aside from a flirtation with a glyph that looked more like an uppercase G.[4]
On the seven-segment displays of calculators and watches, 6 is usually written with six segments. Some historical calculator models use just five segments for the 6, by omitting the top horizontal bar. This glyph variant has not caught on; for calculators that can display results in hexadecimal, a 6 that looks like a 'b' is not practical.
Just as in most modern typefaces, in typefaces with text figures the 6 character usually has an ascender, as, for example, in ![]() .
.
This numeral resembles an inverted 9. To disambiguate the two on objects and documents that can be inverted, the 6 has often been underlined, both in handwriting and on printed labels.
In science
Physics
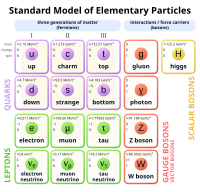
- In the Standard Model of particle physics, there are 6 types of quark and 6 types of lepton
- In statistical mechanics, the six-vertex model has 6 possible configurations of arrows at each vertex
Chemistry
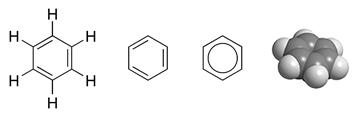
- A benzene molecule has a ring of 6 carbon atoms
- The atomic number of carbon is 6
- The sixfold symmetry of snowflakes arises from the hexagonal crystal structure of ordinary ice
- A hexamer is a oligomer made of six subunits.

Biology
Medicine
- The number of tastes in traditional Indian Medicine called Ayurveda. They are: sweet, sour, salty, bitter, pungent, and astringent. These tastes are used to suggest a diet based on the symptoms of the body
- Phase 6 is one of six pandemic influenza phases
Astronomy
- Messier object M6, a magnitude 4.5 open cluster in the constellation Scorpius, also known as the Butterfly Cluster
- The New General Catalogue object NGC 6, a spiral galaxy in the constellation Andromeda
- The Saros number of the solar eclipse series which began on -2691 March 16 and ended on -1393 May 3. The duration of Saros series 6 was 1298.1 years, and it contained 73 solar eclipses
- The Saros number of the lunar eclipse series which began on -2642 July 25 and ended on -1091 February 10. The duration of Saros series 6 was 1550.6 years, and it contained 87 lunar eclipses
- The Roman numeral VI stands for subdwarfs in the Yerkes spectral classification scheme.
- The Roman numeral VI (usually) stands for the sixth-discovered satellite of a planet or minor planet (e.g. Jupiter VI)
In religion

- The number of points on a Star of David
- The number of orders of the Mishnah
- The number of symbolic foods placed on the Passover Seder Plate
- The Jewish holiday of Shavuot starts on the sixth day of the Hebrew month of Sivan
- The Six articles of belief of Islam.
- In Islam, fasting six days of Shawwal together with the month of Ramadan is equivalent to fasting the whole year.
See also 666.
In music

- The number of strings on a standard guitar
- The number of basic holes or keys on most woodwind instruments (e.g., pennywhistle, clarinet, saxophone, bassoon). These holes or keys are usually not given numbers or letters in the fingering charts
- "Six geese a-laying" were given as a present on the sixth day in the popular Christmas carol, "The Twelve Days of Christmas"
- The concerti grossi Opus 3, organ concertos Opus 4 and Opus 7 (each) by Georg Frideric Handel
- The name of the second album by Mansun released in 1998. It takes its name in part from the main character in the television series The Prisoner, and from A. A. Milne's poetry book, Now We Are Six
- The French composers Georges Auric, Louis Durey, Arthur Honegger, Darius Milhaud, Francis Poulenc and Germaine Tailleferre were part of a group known as Les Six ("The Six" in English) in the 1920s.
- Bands with the number six in their name include 6 O'clock Saints, Eve 6, Slant 6, Sixpence None the Richer, Six In Six, Los Xey (sei is Basque for "six"), Vanity 6, You Me At Six, and Electric Six
- The number of semitones in a tritone
- The number that the sixth album by Dream Theater "Six Degrees Of Inner Turbulence" was based around. The album has six songs. The sixth song — that is, the complete second disc — explores the stories of six individuals suffering from various mental illnesses.
- #6, the pseudonym of American musician Shawn Crahan, when performing with Slipknot
In sports
- In American and Canadian Football, the number of points received for a touchdown
- Six-man football is a variant of American football, played by smaller schools with insufficient enrollment to field the traditional 11-man squad.
- In rugby union, the position of blindside flanker
- In rugby league, the position of 5/8
- In football/soccer, the number of AC Milan's legendary centre back and captain Franco Baresi. The shirt was retired in 1997.
- In football/soccer, the number of Arsenal's legendary centre back and captain Tony Adams. The shirt was retired in 2002 on his retirement but has recently been passed on to Philippe Senderos.
- In football/soccer, the number of Roy Keane for the Irish International Squad.
- In football (soccer), the number of substitutes combined by both teams, that are allowed in the game.
- In baseball, six represents the shortstop's position
- In Australian Rules football, six points are received for a goal
- In cricket (See cricket terminology):
- In ice hockey, the number of players per team, including the goaltender, that are on the ice at any one time, excluding penalty situations.
- Retired number of former baseball players Stan Musial, Tony Lazzeri, Al Kaline, Tony Oliva, Johnny Pesky and Steve Garvey
- In NASCAR, the number 6 car is driven by David Ragan.
- From 1988 to 2006, 6 was the number borne on the car of NASCAR driver Mark Martin.
- The Original Six teams in the National Hockey League are Toronto, Chicago, Montreal, New York, Boston, and Detroit. They are the oldest remaining teams in the league, though not necessarily the first six; they comprised the entire league from 1942 to 1967.
- The National Hockey League and National Basketball Association have six divisions.
- In American college football, there are six conferences that automatically qualify for Bowl Championship Series games.
- In some sports, 6 goals is known as a double-hat-trick, but is very hard to accomplish.
- The retired number of NBA legends Julius Erving and Bill Russell.
- In Volleyball, 6 players from each team on each side play each other.
In technology

- The resin identification code used in recycling to identify polystyrene.
- On most phones, the 6 key is associated with the letters M, N, and O, but on the BlackBerry it is the key for J and K. On BlackBerry 8700 series with full keyboard, it is the key for F.
- The "six meter band" in amateur radio includes the frequencies from 50 to 54 MHz
In television and film
- Number Six is the sixth of the twelve known Cylon models in the science fiction television series Battlestar Galactica
- Number Six is the main fictional character in the 1960s television series The Prisoner
- Six is the name of a character on Blossom
- Six (along with twenty-three and 801) is a recurring number on the revived series of Doctor Who
- The film Six Degrees of Separation deals with the sociological concept of the same name
- Six Degrees of Kevin Bacon is a game that can be played with the names of actors and actresses
- Before TV signals switched to all digital, the audio component of any broadcast channel 6 was located at 87.7 MHz. This meant that people could listen to TV stations such as WSYX using an FM radio (if it could tune to that frequency) when a television was not nearby
- 6 is one of the stitch punks in the 2009 film 9.
In other fields

- In the ancient Roman calendar, Sextilis was the sixth month. After the Julian reform, June became the sixth month and Sextilis was renamed August
- Sextidi was the sixth day of the decade in the French Revolutionary calendar
- The standard term in office for a United States senator is six years
- The name of the smallest group of Cub Scouts, traditionally consisting of six people and is led by a 'sixer'.
- Sixth Officer Hantin in The Pendragon Series
- The number of cans of soda or beer in a six-pack
- The number of fundamental flight instruments lumped together on a cockpit display, called the six-pack.
- The number of feet below ground level a coffin is traditionally buried; thus, the phrase "six feet under" means that a person (or thing, or concept) is dead

- In Astrology, Virgo is the 6th astrological sign of the Zodiac
- The number of sides on a cube, hence the highest number on a standard dice
- The highest number on one end of a standard domino
- The number of dots in a Braille cell.
- There are said to be no more than six degrees of separation between any two people on Earth. See also Six degrees (disambiguation).
- Extra-sensory perception is sometimes called the "sixth sense"
- Six Cardinal Directions: north, south, east, west, up, and down
- The Six Dynasties form part of Chinese history
- 6 is a lucky number in Chinese culture
- The Bionic Six are the heroes of an animated series
- The Birmingham Six were held in prison for 16 years
- 666 is considered the Number of the Beast
- "Six" is used as an informal slang term for the British Secret Intelligence Service, MI6
- Six Flags amusement parks and theme parks
Hexa is Greek for "six". Thus:
- A hexahedron is a polyhedron with six faces, with a cube being a special case
- An hexagon is a regular polygon with six sides
- L' Hexagone is a French nickname for the continental part of France
- Hexameter is a poetic form consisting of six feet per line
- "Hexadecimal" combines hexa- with the Latinate decimal to name a number base of 16
- A "hex nut" is a nut with six sides, and a hex bolt has a six-sided head.
The prefix "hexa-" also occurs in the systematic name of many chemical compounds, such as "hexamethyl"
Sex- is a Latin prefix meaning "six". Thus:
- A group of six musicians is called a sextet
- Six babies delivered in one birth are sextuplets
- People with sexdactyly have six fingers on each hand (see above photo)
- The measuring instrument called a sextant got its name because its shape forms one-sixth of a whole circle
- The ordinal adjective senary
See also
|
|
|
|
References
- ^ David Wells, The Penguin Dictionary of Curious and Interesting Numbers. London: Penguin Books (1987): 67
- ^ Bryan Bunch, The Kingdom of Infinite Number. New York: W. H. Freeman & Company (2000): 72
- ^ Peter Higgins, Number Story. London: Copernicus Books (2008): 12
- ^ Georges Ifrah, The Universal History of Numbers: From Prehistory to the Invention of the Computer transl. David Bellos et al. London: The Harvill Press (1998): 395, Fig. 24.66
- The Odd Number 6, JA Todd, Math. Proc. Camb. Phil. Soc. 41 (1945) 66—68
- A Property of the Number Six, Chapter 6, P Cameron, JH v. Lint, Designs, Graphs, Codes and their Links ISBN 0-521-42385-6
- Wells, D. The Penguin Dictionary of Curious and Interesting Numbers London: Penguin Group. (1987): 67 - 69