User:Tomruen/archive7

2013 talk archives

Uniform hyperbolic tilings

Which families do you plan to create articles for? You've done *642, and *832 is partially completed. Double sharp (talk) 13:55, 27 January 2013 (UTC)

Also, *542 is almost completed. (*732 was done some time ago.) Double sharp (talk) 13:58, 27 January 2013 (UTC)

I hadn't decided, or no plans specifically. I added *642 because with all evens it had 7 subsymmetries by alternation, and *842 also interesting for the same reason. It looks like *552 is next, but odd is more boring. Also *443 and *444 would be good, but not as articles I mean. I wish I could generate the dual images myself. I use Don Hatch's applet for the right-angle snubs, and have to hand-color which is a pain. Probably the summary example content at Wythoff symbol should be moved to Uniform tilings in hyperbolic plane. It would also be nice to systematically upload all the uniform tiling images with p,q,r to 9 each, with multiple images with different perspectives (and Klein!). I did this for fundamental domains, but not all perspectives! [1] Tom Ruen (talk) 22:18, 27 January 2013 (UTC)

Don Hatch's applet can generate the dual tilings for anything except the snub tilings. Double sharp (talk) 14:08, 8 February 2013 (UTC)

I added names for the non-right-angled fundamental domain tilings to the Wythoff symbol table. I've never seen anyone give them names, but (1) we'll need them to create articles with titles that don't look tremendously ugly (which I think we should do for at least *433 − we don't need *444, as all of its tilings are duplicated elsewhere) and (2) they are at least based on the names of the uniform polyhedra with non-right-angled fundamental domains. Double sharp (talk) 13:38, 28 January 2013 (UTC)

Thanks for finishing *552. Double sharp (talk) 13:22, 29 January 2013 (UTC)

I finished *832 for you. Double sharp (talk) 14:33, 28 January 2013 (UTC)

Also, if you want some interesting content about regular polyhedra, try *652. Double sharp (talk) 15:01, 28 January 2013 (UTC)

P.S. For regular polyhedron we need a picture of 5 | 5 3 ({5,6} coloured as (5a.5b)³). Could you make one? Double sharp (talk) 15:02, 28 January 2013 (UTC)

Thanks DS! Looks good all around. I'll make some more images sometime soon I hope. Tom Ruen (talk) 23:44, 28 January 2013 (UTC)

I added pictures for | 6 6 2, | 7 4 2 and | 8 4 2 (the snub images you hadn't done and weren't infinite). Double sharp (talk) 13:17, 29 January 2013 (UTC)

KaleidoTile problems

My copy of KaleidoTile somehow refuses to generate any tiling with p,q,r > 8. Since you've generated some tilings where at least one of them is 9 using KaleidoTile, what is going on? Double sharp (talk) 12:59, 2 February 2013 (UTC)

Don't know - I have a few versions, but I think I mainly use the release one. Or mine says 3.2.2 (2009)! I also have two special ones that show stereographic projections of spherical tilings, and one with Klein projections of hyperbolic. Jeff compiled the variations for me! Tom Ruen (talk) 20:40, 2 February 2013 (UTC)

Tetrahexagonal tiling

I understand what you intend by that fourth diagram , but that literally describes a honeycomb, not a tiling. Non-simplex domain tilings don't have CD diagrams. Double sharp (talk) 13:51, 2 February 2013 (UTC)

There is no Wythoff symbol beyond triangles but the Wythoff construction process and Coxeter diagram work in all cases. That is every reflective fundamental domain has a corresponding Coxeter diagram which defines the connectivity of mirrors and mirror angles as branches. Honeycombs can have a perpendicular infinity (for prismatic domains), like

. Euclidean tilings with *2222 orbifold symmetry have a square or rectangular domain (not simplex) and has CD

(seen as a degenerate tetrahedron). A quadrilateral domain in hyperbolic space can have connected infinities so *3222 is

and *p2q2 is a cyclic graph

. This group can only exist as a degenerate simplex in H2, never a 3D honeycomb. I was experimenting with the

form because Johnson said Vinberg used dotted/broken lines for infinite branches for non-intersecting mirrors in hyperbolic space. (I ordered a pricey used copy of this book to see what I could find [2].) The dotted branches in the middle make the graph look like *pqrs orbifold graph as a 4-sided graph, with implicit cross infinity terms suppressed. The main confusing point for me is there are two uses for infinity, like [(∞,∞,∞)] is an ideal triangle, vertices "meet" at infinity, while non-intersecting mirrors also have an infinity and occur in nonsimplex domains, so *∞222 is confusing as

with the middle ∞ representing an ideal vertex for the quad. So the dotted line infinities would help as

.

Johnson also has a representation (in bracket notation or Coxeter graph branch order) [πi/λ] (imaginary order) generating a pseudogon {πi/λ}, an infinite polygon in the hyperbolic plane). The group represents two ultrapallel mirrors separated by distance λ. So these imaginary branch orders could replace the ∞ branches with πi/λ₁ and πi/λ₂ in

, and would be more clearly useful for nonuniform domains and tilings.

I worked out an example Wythoff construction with *3222,

or

. I'll look for more sources with examples and symbolic usage for nonsimplex domains. There are 9 possible ring patterns for *pqrs in general, since logically at least one of opposite pairs always have to be ringed. 5 have all even terms and can be alternated. I'll add to an article or template sequence when I can confirm the terminology usage. Tom Ruen (talk) 20:24, 2 February 2013 (UTC)

- 6⁴ - also alternated - (3.4)⁴
- (3.4.4)²
- 4⁶ - also alternated - 6⁶
- repeat (3.4.4)²

- 6.6.4.4 - also alternated - (3.4.4)²
- 3.4.4.4.4
- repeat 6.6.4.4 - also alternated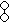
- repeat 3.4.4.4.4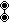
- 6.4.4.4 - also alternated 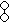- 3.4.4.4.4

Here's a first pass graphic for quadrilateral based Wythoff constructions of uniform tilings. I just labeled by vertex figures for now. For *2222, all the vertex figures are 4.4.4.4 of course! Tom Ruen (talk) 00:29, 3 February 2013 (UTC)

Thanks! We want more of these to clear up misconceptions. When can we expect them in the main CD diagram page? Double sharp (talk) 02:39, 3 February 2013 (UTC)

I did a bit of write up at Coxeter–Dynkin diagram#Higher polygonal groups with some quadrilateral group examples. I'm thinking perhaps the neglected article Wythoff construction should be revamped with the generalized 2D Kaliedoscopic process defined by Coxeter in spherical, Euclidean and Hyperbolic spaces. Tom Ruen (talk) 03:39, 3 February 2013 (UTC)

p.s. If you'd like to do some research, I see usage of Wythoff symbols beyond triangles (like Mandara - The World of Uniform Tessellations), and so there must be some system writeup somewhere, so at some point worth comparing, and perhaps adding definitions to Wythoff symbol. I mean Coxeter started the markups with the crazy uniform stars, and nonwythoffians, but anyway, not clear to me what all is being used. Tom Ruen (talk) 03:39, 3 February 2013 (UTC)

It could be derived from Miller's monster's four-number Wythoff symbol. Double sharp (talk) 14:20, 3 February 2013 (UTC)

Here are the tilings for *3222, symbolic fundamental domains on left and tilings on the right, made by hand with Tyler applet. Tom Ruen (talk) 19:39, 5 February 2013 (UTC)

Nice. Are you going to create articles? If so, I suggest 3.4.4.4.4 be called a rhombitriditetragonal tiling, if I haven't already used that name. It makes sense, being both 3.4.2.4.4.4 (treating "di-" as meaning a digon) and 3.4.4².4 (treating it as what Bowers calls dis, twice).

Could you give a table showing a general tetragonal fundamental domain (p q r s) and showing what happens to the vertex configuration when various nodes are ringed, like you did for a general trigonal fundamental domain (p q r)? What happens in general for n-gonal fundamental domains (p q r s t), (p q r s t u), etc.? Double sharp (talk) 14:29, 6 February 2013 (UTC)

P.S. 6.6.4.4 could be an elongated order-4 hexagonal tiling. Double sharp (talk) 14:30, 6 February 2013 (UTC)

P.P.S 3.4.4.3.4.4 could then be an elongated order-8(? I'm not sure) triangular tiling. Double sharp (talk) 14:33, 6 February

I don't plan on creating individual articles (only cross-referencing quad constructions on shared triangle symmetry articles), and so construction names are not important. I will keep looking for sources and what notations are in use. My current plan is to expand the Wythoff construction article with Spherical/Euclidean/Hyperbolic triangular cases included (maybe moving material from wythoff symbol) and include a section on higher polygonal domains, and using *2222 and *3222 as examples. Tom Ruen (talk) 20:34, 6 February 2013 (UTC)

Oh, in regards to "Could you give a table showing a general tetragonal fundamental domain (p q r s) and showing what happens to the vertex configuration when various nodes are ringed, like you did for a general trigonal fundamental domain (p q r)?" Yes, effectively, the vertex figures are in the graphic File:Wythoff_construction-pqrs.png, but this information can be put in a table too, with CDs. I just want to get that book first to confirm how parallel mirror branches are marked. Tom Ruen (talk) 20:34, 6 February 2013 (UTC)

Do you think we should have similar pictures of the *2222, *3222, *3232, *3322, *3332, and *3333 families just as we have pictures of *333, *433, *443, and *444? Double sharp (talk) 13:01, 23 February 2013 (UTC)

Probably? Not sure what you mean. Using Tyler and recoloring works if you have some patience. I can't do any more for now. Tom Ruen (talk) 21:53, 23 February 2013 (UTC)

Let's see:

(2 2 2 2)

4⁴
4⁴
4⁴
4⁴
4⁴
4⁴
4⁴
4⁴
4⁴

(3 2 2 2)

6⁴
6.6.4.4
(3.4.4)²
6.6.4.4
6.4.4.4
3.4.4.4.4
(3.4.4)²
3.4.4.4.4
4⁶

(3 2 3 2)

6⁴
6.4.3.6.4
(3.4)⁴
6.6.4.3.4
(6.4)²
6.6.4.3.4
(3.4)⁴
3.4.6.3.4
6⁶

(3 3 2 2)

(6.3.6)³
6⁴
(3.6.6)²
6.3.6.4.4
6.6.4.4
6.3.6.4.4
(3.4.4)³
(3.4.4)²
(3.4.4)³

(3 3 3 2)

(6.3.6)³
6.6.3.6.6
(3.6)⁴
6.3.6.4.3.4
6.6.6.4
6.6.3.6.6
(3.4)⁶
6.3.6.4.3.4
(3.6.6)³

(3 3 3 3)

(6.3)⁶
(6.6.3)²
(6.3)⁶
(6.6.3)²
6⁴
(6.6.3)²
(6.3)⁶
(6.6.3)²
(6.3)⁶

Double sharp (talk) 15:59, 27 February 2013 (UTC)

I am still wondering what happens for general polygonal fundamental domains. Double sharp (talk) 16:01, 27 February 2013 (UTC)

I'll trust you. So Tyler could make them all, with annoying hand-recoloring. This graphic File:Wythoff construction-pqrs.png shows the general rules using quadrilateral as an example. There are 2n+1 generator points for n-gon, by n corners, n edges, and interior. Orders are doubled, except on adjacent corners, and 2s are suppressed. Tom Ruen (talk) 19:26, 27 February 2013 (UTC)

BTW, what's a pseudogon? Is it like the Euclidean regular apeirogon, where it just diverges (instead of converges, as the horocyclic tiles of {∞,3} and other similar tilings do) to infinity? Would it then be like a straight hyperbolic line with vertices (marked at equal distance from each other for a regular pseudogon)? (We really should have an article on this distinction, rather than use the lousy sentence at the end of apeirogon. In fact, we should have three pages: apeirogon, horocycle, and pseudogon.) Double sharp (talk) 11:43, 10 March 2013 (UTC)

Uniform dual hyperbolic tilings

I'm making some images with Don Hatch's applet. *832 is up. Watch the templates. :-) Double sharp (talk) 14:46, 8 February 2013 (UTC)

And also *552. Double sharp (talk) 15:00, 8 February 2013 (UTC)

And also *662. Double sharp (talk) 15:02, 8 February 2013 (UTC)

And also *642. Double sharp (talk) 15:21, 8 February 2013 (UTC)

The images are just white tiles on a white background with thin black edges. They seem to look OK on my screen. They do look a bit boring compared with the other colourful images there (I think those are Rocchini's), but as you doubtlessly know, hand-colouring tilings is very time-consuming. ;-) Double sharp (talk) 15:23, 8 February 2013 (UTC)

Looks good! I colorized some of them using MSPaint. Tom Ruen (talk) 20:52, 8 February 2013 (UTC)

Thanks! (You just need to finish up *832 and *552 for everything to be coloured for now. :-)). Double sharp (talk) 02:54, 9 February 2013 (UTC)

I can't seem to generate any tilings where r > 2 on Don Hatch's applet, so for now their duals will have to remain imageless. :-( Double sharp (talk) 02:55, 9 February 2013 (UTC)

Yep, but Tyler can do more if your patient - any vertex figure, but every polygon must be placed by hand (and bug polygon area-fill wrong if curved edges are drawn). Tom Ruen (talk) 03:04, 9 February 2013 (UTC)

p.s. All the template(?) are linked at Uniform_tilings_in_hyperbolic_plane. Also I expanded the alternate forms for [6,6] so a few more images needed. See at Uniform_tilings_in_hyperbolic_plane#The_.5B6.2C6.5D.2C_.28.2A662.29_.286_6_2.29_group_family. Tom Ruen (talk) 04:03, 9 February 2013 (UTC)

Tyler won't work for the duals as it only suppotrs regular polygon tiles.

I added the *742, *842, *443, and *444 templates to Uniform tilings in hyperbolic plane. Double sharp (talk) 04:27, 9 February 2013 (UTC)

No duals, but Tyler can do any uniform snub (when r>2). Tom Ruen (talk) 04:29, 9 February 2013 (UTC)

I'm aware of that, but AFAIK the only dual snub we are missing (i.e. we have all the pictures in the family but not the snub) is | 5 4 3. I'll finish the dual images for *742 and *842 first. Double sharp (talk) 04:50, 9 February 2013 (UTC)

And I got the | 5 4 3 image from Richard Klitzing's website, so there's no problem now. Double sharp (talk) 12:11, 22 February 2013 (UTC)

*842 is done. Double sharp (talk) 04:50, 9 February 2013 (UTC)

As is *742. Now there's just a few alternates to fill in. Double sharp (talk) 04:59, 9 February 2013 (UTC)

Looks good, later just need a bit more effort in consistent colors for uniforms, and centers in general. Tom Ruen (talk) 17:34, 9 February 2013 (UTC)

Article Uniform tilings in hyperbolic plane

Should we include the noncompact tilings like *∞32, *∞42, *∞∞2, *∞33, *∞∞3, *∞∞∞? Double sharp (talk) 07:52, 9 February 2013 (UTC)

Yes, as sections in Uniform tilings in hyperbolic plane sounds good.

Rhombitetrahexagonal tiling

Are you sure that 3 2 2 2 | (omnitruncated *3222) = 4 | 6 2 (cantellated *642)? Don Hatch says they are not, despite sharing the same vertex configuration, 6.4.4.4. What is going on? Double sharp (talk) 07:57, 9 February 2013 (UTC)

BTW, I think you are right. Double sharp (talk) 08:51, 9 February 2013 (UTC)

I can check again. A more useful question also is what Hatch is doing ,worth looking in detail. I emailed him long ago over misuse of "runcination" instead of "cantellation" or "expanded" but he never replied. Tom Ruen (talk) 17:34, 9 February 2013 (UTC)

Personally I always preferred Bowers' "small rhombi-", "small prismato-" terminology, for the way it could easily be extended to higher dimensions and how it was so consistent with the Kepler names! Double sharp (talk) 14:43, 18 February 2013 (UTC)

Isn't that Wendy Krieger's use of runcination? Double sharp (talk) 15:13, 14 April 2014 (UTC)

Polybytes PolyImage Pro

Is that your program? ;-) Double sharp (talk) 08:14, 9 February 2013 (UTC)

Yes, this is the program I use for image manipulation. [3] Tom Ruen (talk) 17:34, 9 February 2013 (UTC)

*∞∞3 alternations

What are [(∞,∞,3⁺)] and [(∞,1⁺,∞,3)]? Double sharp (talk) 12:09, 22 February 2013 (UTC)

Orbifold notation: 3*∞∞ and *(∞3)². Tom Ruen (talk) 21:12, 22 February 2013 (UTC)

More recolouring requested

I uploaded the dual images for *∞32. Double sharp (talk) 11:48, 25 February 2013 (UTC)

Also *∞∞2. Double sharp (talk) 12:34, 25 February 2013 (UTC)

And finally *∞42. I can't create the other dual pictures. Double sharp (talk) 12:46, 25 February 2013 (UTC)

Also, can you make the lines thicker for the same way you did it for and others? Double sharp (talk) 12:50, 25 February 2013 (UTC)

Even more hyperbolic families

For completeness, I added *772 and *882. Can you help me expand the alternations for *882? Double sharp (talk) 17:19, 2 March 2013 (UTC)

Also, could you recolour

? Double sharp (talk) 17:24, 2 March 2013 (UTC)

Done. Tom Ruen (talk) 18:53, 2 March 2013 (UTC)

*444 also needs alternations expanded. Double sharp (talk) 07:43, 3 March 2013 (UTC)

Thanks. Double sharp (talk) 14:40, 4 March 2013 (UTC)

Fractional hyperbolic tilings

The only fractional families that give regular tilings (i.e. of the form p/q r/s 2) are of the form (p p/2 2). I'm only showing (7 7/2 2) below, although I guess a good case could be made to show (9 9/2 2) as well.

(p q r)	q \| p r (p.r)^q	p \| q r (q.r)^p	r \| p q (q.p)^r	q r \| p q.2p.r.2p	p r \| q p.2q.r.2q	p q \| r 2r.q.2r.p	p q r \| 2r.2q.2p	\| p q r 3.r.3.q.3.p
(7 7/2 2)	7^7/2	7/2⁷	File:Uniform tiling s7-t1.png 7.7/2.7.7/2	File:Uniform tiling s7-t12.png 7/2.14.14	7.7.7	File:Uniform tiling s7-t02.png 4.7.4.7/2	File:Uniform tiling s7-t012.png 4.14.4/3.14/13	File:Uniform tiling s7-snub.png 3.3.7/2.3.7

All my own names, but extrapolated from the uniform polyhedra. I can change some if some are degenerate. I have not tabulated the inverted forms (7 7/5 2), (7/6 7/2 2) and (7/6 7/5 2) because they are extremely confusing (just what is a 7/6.14/2.14/2? Doesn't it resolve to a heptagonal dihedron? But how does that make sense? Is it multi-covered?). Double sharp (talk) 11:38, 4 March 2013 (UTC)

The only online source I know for uniform star tilings is Mandara - The World of Uniform Tessellations. I don't have a great trust in Wythoff constructions for stars, with degeneracies, confuses me easily anyway. The Euclidean stars are given at Uniform_tiling#Expanded_lists_of_uniform_tilings, taken from Tilings and Patterns, sec 12.3 (hollow tilings). Tom Ruen (talk) 19:23, 4 March 2013 (UTC)

Colouring scheme used is Tyler's default. Double sharp (talk) 12:17, 5 March 2013 (UTC)

644 and 643 tilings

Why those two in particular? Double sharp (talk) 11:35, 5 March 2013 (UTC)

Sorry for not being orderly. (6 4 2) was interesting for being all evens, all different, so I extended from there. (8 6 4) might be fun too. Tom Ruen (talk) 19:23, 5 March 2013 (UTC)

Hmm, *544 as well...perhaps *663? Double sharp (talk) 12:20, 7 March 2013 (UTC)

Probably, although we don't want to add too many with blank images, and you must be getting tired too! :) Tom Ruen (talk) 19:31, 7 March 2013 (UTC)

For a complete sampling, we can just add *555, *663, *664, and *666. Those with both 6 and 5 are not too interesting. Double sharp (talk) 05:31, 8 March 2013 (UTC)

But (slightly) less ambitiously, we could stick with what you have. Most of the ones I mentioned earlier are subsymmetries of other symmetries already covered, so there will be a lot of duplicates which we don't really need.

Could you make the pictures for s5s3s3*a, s5s4s4*a, s6s3s3*a, s6s4s3*a, and s6s4s4*a? I know Tyler can do it, and I'm not very sure about getting the vertex configurations be of consistent handedness for chiral tilings! For the infinite snubs, you can use an approximation in Tyler like a 100-gon (which, at the default resolution, is close enough to look the same, but to be safe you might want to use a 1000- or 10000-gon). Double sharp (talk) 11:26, 10 March 2013 (UTC)

For what its worth, a computer search of uniform hyperbolic vertex figure User:Tomruen/HyperbolicVerfs, but no tests yet for shared constructions. Tom Ruen (talk) 05:03, 8 March 2013 (UTC))

Why 9? I can't make them... Double sharp (talk) 05:15, 8 March 2013 (UTC)

Okay, just 8 now, and new table with Anton's graphics at: User:Tomruen/HyperbolicVerfs2 I think I'm done for the night! :) Tom Ruen (talk) 05:33, 8 March 2013 (UTC)

p.s. This table above can be used to error-check vertex figures in like Template:Order 5-3-3 tiling table. I could rearrange the columns, but this was the easiest order for me to generate. Tom Ruen (talk) 05:59, 8 March 2013 (UTC)

I copied your BIG HONKING TABLE and pasted it at Uniform tilings in hyperbolic plane. Can you make an addendum to it consisting of tilings of the form (∞ q r), q,r = 2,3,4,5,6,7,8,∞? That would allow a summary to be made for the affine ones as well. (I really ought to expand the quadrilateral domain tilings at some point.) Double sharp (talk) 14:52, 8 March 2013 (UTC)

Holosnubs

[4] Double sharp (talk) 05:52, 8 March 2013 (UTC)

Yes, I know about them, but content to leave the star polytopes/honeycombs alone. Tom Ruen (talk) 05:57, 8 March 2013 (UTC)

s{5,4} tiling

The original picture had colours inconsistent with the rest of the snub images. Could you recolour it to use the red/yellow/cyan colour scheme of the others? (I uncoloured it first to make it simpler.) Double sharp (talk) 14:41, 8 March 2013 (UTC)

Thank you for lending your time to help us improve Wikipedia. If you are interested in editing more often than once in a while, we welcome you to log in and participate in our WikiCommunity. Double sharp (talk) 05:31, 17 March 2013 (UTC)

? —Tamfang (talk) 04:54, 10 July 2013 (UTC)

I meant {{thank you}} (

Thank you), instead of {{thanks}}. Double sharp (talk) 15:14, 14 April 2014 (UTC)

-kai- forms

We need to make sure there is no confusion between tetradecagonal tiling (4.10.4.10) and tetrakaidecagonal tiling (14.14.14). Double sharp (talk) 11:08, 10 March 2013 (UTC)

It appears that the "kai" serves as a marker to interpret the two numbers it is between as added to form one number – cf. tetratetradecagonal tiling (4.8.10.8) vs. tetratetrakaidecagonal tiling (4.14.4.14). Admittedly we'll never actually create articles on such high numbers, but we do need to guard against misinterpretation. Double sharp (talk) 11:11, 10 March 2013 (UTC)

Yes, that's John Horton Conway's advicemathforum.org, but unsure how to defend unless in the intro of every article of potential conflict, certainly they are all way too short now anyway. Tom Ruen (talk) 18:57, 10 March 2013 (UTC)

p.s. Truncated tetraapeirogonal tiling vs tetrapeirogonal? Tom Ruen (talk) 19:10, 10 March 2013 (UTC)

I prefer to keep the hiatus rather than remove it (as you do). It makes the elements clearer and is more in line with how I would imagine tetraapeirogonal pronounced in English (probably something like [tɛtɹə.əpeɪɹogənəl]). Ideally I would write tetra-apeirogonal tiling or tetraäpeirogonal tiling (with a diaeresis), but the former is inconsistent with every thing else and almost nobody uses the latter anymore. Double sharp (talk) 12:07, 11 March 2013 (UTC)

P.S. Chemical nomenclature keeps hiatuses, cf. triuranium octaoxide. Double sharp (talk) 11:07, 17 October 2013 (UTC)

Hyperbolic floret pentagonal tilings

I have the dual images for s{5,5}, s{8,3}, and s{∞,3} (better than nothing) from Don Hatch's page, but they are overlaid with the base snub tiling. Do you have any solution to this? Double sharp (talk) 05:26, 17 March 2013 (UTC)

(BTW, you could also suggest to Don Hatch that it might make more sense if there was a key control to display the snub dual. Also, does Ctrl+S (snub) work for non-omnitruncates? I think it should! If it doesn't, suggest Ctrl+A (alternate). Double sharp (talk) 05:30, 17 March 2013 (UTC))

I can't trivially filter out white, easier to remove RGB components, could be done, but hard given white is overlay, so blue is lost on small scales. Don Hatch wrote it a decade ago and has never replied to any emails. A union of regular and dual is dual tiling to t1. Tom Ruen (talk) 05:51, 17 March 2013 (UTC)

p.s. For cross-referencing, Anton made a collected list of all shared vertex figures and duals, put into a table by me at User:Tomruen/tempxx. I could shorten a lot if I filtered out all rows without duplicates! Tom Ruen (talk) 00:13, 18 March 2013 (UTC)

Okay, filtered shorter list User:Tomruen/tempxx2. Tom Ruen (talk) 00:23, 18 March 2013 (UTC)

[6,5] tilings

Since you've decided to start articles on this series (why, BTW?) I've uploaded dual pictures for the ones Tamfang didn't make. Please colour them. :-) In return for that I will create the articles you did not. Double sharp (talk) 07:43, 21 March 2013 (UTC)

I really hope you're not planning to create articles for all the tilings that can be generated for all hyperbolic simplex domains with 2 ≤ p,q,r ≤ 8, just to be able to use all of Tamfang's pictures... *shudders* Double sharp (talk) 08:18, 21 March 2013 (UTC)

No, [6,5] just smooths out the regular {p,q} table, although only 5 families left there, so maybe those, 2 ≤ p,q ≤ 8. I'm not even convinced individual articles are good as-is give minimal unique information about each, so perhaps better to combine them into one article with sections like the higher uniform polytopes. Tom Ruen (talk) 18:54, 21 March 2013 (UTC)

It also makes sense to include [6,5] because the regular ones of this family have some connection with some uniform polyhedra (see ditrigonal dodecadodecahedron). Only [7,5], [7,6], [8,5], [8,6], and [8,7] are left currently. (Are you going to do the infinite regular ones as well?) BTW, are you going to do articles for the pqr groups you made templates and sections in Uniform tilings in hyperbolic plane for? Double sharp (talk) 04:43, 22 March 2013 (UTC)

I'm just an inchworm for now, but looking how to best include more information about symmetry, currently collecting in omnitruncated form articles, and quick redirects as pqr symmetry. It would be nice (to reduce errors) to autogenerate all the Template:Order p-q-r tiling table templates even if most unused, but pictures would be empty outside of Anton's sets. Tom Ruen (talk) 05:29, 22 March 2013 (UTC)

I spammed out the table at Wythoff symbol.

For reference, my names for the general (p q r) tilings are (assuming p ≥ q ≥ r):

Anton -1: Di-q-gonal r-p-gonal tiling

Anton -3: r-q-p-gonal tiling (the logic is that the doubled one is stated last)

Anton -2: Di-r-gonal q-p-gonal tiling

Anton -6: r-p-q-gonal tiling

Anton -4: Di-p-gonal r-q-gonal tiling

Anton -5: q-p-r-gonal tiling

Anton -7: r-gonally truncated q-p-gonal tiling

Snub (if ever uploaded would be Anton -8, presumably?): Snub r-q-p gonal tiling (if two are the same, move them so that the two alike ones are not together).

Nah, I called them H2_snub_###a, where the projection's central simplex contains a vertex, and H2_snub_###b, where it does not. —Tamfang (talk) 22:23, 15 June 2013 (UTC)

Checking for duplicates is a must, especially when two or more of p, q and r are equal. Double sharp (talk) 06:01, 22 March 2013 (UTC)

I uploaded s{7,5}. The rest of the snubs are coming. Double sharp (talk) 14:59, 22 March 2013 (UTC)

s{8,6} is up. s{8,5} is next. Double sharp (talk) 12:00, 26 March 2013 (UTC)

Looks good, even if eventually most should just be moved to a subarticle. The names are problematic, but I'm not going to object. Tom Ruen (talk) 22:36, 22 March 2013 (UTC)

The names were based on the names of analogous uniform polyhedra, BTW. Double sharp (talk) 10:18, 23 March 2013 (UTC)

Those names always confusing too. There's two sets of names at [5], not sure the newer ones are any better. Tom Ruen (talk) 19:20, 26 March 2013 (UTC)

I found them confusing at first as well, but when I really thought about them they began to make sense.

I do not find Johnson's names an improvement (the TOCID notation is very opaque), but I do find Bowers' names to actually be improvements. Double sharp (talk) 12:45, 27 March 2013 (UTC)

Filling uniform polyhedra

You are doubtlessly aware of the problem with filling some of the non-orientable uniform polyhedra, that some surfaces with nothing below them ("membranes") get filled in. (Sidhei has one of the most blatant examples.) Since there don't seem to be pictures of the "neo filling" (the one Stella uses by default and calls "Auto Filling" – as normal for orientable polyhedra, but binary filling of faces of non-orientable polyhedra), I made them for those that look different (sidhei, gidhid, geihid, groh, giddy, gird, gisdid, gidrid, gidisdrid). Double sharp (talk) 15:04, 22 March 2013 (UTC)

The filenames just have a " 2" added. I admit to not seeing the difference for gisdid (can you?). Double sharp (talk) 15:06, 22 March 2013 (UTC)

This is outside my concerns. I am interested in hyperbolic nets (topological - without geometric intersections) of some of the uniform stars, especially ones with convex faces. Tom Ruen (talk) 22:38, 22 March 2013 (UTC)

By "hyperbolic net", you mean? Like the Euclidean net at octahemioctahedron? Double sharp (talk) 06:21, 23 March 2013 (UTC)

Then the nets are sections of:

Pip - 4.4.5 (S)
Pap - 3.3.3.5 (S)
Stip - 4.4.5/2 (S)
Stap - 3.3.3.5/2 (S)
Starp - 3.3.3.5/2 (S)
Tet - 3.3.3 (S)
Tut - 6.6.3 (S)
Oho - 6.3.6.3 (E)
Thah - 4.3.4.3 (S)
Oct - 3.3.3.3 (S)
Cube - 4.4.4 (S)
Co - 4.3.4.3 (S)
Toe - 6.6.4 (S)
Tic - 8.8.3 (S)
Sirco - 4.3.4.4 (S)
Girco - 4.6.8 (S)
Snic - 3.3.3.3.4 (S)
Socco - 8.3.8.4 (H)
Gocco - 8/3.3.8/3.4 (S)
Cho - 6.4.6.4 (H)
Cotco - 8/3.6.8 (S)
Querco - 4.3.4.4 (S)
Sroh - 8.4.8.4 (H)
Quith - 8/3.8/3.3 (S)
Quitco - 8/3.4.6 (S)
Groh - 4.8/3.4.8/3 (S)
Ike - 3.3.3.3.3 (S)
Doe - 5.5.5 (S)
Id - 3.5.3.5 (S)
Ti - 6.6.5 (S)
Tid - 10.10.3 (S)
Srid - 4.3.4.5 (S)
Grid - 4.6.10 (S)
Snid - 3.3.3.3.5 (S)
Sidtid - 5/2.3.5/2.3.5/2.3 (S)
Siid - 6.5/2.6.3 (S)
Seside - 3.5/2.3.3.3.3 (S)
Saddid - 10.3.10.5 (H)
Sissid - 5/2.5/2.5/2.5/2.5/2 (S)
Gad - 5.5.5.5.5 (H)
Did - 5/2.5.5/2.5 (S)
Tigid - 10.10.5/2 (S)
Raded - 4.5/2.4.5 (S)
Sird - 10.4.10.4 (H)
Siddid - 3.3.5/2.3.5 (S)
Ditdid - 5/2.5.5/2.5.5/2.5 (H)
Gidditdid - 10/3.3.10/3.5 (S)
Sidditdid - 10.5/2.10.3 (H)
Ided - 6.5/2.6.5 (H)
Idtid - 10/3.6.10 (S)
Sided - 3.5/2.3.3.3.5 (H)
Gidtid - 3.5.3.5.3.5 (H)
Giid - 6.3.6.5 (H)
Seihid - 10.3.10.3 (H)
Siddy - 10.6.10.6 (H)
Sidhid - 10.5.10.5 (H)
Gissid - 5/2.5/2.5/2 (S)
Gike - 3.3.3.3.3 (S)
Gid - 5/2.3.5/2.3 (S)
Tiggy - 6.6.5/2 (S)
Ri - 6.4.6.4 (H)
Gosid - 3.3.5/2.3.3 (S)
Quit Sissid - 10/3.10/3.5 (S)
Quitdid - 10/3.4.10 (S)
Isdid - 3.5/2.3.3.5 (S)
Gaddid - 10/3.5/2.10/3.3 (S)
Sidhei - 6.5/2.6.5/2 (S)
Giddy - 6.10/3.6.10/3 (H)
Gisdid - 3.5/2.3.5/2.3.3 (S)
Gidhei - 6.5.6.5 (H)
Quit Gissid - 10/3.10/3.3 (S)
Qrid - 4.5/2.4.3 (S)
Gaquatid - 10/3.4.6 (S)
Gisid - 3.5/2.3.3.3 (S)
Gidhid - 10/3.5/2.10/3.5/2 (S)
Geihid - 10/3.3.10/3.3 (S)
Sirsid - 3.3.3.3.3.5/2 (S)
Gird - 4.10/3.4.10/3 (S)
Girsid - 3.3.3.3.5/2 (S)
Gidrid - 4.5/2.4.3.4.5/2.4.3 (H)
Gidisdrid - 5/2.4.3.3.3.4.5/2.4.3.3.3.4 (H)

Double sharp (talk) 10:17, 23 March 2013 (UTC)

Yes, although simple pass would ignore ones with star polygon. Tyler could be used if we were patient and careful, but really its hard to immediately see how they wrap around without great care. Tom Ruen (talk) 20:41, 23 March 2013 (UTC)

Also related to hyperbolic tilings but not enumerated in any known sources are semiregular infinite skew polyhedron like File:Skew polyhedron 4446a.png with 4.4.4.6 vertex figure, colored there with same symmetry as hyperbolic *3222. Tom Ruen (talk) 20:45, 23 March 2013 (UTC)

List of uniform polyhedra by Schwarz triangle

I have been thinking about making pics like your KaleidoTile ones using Stella to cover this list properly (coloured faces according to symmetry positions p/q/r). What do you think? Double sharp (talk) 15:00, 30 March 2013 (UTC)

Colour scheme: p=red, q=yellow, r=blue, snub=cyan. Double sharp (talk) 15:08, 30 March 2013 (UTC)

How should I handle coincident/degenerate cases?

Eg. 2tet - two coincident tetrahedra. How should I colour such an object? I would prefer just using one colour, although symmetry colouring gets confusing... Double sharp (talk) 15:14, 30 March 2013 (UTC)

I am working on JUST the tetrahedrals for now. If things go well I will start on the octahedrals and dihedrals and the monstrous icosahedral section. (Now I am wondering whether doing this by hand is really a wise idea, but alas cannot think of another solution.) Double sharp (talk) 15:21, 30 March 2013 (UTC)

FYI I also created a 4D version User:Double sharp/List of uniform polychora by Goursat tetrahedron, but never got around to filling it up. I wonder if this might be useful...it would certainly be a lot of work to put on WP! I could also make a 5D version, but beyond that Klitzing stops giving starry cases, so List of uniform n-polytopes does the work. Double sharp (talk) 15:47, 30 March 2013 (UTC)

Euclidean version: User:Double sharp/List of uniform tilings by Schwarz triangle. (Only the polyhedron one ever got finished.) Double sharp (talk) 15:52, 30 March 2013 (UTC)

Ambitious! I'm content to leave the stars alone, too confusing. I remember reading somewhere Bowers and others gave up on the Goursat tetrahedron approach, although I think Johnson's offline dissertation has info on it. I saw today a new article on duals to convex uniform honeycombs called Catoptric tessellation by Conway's names. They're tough to visualize with irregular cells but makes me want to look again at Conway polyhedron notation extended to polychora! Tom Ruen (talk) 22:56, 30 March 2013 (UTC)

Also I seriously think we should split the Platonic and Archimedean polychora (polyhedral prisms included) back out into separate articles...they have enough info to justify that. They have about as much content as the individual Archimedean solid articles. Double sharp (talk) 14:49, 7 April 2013 (UTC)

I'm still not convinced. Its less work to edit and maintain a fewer number of articles. It would also be good to convert the stats into a db file. Tom Ruen (talk) 21:42, 7 April 2013 (UTC)

I will give it a try in userspace first to see how it would work before deciding whether or not to do this in article space. I would not do this for higher-dimensional polytopes as (1) I don't have as much info about them as in 4D and below and (2) the number of permutations of ringed and unringed nodes in the Wythoff symbols increases exponentially with the number of nodes, so it would quickly become unwieldy. Double sharp (talk) 15:37, 8 April 2013 (UTC)

Category:H2 symmetry group

Category:H2 symmetry group, which you created, has been nominated for possible deletion, merging, or renaming. If you would like to participate in the discussion, you are invited to add your comments at the category's entry on the Categories for discussion page. Thank you. Pichpich (talk) 01:41, 1 April 2013 (UTC)

Possible mistake on article Elongated square gyrobicupola

Hi,<br\> I'm contacting you because I read your 2009 comments on page Talk:Elongated_square_gyrobicupola.<br\> Can you please check that the image<br\> <br\> is actually an elongated square gyrobicupola? It doesn't look one to me: can you see that triangles and squares are not alternated as they are supposed to be?<br\> Cheers,<br\> Thewarriltonsiegedoc (talk) 02:41, 16 April 2013 (UTC)

Its NOT a Rhombicuboctahedron if that's what you're asking. It is hard to see. The triangle on the left is misaigned with the triangle on the lower right, as an elongated square gyrobicupola should be. Tom Ruen (talk) 02:55, 16 April 2013 (UTC)

Ok, no, my doubt was that it was not an elongated square gyrobicupola but a normal rhombicuboctahedron. Now I see the rotated square cupolae is on its side and the octagonal prism is presented vertically. I believe that the graphic representation should be rotated so that it would look like its solid version, with horizontal octagonal prism:

which is also consistent with its graphic explanation

You see that in this version the square cupolae is at the front of the figure under the horizontal octagonal prism? Do you happen to have the code used to generate the figure

? In this case I would recommend to slightly rotate it so that the readers don't have to twist their neck on one side to be able to compare the figure to the one used for its explanation.

(Actually I think that it would be more intuitive if the rotated square cupolae was on the top of the octagonal prism, not under (?!))

Thank you for your attention.

Thewarriltonsiegedoc (talk) 21:03, 16 April 2013 (UTC)

Rhombille tiling in English heraldry.

On 13 April 2013 you commented on a proposed edit to the page on Rhombille tiling that "This pattern is more clearly derived from a trihexagonal tiling than rhombic tiling."

Thank you for the advice - you are right, I overlooked that aspect. Even so I find the economy of the rhombille pattern marginally more satisfying, in that rhombille tiling only requires identical tiles in 2 colours to generate the pattern, whereas trihexagonal tiling needs 2 distinct tiles (but I'm an Architect first (and mathematician second) and thus (probably) notably peculiar in such matters).

I have revised my edit and my web pages http://www.pleiade.org/col_geal/aux_armes-symbolism.html#geometry_arms and http://www.pleiade.org/col_geal/aux_armes-symbolism-notes.html#fig_2 to reflect your comment.

My regards, Adeianike (talk) 14:43, 17 April 2013 (UTC)

Sure, looks like both work with added edges. There were some colorings of the rhombille tiling removed last year, historical page here, 3 of them had hexagonal stars, with one like yours. [6]. Tom Ruen (talk) 19:11, 17 April 2013 (UTC)

E-clipse

You have seniority. I have to take your move of the section I just worked on as, in part at least, an endorsement of the whole of it (completely unsourced until I got there; still largely unsourced, including really "original [something]" from me on ME). But it feels a little like having the "fun" stuff up top. Except that the bulk of the article (now below "me") did seem sort of like "boilerplate" (not specific to the one eclipse). Anyway, mostly, just a "Hello and nice to make your acquaintance". Cheers. Swliv (talk) 03:53, 25 April 2013 (UTC)

I don't have a stake on contents or verifiability, just wanted information about this specific eclipse above the "related eclispes" section, whether it needs to be challenged or sourced I'll leave that to you. Tom Ruen (talk) 05:04, 25 April 2013 (UTC)

I'm glad I worked it out to the degree of addressing you as I did on the 25th. Now I've reworked, trimmed and better integrated my contribution. Thanks for helping me sort it through. Swliv (talk) 18:07, 26 April 2013 (UTC)

Cube vs. regular hexahedron

Hi Tom,

Just noticed your move war. I'm happy to support you in any way I can as a dumb user, drop me a line if there's anything I can do. — Cheers, Steelpillow (Talk) 21:04, 1 May 2013 (UTC)

And the other guy has earned an indef block. Ah well, it's the thought that counts. — Cheers, Steelpillow (Talk) 21:08, 1 May 2013 (UTC)

Hi Guy. Thanks! I'm just like you! Fortunately an admin(?)-person handled it. It is usual to have a persistent troll! Tom Ruen (talk) 21:31, 1 May 2013 (UTC)

New article of possible interest to you

See First stellation of rhombic dodecahedron. —David Eppstein (talk) 17:04, 11 May 2013 (UTC)

Upcoming Wikipedia meetups

In the area? You are invited to the upcoming Minnesota meetups.

To kick-off monthly meetups in the Twin Cities, two events will be held in Special Collections at Minneapolis Central Library this summer. These are mostly planned as opportunities for Wikipedians to discuss editing, but all are welcome!

Special Collections contains many valuable historical resources, including the Minneapolis Collection, consisting of files on hundreds of topics related to Minneapolis from neighborhoods to politicians (it's best to call or email in advance to request materials). Free wifi and several public computers are available.

Place: Minneapolis Central Library, 300 Nicollet Mall, Minneapolis
Special Collections (4th floor)
Dates: Saturday, June 1
Saturday, July 6
Time: 12:30pm–2:30pm+

For more info and to sign up (not required), see the meetup talk page.

This invitation was sent to users who were interested in past events. If you don't want to receive future invitations, you can remove your name from the invite list. —innotata 14:18, 24 May 2013 (UTC)

Re: New flat torus picture

Great animated picture! Think you could do something similar for the non-intersecting Klein Bagel (w = sin v)? Klein_bagel#Parameterization Cloudswrest (talk) 03:42, 11 June 2013 (UTC)

Sorry, not my picture, just relinked from duocylinder. Tom Ruen (talk) 03:53, 11 June 2013 (UTC)

Great American Wiknic

In the area? You're invited to the Great American Wiknic.

Place: north of Minnehaha Falls in Minnehaha Park, Minneapolis
Date: Saturday, June 22, 2012
Time: 12–4 pm

Accessible from the Minnehaha Park METRO station, bus, walk, bike, or car
If driving, free parking available on 46th Ave. S, and pay parking in the park
Food and drink options nearby, or bring your own... maybe even to share!

For more, and to sign up (encouraged, not required) go to the meetup talk page.

This invitation was sent to users who were interested in past events. If you don't want to receive future invitations, you can remove your name from the invite list. —innotata 02:58, 13 June 2013 (UTC)

And now for something completely loony

Do you know enough to generate the analemma formed by Earth's apparent motion in the Moon's sky? Is it even approximately a closed curve? —Tamfang (talk) 22:25, 15 June 2013 (UTC)

I can simulate that motion, but it is chaotic and I don't have a rotating reference-frame history that can easily be drawn. I can make an animation.... Tom Ruen (talk) 22:37, 15 June 2013 (UTC)

Here's a 365 day animation from today (shows phases, but no surface detail), viewed from crater on right horizon of the moon, facing western horizon. So you'd really see this motion if you lived there, most noticable if the earth is near the horizon. Tom Ruen (talk) 22:44, 15 June 2013 (UTC)

Thanks, that's enough to tell me that it's roughly elliptical rather than a figure-eight, satisfying my curiosity. I suppose the colored dots are other planets? —Tamfang (talk) 05:51, 16 June 2013 (UTC)

Yep, planets and sun - I turn off background star, etc, but don't have an UI option to selectively turn off planets. A figure-8 comes from a large inclination of the axis to orbit, but the moon is doubly weird (or simple) being tidally-locked facing. This is more like a spirograph that perhaps repeats after its 18 year eclipse cycle? Tom Ruen (talk) 21:08, 16 June 2013 (UTC)

E6-An alternative description

Hello Tomruen! I don't think the version as it appears is consistent. Let me explain why and please tell me if I am wrong. I take the labels of the diagram as it appears(6 being on the bottom) as my starting point. Then (reading off the matrix as it is given) we see that the fifth and sixth simple roots are:

a_{5}=(0,0,0,1,1,0)

a_{6}=(-{\frac {1}{2}},-{\frac {1}{2}},-{\frac {1}{2}},-{\frac {1}{2}},-{\frac {1}{2}},{\frac {\sqrt {3}}{2}})

their dot product is $a_{5}\cdot a_{6}=-1$ and according to the Dynkin rules the dot product of two simple roots is -1 if and only if the two nodes are connected by a single line in the Dynkin diagram. This is clearly inconsistent since in the given diagram nodes 5 and 6 are not connected. I believe the version of the matrix I gave in my edit is the one that is consistent with the diagram, but please let me know if and why you disagree.

Best, Bilingsley (talk) 09:36, 4 July 2013 (UTC)

Tom, I think Bilingsley is correct. If you take the dot product of each of 6 rows of the simple roots against all 6 rows, they create the Cartan matrix (under his change). I am checking the other Lie group pages to verify they are correct.

It looks like for the E8 page simple roots, we get the given Cartan matrix when the rows are reordered to {8,7,5,4,3,2,1,6}.

For the E7 page simple roots, we get the given Cartan matrix when the rows are reordered to {7,6,4,3,2,1,5}.

Jgmoxness (talk) 21:31, 4 July 2013 (UTC)

Thanks for checking, Jgmoxness. It seems like the diagram indices should be renumbered to match the matrices rather than the reverse. Maybe one of your SVG diagrams would be better (than the templates) with all the nodes properly labeled? Tom Ruen (talk) 22:09, 4 July 2013 (UTC)

BTW - the D4 on the Root System page is properly ordered.

IMO, renumbering the Dynkin vs. reordering the simple roots is equivalent from the perspective of the rule Bilingsley pointed out. My preference is to reorder the simple roots (as he suggested for E6 and I suggested for E7 and E8) because the Dynkin order with the last node off the line is consistent with directly creating the Cartan matrix with a very simple algorithm as you build the Dynkin (which I use in my Mathematica Demonstrations here [[7]]). I understand the aesthetic desire to keep the order of simple roots, and I see the reordering pattern seems to favor shifting the Wiki page Dynkin diagrams to have "off-the-line nodes" to be on the right hand side (vs. left) and that node number coming before the last two on the line.

Additionally, I have found an interesting set of simple roots (a permutation of the indices within each row) that links the split real even (SRE) E8 vertices described on that page directly with their positive (and negative) algebra roots. This is described in equations 7 and 8 of [[8]], specifically:

algebra root[i]=Inverse[Transpose[simple root[i]]].SRE[i]

and E7 is (of course) a natural subset of that SRE E8 as well.

So I guess I would like to undo the undo of the Bilingsley E6 change and reorder E7 and E8 simple roots, but first let me check to see if there is an easy way to get both of our aesthetic desires accomplished with new Dynkin diagrams as you suggest.

Jgmoxness (talk) 03:48, 5 July 2013 (UTC)

Sure, do whatever is best, for all E6/7/8. Tom Ruen (talk) 04:49, 5 July 2013 (UTC)

I uploaded new images to match the existing order File:DynkinD4_labeled.png, File:DynkinE6_labeled.png, File:DynkinE7_labeled.png, File:DynkinE8_labeled.png, more readable than the SVG, but I see at [9] there's two systems apparently (Dynkin ordering, Bourbaki ordering) which this order is none! Dynkin is more sensible, but it counts from the end of the 2-branch, which would but the funny coordinate one first. Tom Ruen (talk) 01:30, 6 July 2013 (UTC)

I don't know why I bother - you say do what is best and you replace everything I worked to do.

Some piece of work.

Jgmoxness (talk) 04:09, 6 July 2013 (UTC)

I'm sorry, I was frustrated by the templates not being able to label branching graphs, hoped your SVG are easy to make, but they are hard to read when they're small. Maybe you can make a "big" flag sometime, so they're more readable when small? I don't like my hand-made PNG, but only 3 cases. I'm still concerned the order isn't standard, but don't have time to look at printed sources for ideas. Tom Ruen (talk) 04:15, 6 July 2013 (UTC)

I too apologize for getting upset at your replacements (which are admittedly more readable - and that is the WP way). I made mine more readable as SVG and put the order back to canonical Dynkin order (I don't like Bourbaki either).

The Dynkin diagram generates the Cartan matrix directly. Reordering the nodes can create a valid Cartan matrix, but not the canonical ones shown. The specific E7/E8 Cartan matrices shown on the WP pages are created with the left side node linked to node 3 (as shown on the website above). That Cartan matrix should then be generated in the row by row dot product for any given simple roots matrix as pointed out above. The Cartan matrix is then used to generate the poset algebra roots used in the Hasse diagrams shown on those pages. This means there is a 1 to 1 correspondence between Cartan, Dynkin, simple roots and the Hasse diagrams!

The problem for the WP pages is that in order to get the row by row dot product to produce the given Cartan matrix, the simple root order or the Dynkin diagram nodes need to be re-ordered. Unfortunately, the Dynkin node reordering (as you and I have done so far) doesn't directly generate the given Cartan matrix and it isn't the canonical order as you pointed out.

They are all "valid algebras" just not the SAME algebras. So the real problem is in errant notions about what are "standard" Cartan, Dynkin, simple roots and poset roots.

The final cross check of consistency across Dynkin, Cartan, and Hasse posets is that the transpose of the simple roots matrix dot product with poset algebra root vectors generates the group vectors.

BTW - the given simple roots on the E8 page is missing a minus sign on the last element of the row of +/- 1/2s in order to generate the split real even E8 group as shown on that page. It is correct on the Root system page for the alternate Dynkin node order.

The consistent set of E6/E7/E8 Dynkin, Cartan, and simple roots (consistent with poset Hasse diagrams shown) are as follows:

So I recommend we fix the simple root diagram orders (and the E8 minus sign), and make sure the E7 & E8 Cartan matrix is consistent with the shown right side vertical nodes connecting node 4 and 5 respectively.

I've already uploaded the new SVG Dynkin diagrams with larger nodes and fonts and can modify the pages if you agree

Jgmoxness (talk) 01:24, 7 July 2013 (UTC)

Looks good. Please use your new ones. Since you have the different systems, I agree its good to include multiple systems, with Cartan and Simple root matrices together. Tom Ruen (talk) 01:43, 7 July 2013 (UTC)

I noticed you put in a D4 Dynkin with alternate node ordering. Interestingly, due to the triality symmetry of the D4 Dynkin (and the corresponding simple roots and the resulting Cartan matrices), those two node orderings are equivalent. As I said above- D4 was correct as-is, so you could undo that change if desired.

Jgmoxness (talk) 15:29, 7 July 2013 (UTC)

Yes, I made it just to explicitly label all 4 nodes, File:DynkinD4_labeled.png, unlike the template, but maybe 3/4 should be swapped positionally for consistency. Tom Ruen (talk) 15:42, 7 July 2013 (UTC)

License tagging for File:Circle limits III with overlay.png

Thanks for uploading File:Circle limits III with overlay.png. You don't seem to have indicated the license status of the image. Wikipedia uses a set of image copyright tags to indicate this information.

To add a tag to the image, select the appropriate tag from this list, click on this link, then click "Edit this page" and add the tag to the image's description. If there doesn't seem to be a suitable tag, the image is probably not appropriate for use on Wikipedia. For help in choosing the correct tag, or for any other questions, leave a message on Wikipedia:Media copyright questions. Thank you for your cooperation. --ImageTaggingBot (talk) 03:05, 6 July 2013 (UTC)

File:Circle limits III with overlay.png

You need to give this file a fair-use rationale for its use in tritetragonal tiling. (It may seem silly, but the rules are that every fair-use image needs a separate justification for every article that it appears in.) —David Eppstein (talk) 06:54, 6 July 2013 (UTC)

Thanks David. I added a section, and understand why its needed. Maybe with some time I'll try another overlay effort on a higher resolution version (like [10]), and lower again for wikipedia upload. Tom Ruen (talk) 01:43, 7 July 2013 (UTC)

Coxeter groups of Dynkin diagrams

I just noticed your extensive work on the Coxeter groups of Dynkin diagrams. It might be interesting to show the corresponding Cartan matrix for each diagram. I have a tool that can very quickly generate an SVG of the combined Dynkin and Cartan (as you see above). We could hyperlink to the File: image in commons (vs. attempt to squeeze the pic into these pages). Let me know if you're interested (as I don't want to step on your work and/or waste time and effort). Jgmoxness (talk) 14:12, 9 July 2013 (UTC)

Not sure. I assume you're talking about Coxeter-Dynkin diagram article? I'm not sure of the value of the Cartan matrices as graphic representations, vs something you might copy&paste for calculations. Lastly, a node order would have to be assumed, which there is no standard that can be assumed to be useful? Tom Ruen (talk) 19:56, 9 July 2013 (UTC)

More important I think might be to generate the full list of 238 hyperbolic Dynkin diagrams in ranks 3-10, enumerated at [11] since my templates are too limited. Tom Ruen (talk) 20:01, 9 July 2013 (UTC)

Yes, the Coxeter–Dynkin_diagram article.

Not sure what you mean by "copy/paste for calculations" (do you really think anyone is copy/pasting the WP text into a symbolic or algorithmic solver?). I could paste the text version of the Cartan array in the description of the SVG for that purpose.

There is a 1 to 1 correspondence between Dynkin and Cartan. Node order (whether canonical or NOT) is still VALID algebra and IMO, if no one has declared what is canonical it shouldn't prevent the publishing of the Cartan (e.g. declare nodes on top are numbered before nodes on the bottom).

Point is - the Dynkin creates the Cartan and they inform each other.

My offer is to generate Cartans for your Dynkins. If you want to generate 238 Dynkins - fine. I will follow with up to 238 Cartan+Dynkin SVGs. (if you can't generate the Dynkin due to limitations - I might be able to fill it in with my tools generated similarly sized SVG - and still URL point to the larger SVG+Cartan like the others would be).

Yes or No ??

Jgmoxness (talk)

I'm sorry. I guess the simple answer is no. I don't see how a Cartan matrix is helpful for readers. Tom Ruen (talk) 03:34, 10 July 2013 (UTC)

It's no more or less helpful than the Dynkin. Since they are equivalent - why do you have a bias to the Dynkin?

Jgmoxness (talk) 03:38, 10 July 2013 (UTC)

Diagrams are good for human readability. Matrices are good for computation. Knowing how to convert is important, but readers will not be doing calculations. Plus for undirected diagrams entries are not integers, so a Coxeter matrix is more convenient matrix compliment, and still hard to read in all but the most trivial cases. Tom Ruen (talk) 03:49, 10 July 2013 (UTC)

I understand your opinion, but I may do it anyway.

Jgmoxness (talk) 12:42, 10 July 2013 (UTC)

Do you actually show square roots in your matrices for undirected graphs? Tom Ruen (talk) 20:35, 10 July 2013 (UTC)

The Coxeter graphs shouldn't have arrows, like at File:DynkinC2Affine.svg. Also Cartan wrong for G~2, should be -3,-1, not -4,-1! File:DynkinG2Affine.svg Tom Ruen (talk) 06:02, 16 July 2013 (UTC)

ok, I will fix them. Thanks for checking.

Jgmoxness (talk) 13:19, 16 July 2013 (UTC)

ok, I think I've addressed your concerns (although you may not like the directed and undirected in one graphic, but I think it helps understand the differences).

Please see my attempt to generate the first 5 of the 238 enumerated hyperbolic Dynkin diagrams

Jgmoxness (talk) 21:37, 28 July 2013 (UTC)

Looks ok on first impression. One thought, drop the colors, and all filled or unfilled nodes, unless that have some clear meaning and purpose, or plan to upload varied format sets. If I used a branch color for instance, I'd use to imply branch order, but in general they are B&W. If they look "professional" I imagine some mathematicians might borrow them from wikipedia for papers, etc. Also consider how they look at different sizes, maybe these are good. Tom Ruen (talk) 23:33, 28 July 2013 (UTC)

Minnesota Wikipedia Meetup on August 3

In the area? You are invited to the upcoming Minnesota monthly meetup on August 3.

Place: Lavvu Coffee House
813 4th St SE, Minneapolis 55414
Date: Saturday, August 3
Time: 1:00pm-3:00pm+

For more info and to sign up (not required), see the meetup talk page.

This invitation was sent to users who were interested in past events. If you don't want to receive future invitations, you can remove your name from the invite list.

—innotata 23:59, 24 July 2013 (UTC)

Alternated form of orthoplex

In some pages on Gosset polytopes you use a Coxeter diagram trick (which alas I don't fully understand) to work out their facets, and when you get a facet corresponding to a D_n Coxeter diagram you say it corresponds to an orthoplex 'in its alternated form'. What does that mean, exactly? Is it an orthoplex with its facets colored with two colors in an alternating way? John Baez (talk) 16:08, 25 July 2013 (UTC)

Hi John! I've seen and admired your wonderful blogs! Yes, they're the same polytope in two different kaleidoscopic constructions (set of mirrors), so the D_n family has a fundamental domain twice as large as the BC_n fundamental domain. That is you can get the D_n domain by removing one end-mirror of BC_n. And yes, that makes alternating "colored" facets in certain symmetry positions. For example, easier to see, BC_3 (octahedral) and D_3=A_3 (tetrahedral), the cuboctahedron, among others has two colorings:

Tetrahedral:

as diagram

or

, and Octahedral:

as diagram

.

So the "trick" can be shown in Coxeter diagram correspondence tables like this. I labeled the nodes by index that correspond in each row, and the black nodes are "added" or "removed" depending on the direction. So these are just Coxeter groups and lastly you can identify related polytopes by giving the SAME indexed nodes the SAME ring-state, AND the black nodes have to remain unringed (inactive mirror). The rules of the correspondences can be deduced if you look at the polytopes. Otherwise you have to look at the fundamental simplex, remove a mirror, and see what comes out. Does that help?

ALSO note that removing a mirror can also create a nonsimplex domain, like removing the diagonal of a 45-45-90 triangle ([4,4] group,

) creates a square domain, which is rank 4 diagram with parallel mirrors

. Tom Ruen (talk) 19:48, 25 July 2013 (UTC)

I'll have to think about this more - that was a very dense burst of information you emitted there! But thanks. And thanks immensely for all the pictures you've been creating. I've been using many in blog articles and with your blessing will also use some in a forthcoming page featuring mathematical images at the American Mathematical Society website, to be called something like "Visual Insight" John Baez (talk) 23:22, 26 July 2013 (UTC)

Cool. I'll look forward to seeing your paper! Tom Ruen (talk) 00:14, 27 July 2013 (UTC)

Tetraoctagonal tiling

It seems like the picture is not correct. It shows order-8 square tiling (4.4.4.4.4.4.4.4) instead of tetraoctagonal tiling (4.8.4.8)? Stannic (talk) 15:45, 14 August 2013 (UTC)

Got it. Fixed at Template:Uniform_hyperbolic_tiles_db Tom Ruen (talk) 19:38, 14 August 2013 (UTC)

Categorizing images of tilings at Commons

Hello, please take a look at this and this page. — Stannic (talk) 18:23, 18 August 2013 (UTC)

Proposal to move unsorted images of hyperbolic tilings

Hello, please take a look at this discussion again. You've created many images that are in categories discussed there, so your opinion is welcome. — Stannic (talk) 17:12, 29 August 2013 (UTC)

Mixed Latin Greek etymology

Good call, this. I had been wondering how we could drop these unencyclopedic commands without losing the information. It looks like you found the proper solution. Let's indeed leave the decision to avoid using mixed Latin/Greek etymology to our readers. Cheers - DVdm (talk) 09:59, 1 September 2013 (UTC)

Nomination for deletion of Template:Infobox Solar eclipse2

Template:Infobox Solar eclipse2 has been nominated for deletion. You are invited to comment on the discussion at the template's entry on the Templates for discussion page. eh bien mon prince (talk) 21:04, 1 October 2013 (UTC)

Digonal antiprism image

		The Wikignome Award
		Thanks for the digonal antiprism and digonal trapezohedron images, and related editing. 75.150.168.6 (talk) 21:47, 6 October 2013 (UTC)

You've got mail

Hello. Please check your email; you've got mail!
It may take a few minutes from the time the email is sent for it to show up in your inbox. You can remove this notice at any time by removing the {{You've got mail}} or {{ygm}} template.

— Cheers, Steelpillow (Talk) 09:23, 14 October 2013 (UTC)

Cantellated 7-cubes (Tricantitruncated 7-cube) & Cantellated 7-orthoplexes (Cantitruncated 7-orthoplex)

Hi, I've been going through Articles with missing files trying to remove junk but more importantly fix important missing files. I noticed that Cantellated 7-cubes#Tricantitruncated 7-cube is missing File:7-cube t234.svg and Cantellated 7-orthoplexes#Cantitruncated 7-orthoplex is missing File:7-cube t456.svg from the sets of images that it looks like you originally created. I have no idea how to create these and so thought I'd just point them out in case you hadn't noticed and could remedy. Cheers KylieTastic (talk) 17:35, 26 October 2013 (UTC)

Hi Kylie, thanks for the note. Unfortunately no easy fix I think. Some of the SVG files were too big to render with the wiki engine, and were eventually deleted, a full list at List_of_B7_polytopes. So the template tables would have to be modified to skip files that don't exist, and I know there's a way, but not within my knowledge. Tom Ruen (talk) 18:50, 26 October 2013 (UTC)

Hi - I've updated the template to allow for a parameter to exclude the B7/A6 image - I couldn't find out if you can detect if an image exists to do it dynamically. KylieTastic (talk) 12:04, 4 November 2013 (UTC)

Thanks for the fix at Template:B7 Coxeter plane graphs. When I see the problem elsewhere, I'll repeat it. Tom Ruen (talk) 23:14, 4 November 2013 (UTC)

Quilting

I made a quilt using one of your models as the inspiration, check it out here: http://www.flickr.com/photos/amandamaher/8929265191/in/photostream/ — Preceding unsigned comment added by 66.56.15.76 (talk) 16:48, 3 November 2013 (UTC)

Nice! Tom Ruen (talk) 06:25, 4 November 2013 (UTC)

For reference, this model is W₄₈. Double sharp (talk) 14:54, 24 March 2014 (UTC)

Grand antiprism

Hey Tom,

I was thinking, in your picture for the grand antiprism, if you put an icosahedral face on the visible ends of the pentagonal antiprism stacks, the picture would be suitable as net for the 600-cell. Cloudswrest (talk) 00:03, 7 November 2013 (UTC)

I'm sorry, which picture? Grand antiprism Tom Ruen (talk) 00:12, 7 November 2013 (UTC)

This one https://en.wikipedia.org/wiki/File:Grand_antiprism_net.png Cloudswrest (talk) 00:32, 7 November 2013 (UTC)

I'm muddled or lazy on seeing the derivation of the grand antiprism from the 600-cell. It looks like there's no 600-cell net uploaded, but I just checked Stella4D, and it is a big spherical mess of tetrahedra, a completely different connectivity. It would be great if we could show the relations. 00:40, 7 November 2013 (UTC)

The only difference is the interior of those blue pentagonal antiprism cylinders. In the grand antiprism they are "hollow". In the 600-cell they are filled with tetrahedra. Cloudswrest (talk) 00:44, 7 November 2013 (UTC)

Okay, I sort of see? The pentagonal antiprisms are "voids" from deleted tets. So There's 300 tets missing, and 20 A5's, so each A5 should contain 15 tets (5 up, 5 down, and 5 edge-wise?). ... Yes, that works! So a 600-cell could be similar by making a 15-tet cluster as almost a pentagonal prism with fold angle gaps. I'm just not sure how much that would distort the 3D net. Tom Ruen (talk) 01:02, 7 November 2013 (UTC)

Yeah you're right if you want to be 3-D isometric. I was thinking of cheating and just putting a 5-triangle end caps on the visible ends of the cylinders. Oops. Cloudswrest (talk) 01:08, 7 November 2013 (UTC)

It's still a good idea, to show the grand antiprism's relation to lower symmetry "coloring" of the 600-cell, [5,3,3], order 14400, vs [[10,2⁺,10]], order 400, subgroup index 36. Tom Ruen (talk) 03:49, 7 November 2013 (UTC)

Tested a picture, hand-edited net picture, on the right! Tom Ruen (talk) 03:58, 7 November 2013 (UTC)

Incidentally my real interest is to have a [5,3,3] subgroup tree, like the icosahedral tree to the right, but so far no one has attempted that to my knowledge! Tom Ruen (talk) 04:02, 7 November 2013 (UTC)

Update of Icosahedral_subgroup_tree.png

Hi, I just remade https://en.wikipedia.org/wiki/File:Icosahedral_subgroup_tree.png in graphviz: https://commons.wikimedia.org/wiki/File:Icosahedral_subgroup_tree_(graphviz_version).png I hope I removed that "ms-paint"-flair. The source files can be found on github: https://github.com/jaseg/icosahedral-symmetry

Regards, jaseg — Preceding unsigned comment added by Jaseg (talk • contribs) 13:34, 18 November 2013 (UTC)

Hi jaseg. That's very nice as far as a graph/tree goes, but can it be readable on smaller sizes, i.e. having larger nodes, and shorter edges? Tom Ruen (talk) 14:14, 18 November 2013 (UTC)

Coxeter notation

Hello, Tom.

I am trying to read the article about Coxeter notation, but I still don't understand the strict algorithm explaining this nomenclature for point groups with two +'s inside brackets. I understand the idea of adding one + (inside or outside the brackets), but two +'s is too complicated for me. For example, why [2+,4+] - is 4 and [2+,2+] - is 1 Do you know, by chance, explanation? Thank you,

Boris Bor75 (talk) 08:24, 23 November 2013 (UTC)

Yes, it is tricky. Coxeter writes this in the [2+,2n+] cases for Schönflies notation, S_2n, Hermann–Mauguin notation 2n or n, but Johnson generalized it to any two even order branches, [2p⁺,2q⁺] as well. Computationally [2⁺+,2n⁺], generated from [2,2n] by reflection set {1,2,3} corresponding to the 3 mirror nodes of the coxeter diagram , become glide reflections or improper rotations set {123,231,312}. In the [2⁺,2⁺] case, you have 3 orthogonal mirrors, and adding the product of all 3 operations in one step only leads to the opposite point across the origin, thus a point reflection. You can see [2⁺] as a point reflection in 2D, [2⁺,2⁺] a point reflection in 3D, [2⁺,2⁺,2⁺] a point reflection in 4D, etc... Tom Ruen (talk) 00:35, 24 November 2013 (UTC)

Generators and relations for descrete groups, Coxeter and Moser, 4th edition, 1980 is the book you want. It out of print, hard to buy, but at my local university library, so I expect you can find it too. [12] Tom Ruen (talk) 00:53, 24 November 2013 (UTC)

Yes, I took this book from library. Thank you. This is interesting, that [2⁺,2n⁺] cases for Schönflies notation, S_2n. I just couldn't understand, what is the formal definition for [n+,m+]. I checked today the book of Conway and Smith "On Quaternions and Octonions" and I like what he wrote "When just one of the numbers p,q,...r,s is even say that between Rk and Rk+1, there exists two further subgroups, namely... [p⁺,q,...,r,s] and [p,q,...,r,s⁺] consisting of words that mention respectively R1...Rk, and Rk+1..Rn evenly, whose intersection is the index 4 subgroup [⁺p,q,...,r,s⁺] of words that mention both of these sets evenly." I just wondering, that maybe in this case formal definition cold be: [m⁺,n⁺] is intersection of [m⁺,n] and [m,n⁺]. As I see now, you also wrote something like this on the article "The full gyro-p-gonal group, [2⁺,2p], abstractly Dih_2p, of order 4p. The gyro-p-gonal group, [2⁺,2p⁺], abstractly Z_2p, of order 2p is a subgroup of both [2⁺,2p] and [2,2p⁺]." Bor75 (talk) 04:50, 24 November 2013 (UTC)

Yes. And sadly, you can't look at Conway's "version" of Coxeter's notation. That is Conway agrees with odd-branched +'s but not even, like Coxeter's [3⁺,4,3⁺] is close (as [⁺3,4,3⁺] for Conway), with a separating even branch, but not for [4⁺,4⁺]. Conway's [⁺4,4⁺] would mean Coxeter's [1⁺,4,4,1⁺]. Conway might write [⁺4,3⁺], while Coxeter's real notation is [1⁺,4,3⁺], Conway's [⁺4,3,3⁺] is Coxeter's [1⁺,4,(3,3)⁺]. Tom Ruen (talk) 05:29, 24 November 2013 (UTC)

p.s. My effort to sort out Conway's Quaternion 4D groups from Coxeter's is here User:Tomruen/polychoric_groups. I got 100% translation on the main groups, but failed to sort out the duoprismatic notations clearly. Tom Ruen (talk) 05:51, 24 November 2013 (UTC)

Actually I was just looking with this in the hyperbolic plane [4,6], looking at the same tiling with edge colorings to show the subsymmetries. [6,4], [6+,4], [6,4+], and [6+,4+]. Tom Ruen (talk)

Thank you, Tom, for your explanations. Frankly speaking, it is too complicated for me. I just wanted to understand the Coxeter notation when it is applied to point groups in usual Euclidean space. I don't know much about tiling and still don't understand much about coxeter diagram. I am more experienced in symmetry (in Euclidean space), crystallography, and group theory. But these coxeter notations are mentioned in tables of articles "Crystal system", "Point group", "Crystallographic point group". When I follow link from these articles to "Coxeter notation" article, I don't understand much.

First, because this article gives very general definition, applied to such objects, about which I don't know much (while I just wanted to understand their meaning for usual point groups).
Second, in the first sentence, the article explains the notations in terms of Coxeter-Dynkin diagram. I don't know about them. I go to Coxeter–Dynkin diagram article and to understand it I need to read one more article - Coxeter group. Coxeter group article give strange and very formal definition and sends me back to Coxeter–Dynkin diagram and to Reflection group article and some other articles. Reflection group article gives some general information which is not helpful.

Too much work for person, who just wanted to understand the meaning of Coxeter notation for point groups :) Bor75 (talk) 23:42, 24 November 2013 (UTC)

I understand. The Coxeter group article does need to be better integrated with the Coxeter diagram article. Coxeter's work starts with reflection groups, and sees everything else as subgroups from that. Orbifold notation is more natural approach for identification, but less useful for showing subgroup relations. So there's advantages and disadvantages to each system, which is why I try to support them all in articles about symmetry. Tom Ruen (talk) 02:52, 25 November 2013 (UTC)

Thanks

Hey, thanks for helping out with the AdS/CFT article. I didn't know what you where trying to emphasize in one of your edits, and I only undid it because the word "and" was missing. Polytope24 (talk) 04:59, 9 December 2013 (UTC)

Sure, I saw after that I was sloppy in my edit. I looked only after surprised to see the image I uploaded in that physics article, and reused in File:AdS3 (new).png. I wasn't sure if I was being too exact, replacing quadrilateral with square. I assume there's nothing specific about that tessellation, but I'm not sure, many more at uniform tilings in hyperbolic plane. Tom Ruen (talk) 05:27, 9 December 2013 (UTC)

Missing near-uniform polyhedra

We have no article on the pseudoquasirhombicuboctahedron? It's a near-uniform polyhedron (in the sense that it has regular faces and all vertices have the same vertex figure, but it is not vertex-transitive) that you get when you twist half of the nonconvex great rhombicuboctahedron by 45 degrees; see Grünbaum's "An enduring error". Seems like the sort of article that you'd enjoy creating and creating one of your excellent illustrations for. —David Eppstein (talk) 07:06, 17 December 2013 (UTC)

This? Double sharp (talk) 08:02, 17 December 2013 (UTC)

I see Double Sharp started it here User:Double_sharp/Pseudo-great_rhombicuboctahedron Tom Ruen (talk) 14:02, 17 December 2013 (UTC)

This graphic is so misleading I nominated it for deletion

See here: File:Lunar_eclipse_optics.jpg Your input to any discussion of the nomination would be appreciated and totally optional of course. — TimL • talk 10:13, 22 December 2013 (UTC)